Weights
Different combinations of the weights available allow you to make different totals. Which totals can you make?
Problem
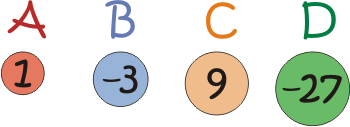
Imagine you have two of each of the 'weights' above.
Different combinations of the weights available allow you to make different totals.
For example:
- $B + C = 6$
- $B + 2C = 15$
- $A + 2B + C = 4$
- $2A + B + 2C + D = -10$
The largest total you can make is $20$ (check you agree).
The smallest total you can make is $-60$ (again, check you agree).
Can you make all the numbers in between?
Is there always a unique way of producing a total, or can different combinations produce the same total?
Extension:
If you are allowed just three different weights this time ($E$, $F$ and $G$), and at least one must be a negative weight, and you are now allowed to have up to three of each, you could choose:
$F = -4$
$G = 5$
You could make $7$ and $-10$:
$2E + 3F =$ $-10$
Choose your three weights and test out which totals you can make.
Which set of three weights ($E$, $F$ and $G$) allows you to make the largest range of totals with no gaps in between?
With thanks to Don Steward, whose ideas formed the basis of this problem.
Getting Started
The largest total you can make is $20$ ($2A + 2C$).
The smallest total you can make is $-60$ ($2B + 2D$).
There are some totals that are easier to produce than others (perhaps those that require just one or two weights), so it may be a good idea to start with those and then build on them with a few extra weights.
Student Solutions
We received a complete set of solutions, showing that all the numbers between -60 and 20 could be done, from Fred, Chester and Tom of Hotwells Primary School, by Jordan from Isleworth and Syon School and from Josh B, Matthew H, Seb W, Ben S, Max G and Jamie W, all in 8P1 at Hove Park School. Here is the solution from the Hove Park students (addition signs have been omitted so it is easier to see the pattern that forms).
When you are faced with such a problem it is helpful to work systematically. An anonymous solver used a stem leaf diagram.
It is possible to make every number between -60 and 20. I found this out by doing a series of different
combinations of the weights. I started by adding weight A to weight B then 2A to B then 2B to A and then 2A to 2B. After I found out every possible combination, I was left with 81 answers. I wrote all
the numbers out on a number line and used a stem leaf diagram to put them in size order.
The stem leaf diagram made it easy to see if there were any missing numbers, which there were not.
Here is the stem leaf diagram that I drew out.
2|0
1|0,1,2,3,4,5,6,7,8,9
0|0,1,2,3,4,5,6,7,8,9,-1,-2,-3,-4,-5,-6,-7,-8,-9
-1|0,1,2,3,4,5,6,7,8,9
-2|0,1,2,3,4,5,6,7,8,9
-3|0,1,2,3,4,5,6,7,8,9
-4|0,1,2,3,4,5,6,7,8,9
-5|0,1,2,3,4,5,6,7,8,9
-6|0
For the first part of the problem, we also received this great solution from Rhea in Loughborough High School. She found a convincing argument for why all the numbers between -60 and 20 can be made, which didn't require her to write down a combination for every single number between -60 and 20.
The Maths Challenge Group at Colyton Grammar School explained how they approached the problem:
Teachers' Resources
Why do this problem?
This problem offers students an opportunity to add positive and negative numbers while challenging them to work systematically.
Possible approach
This printable worksheet may be useful: Weights.
Introduce the scenario as it appears in the problem. Negative weights could be seen as strong helium balloons lifting up the pan of the weighing scales.
Students could suggest a few examples for the class to work through and clarify the procedure. Ask about the largest total and the smallest total, giving time for students to explain to their partners why $20$ and $^-60$ are correct.
Set the class working on the main task:
Can you make all the numbers in between? Can you show us how?
Is there always a unique way of producing a total, or can different combinations produce the same total?
There are different strategies for tackling this problem so if you observed that students approached the task in different ways, ask (carefully selected) students to describe how they did it. This could lead to a discussion of the merits of the different approaches.
Key questions
Can you make all the numbers in between? Can you show us how?
Is there always a unique way of producing a total, or can different combinations produce the same total?
Possible support
Organise students into small teams with a big central sheet of paper. Teams can write the numbers from $20$ to $^-60$, and then students can fill in any sum that they find. Ask students to pass their suggested sum to a team mate for checking before it is written on the team sheet. If appropriate, the teams could be racing each other to get as many totals as possible, but organise a penalty system for errors (thus really encouraging the checking process!).
Teachers may like to take a look at the article Adding and Subtracting Negative Numbers
Possible extension
The extension task in the main problem is suitable here.
