Two trains
Two trains started simultaneously, each travelling towards the other. How long did each train need to complete the journey?
Problem
A train started at Amityville and travelled at a constant velocity towards Brookville. At the same time, a train started at Brookville and travelled at a constant velocity towards Amityville.
After they met, the first train travelled for nine more hours before reaching Brookville, and the second train travelled for four more hours before reaching Amityville.
For how long did each train travel altogether?
This problem is taken from the World Mathematics Championships
Student Solutions
Answer: $10$ hours and $15$ hours
Using ratio
Image

Ratios $4 : k$ and $k : 9$ are equivalent $\Rightarrow k = 6$
(if $k=6$ isn't obvious you can get it from $\frac4k=\frac k9\Rightarrow k^2 = 4\times9$)
So journeys are $6+9$ and $6+4$ hours long.
Using fractions of the total journey times
Image

The fraction of the journey that the red train completes in $k$ hours is the same as the fraction of the journey that the blue train completes in 4 hours.
$$\begin{align}\dfrac k{k+9}&=\dfrac 4 { k+4}\\
\Rightarrow k (k+4)&=4(k+9)\\ \Rightarrow k^2+4k&=4k+36\\ \Rightarrow k^2 &= 36\\
\Rightarrow k &=6\end{align}$$ So journeys are $6+9$ and $6+4$ hours long.
Using distance = speed $\times$ time
Suppose that the train from A to B travels at $a$ km per hour, and the train from B to A travels at $b$ km per hour. Then this diagram shows the distances travelled by each train in each part of the journey:
Image
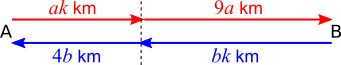
So $ak=4b$, and $9a=bk$.
We can make $a$ (or $b$) the subject of the first equation, and substitute it into the second: $$\begin{align}a&=\dfrac{4b}{k}\\ \Rightarrow 9\frac{4b}k &= bk\\
\Rightarrow 9\times 4b &= b\times k^2\\
\Rightarrow 36 &= k^2\end{align}$$
So $k=6$, which means the journey from A to B is $15$ hours long, and the journey from B to A is $10$ hours long.We can make $a$ (or $b$) the subject of the first equation, and substitute it into the second: $$\begin{align}a&=\dfrac{4b}{k}\\ \Rightarrow 9\frac{4b}k &= bk\\
\Rightarrow 9\times 4b &= b\times k^2\\
\Rightarrow 36 &= k^2\end{align}$$
