Three Squares
What is the greatest number of squares you can make by overlapping three squares?
Problem
What is the greatest number of squares you can make by overlapping three squares of the same size?
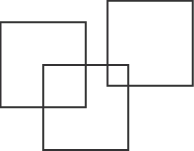
We would also like to know how you go about the task. How will you know if your way really does have the greatest possible number of squares?
You could use this interactivity to try out your ideas:
Getting Started
What do you know about squares? Are you sure all the shapes you are counting are squares?
How will you count the squares? It might help to draw the arrangement and use coloured pencils or pens to outline the squares. Squared paper will be useful!
Student Solutions
We had quite a lot of solutions come in for this task. I've shown here the ones who have seen that overlapping in a diagonal way produces the most squares. Other arrangements were not able to find nearly as many squares.
Children from St Joseph's Corinda Australia sent in their solutions:
We quickly figured out that the squares won't work horizontally or vertically, so we place them diagonally. By touching the first two squares, and overlapping the third square in the middle, we had three big squares and four small squares in the middle for a total of seven squares. We then realised that if we overlapped the first two squares, we would create an extra square in the middle for a total of eight.
For four squares, we continued with overlapping diagonally. We added a square at the bottom and managed to get a total of fifteen squares.

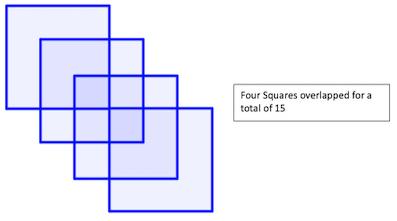
Mrs. Harrell's Crew from Glenolden School in the U.S. wrote:
We realized from the example that we would have to overlap even more to try to get more than just five squares.
We tried to first overlap all three squares with at least one corner in common. At first, we were excited to have gotten what we thought was six squares, but then we noticed there were more than just three big squares and three tiny squares, there were also two medium squares.
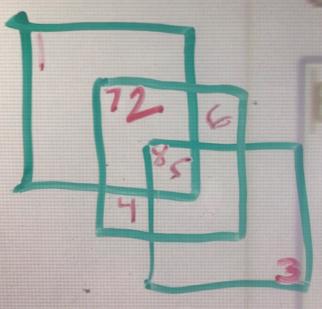
Andy from the American International School of Guangzhou (AISG) in China said:
In this problem, we had to make the most squares as it is possible. I made eight squares using three squares and using four squares I made 13 squares.
You play this game like you have to overlap the squares and make many squares. My method was to keep trying different shapes with squares.
I got eight squares by putting two square edge to edge and put the last one in the middle.
I got 13 squares by same as how I got eight squares but I put one more in the bottom.

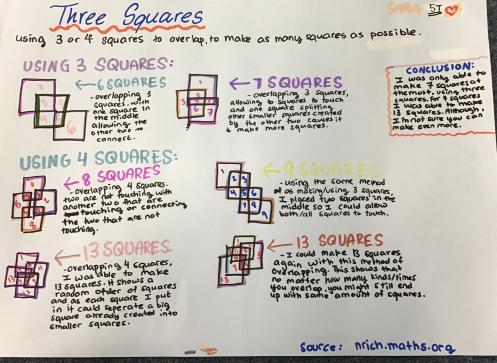
Sara also from the American International School Of Guangzhou (AISG) said:
For this problem, I was able to make seven squares using three squares and 13 using four squares. First, using three squares I placed one square in the middle and placed another on top with a rectangle in the middle of the two squares. By doing this, I was able to split the rectangle in two creating two extra squares. Also, by placing the third square in the middle, I was able to create another two extra squares. Adding the next three squares originally, creating seven squares. (See picture below.)
Using four squares, I made 13 squares. I found two different ways to overlap the squares but still ended up with 13 squares. I placed, again, a square in the middle and placed two on the top left and right side of the first square. And, on top of that the last of the four squares.
Abbots Langley School Maths Club sent in their thoughts:
We think that the highest number of squares is eight.
To work this out we overlapped the squares as many times as we could. We realised that in order to get the highest number of squares we would need to overlap every square with the result being a square.
Clive, James and Harry from Christchurch in New Zealand sent in a different solution for the version of this problem with four squares:
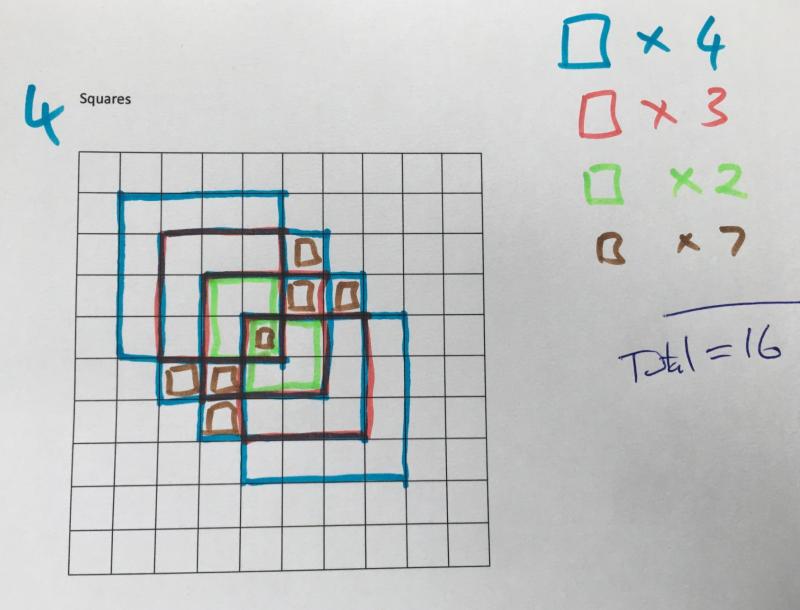
We think we have 16 squares comprising 4 large squares (the ones we started with), 3 squares that are ¾ the original size, 2 squares that are ½ the original size and 7 squares that are ¼ the original size. (The fractions of the original sizes are referring to the side lengths of the squares rather than the areas.)
This picture and key make it really clear that you have arranged these in a way that gives 16 squares altogether. I think this is the most squares that can be made with the four large ones - well done!
Teachers' Resources
Why do this problem?
This problem is fantastic for reinforcing the properties of a square. The interactivity will enable learners to access the task immediately so they can easily begin to explore. This in turn means that they are much more likely to become curious about the challenge of finding as many squares as possible, so are motivated to work mathematically. The interactivity not only supports the exploratory nature of the problem, but also helps to deepen children's understanding of what makes a square a square.
Possible approach
Key questions
How do you know that it isn't possible to make more squares?
Possible extension
Possible support
Learners could start by looking at two squares and using the interactivity will help.
