Sum of 1s
Can you find the last 5 digits of this sum?
Age
11 to 14
Challenge level
Problem
What are the last 5 digits of the sum $1+11+111+1111+\ldots+\underbrace{111...111}_{\text{2016 digits}}$?
This problem is adapted from the World Mathematics Championships
Student Solutions
Answer: 56566
Writing it out in columns
In the units column, there are 2016 1s:
Image
In the tens column, there are 2015 1s and 201 carried from before
2015 + 201 = 2216
Image
In the hundreds column, there are 2014 1s
2014 + 221 = 2235
Image
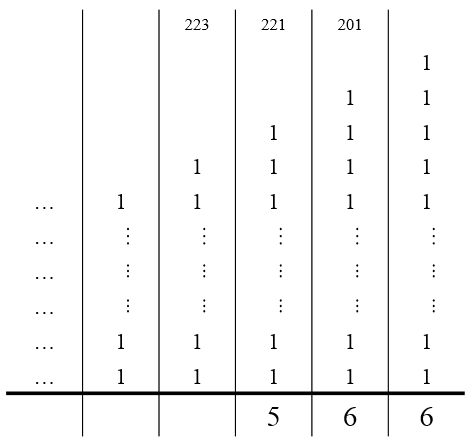
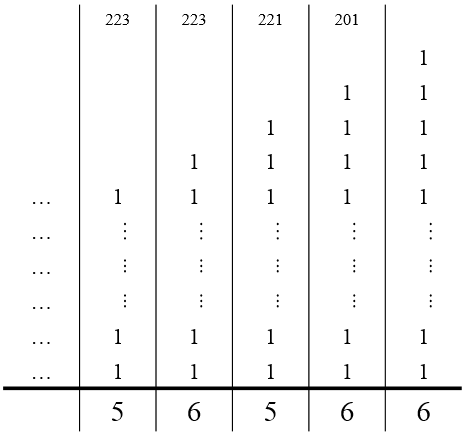
Continue:
Image
Image
Using place value
The units (ones) digit of every number in the list is 1, so there are 2016 units (ones) in the sum.
The first number doesn't have a tens digit, but the tens digit of every other number is 1, so there are 2015 tens from the tens digits in the sum.
And so on.
This is filled in in the table below, which shows the place value of each digit. At the bottom, adding the digits in each of the last five columns gives the last five digits of the sum, which are 56566.
| TTh | Th | H | T | U | |||
| 2 | 0 | 1 | 6 | ||||
| 2 | 0 | 1 | 5 | ||||
| 2 | 0 | 1 | 4 | ||||
| 2 | 0 | 1 | 3 | ||||
| 2 | 0 | 1 | 2 | ||||
| 5 | 6 | 5 | 6 | 6 |