Squares and Cubes
How many positive two-digit numbers are there whose square and cube both end in the same digit?
Age
11 to 14
Challenge level
Problem
How many positive two-digit numbers are there whose square and cube both end in the same digit?
This problem is taken from the UKMT Mathematical Challenges.
Student Solutions
Answer: 36
When two numbers are multiplied, the units digit of the product is always the same as the units digit of the product of the units digits. Click below to see why.
This can be seen by considering a 'grid' method, in this example to multiply 47 by 382. The units digit is 4, because 7$\times$2 is 14 which has units digit 4.
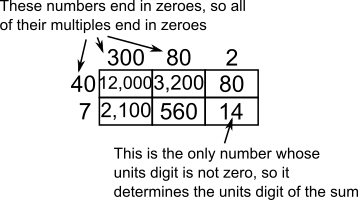
Image
| last digit of number | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 |
|---|---|---|---|---|---|---|---|---|---|---|
| last digit of square | 0 | 1 | 4 | 9 | 6 | 5 | 6 | 9 | 4 | 1 |
| last digit of cube | 0 | 1 | 8 | 7 | 4 | 5 | 6 | 3 | 2 | 9 |
Numbers ending in 0, 1, 5 or 6
There are 9 two-digit numbers ending in each digit (e.g. 10, 20, 30, ..., 90)
That gives a total of 36 numbers.