The square under the hypotenuse
Problem

The Square Under the Hypotenuse printable worksheet - initial problem
The Square Under the Hypotenuse printable worksheet -different methods
This right-angled triangle has a base of 3 and a height of 6 units.
How might you construct the square, which just touches the hypotenuse?
Can you work out the side length of the square?
Can you think of more than one way to work it out?
What if the side lengths of the triangle were 12 and 4 units long?
What if they were $a$ and $b$ units long?
Once you've had a go at solving this, click below to reveal three different approaches.
Can you take each starting point and turn it into a solution?
Method 1
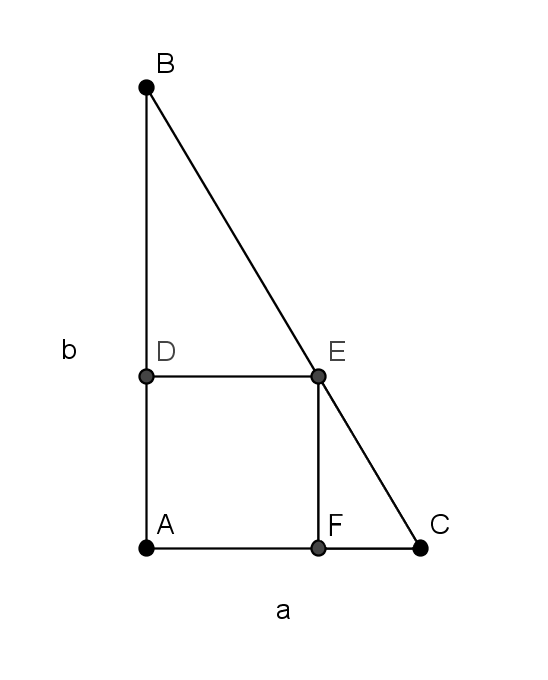
There are some similar triangles in the image below.
How could you use these similar triangles to find the side length of the square?
Method 2
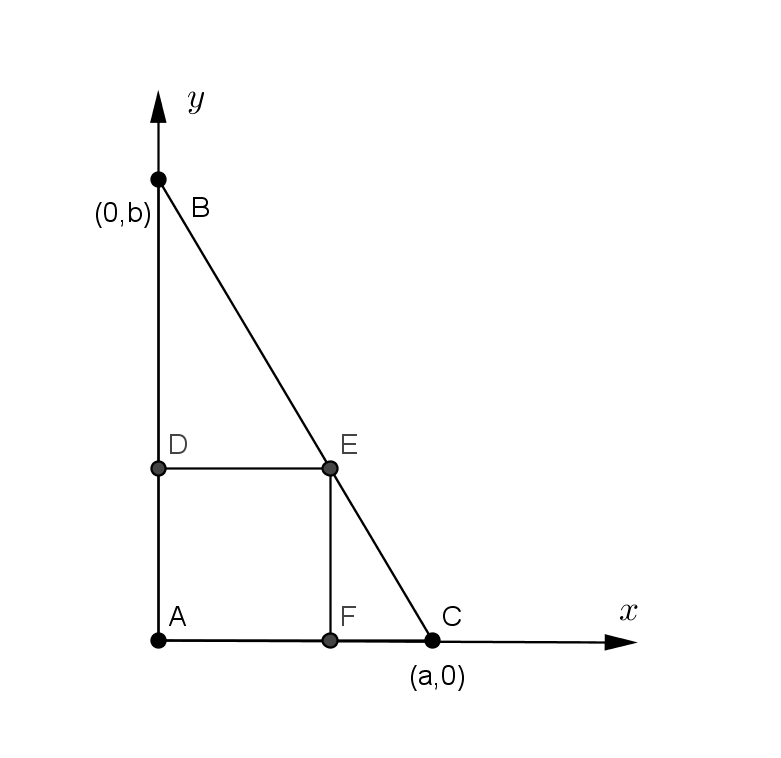
We can draw the triangle on a set of coordinate axes.
What is the equation of the line $BC$?
What do we know about the coordinates of point $E$?
How could you use this to find the side length of the square?
Method 3
Can you see how to create the rectangle on the right from the rectangle on the left?
Find expressions for the areas of the two rectangles.
How could you use these expressions to find the side length of the square?


Getting Started
Method 1:
If the square has side length $x$, what are the side lengths of the smaller triangles?
How do we know the triangles are similar?
Using the fact that the triangles are similar, can we write any equations involving $x$?
Method 2:
If we draw the triangle on a coordinate grid, with the right angle at the origin, what are the coordinates of the vertices of the triangle?
How can we work out the equation of the hypotenuse?
What would the equation of the diagonal of the square be?
Method 3:
What is the length and width of the original rectangle?
What are the dimensions of the rearranged rectangle?
Can you use the fact that the areas are the same to write an equation?
Student Solutions
This was a very popular problem! We received correct full solutions, or partial solutions, from Zhifang and Sevinch from St George's International School Rome; Carlos at King's College, Alicante; Sanika at PSBBMS in India; Shayan and Angus at St Stephen's School, Carramar; Sammy at Lancing College; Max at West Island School in Hong Kong; Xinrong at Howell's School in Wales; Arnik and Viktor at Wilson's School; Chamundeshwari from Wolsey Hall in India; Dibyadeep from Tanglin Trust School in Singapore; and from Wendy, Ananya and Ana (do get in touch to tell us your schools). Well done, everyone.
Exploring different ways to solve a problem can be a fascinating process. Knowing alternative methods can help you to build strong connections between different areas of mathematics as well as ensuring that you have other approaches to explore when you get stuck (which happens to us all!).
This particular problem challenged you to explore three different methods and we received solutions for each of the methods. Clearly some of you preferred one method over another, depending on your past experiences and favourite ways of working. Do read through each of the approaches below and perhaps try them out for yourself.
Method 1
Method 1 suggested exploring an approach using similar triangles. This was a popular approach, and it was pleasing to see how many of you remembered to check that you did indeed have similar triangles rather than simply assuming that they were similar.
Here's Sammy's approach for finding the length of the side of the square using Method 1:

Method 2
The hint for Method 2 suggested adopting an approach using co-ordinates. Here's Ana's solution for Method 2:

In this case, where a = 6 and b = 3,
p = $\frac{18}{9}$ = 2
Thank you, Ana.
Method 3
Method 3 suggested trying to create rectangles. Here's Sanika's approach using that method:

In this case, where A = 6 and B = 3,
$S = \frac{AB}{A+B} = \frac{18}{9}$ = 2
Having had a chance to read through these solutions, which use three very different methods, which do you prefer and why?
Teachers' Resources
Why do this problem?
Students often think that there is only one correct way to approach a geometrical challenge. This problem poses a geometrical problem and offers three starting points that lead to different methods to solve it. We hope this will encourage students to reflect on what might be their preferred approach and why, and for them to develop a flexible attitude to geometrical problem solving.
Possible approach

Start by showing this right-angled triangle, and inviting students to discuss how they would construct the square so that it just touches the hypotenuse. This could be a good opportunity to practise some construction techniques.
Next, pose the main problem; we could find the side length of the square by measuring, but this might not be accurate. Is there a way we can calculate the side length of the square using geometrical reasoning?
Give students some time to explore ideas. In the problem, there are starting points for three different methods:
- Using similar triangles
- Drawing the triangle on coordinate axes and finding the point of intersection of the hypotenuse with the line $y=x$
- Rotating the triangle to make a rectangle, then cutting up and reassembling the rectangle and equating areas.
These starting points could be used for the particular case of a triangle with side lengths 6 and 3, the suggested follow-up 12 and 4, and then for the general case with side lengths a and b. (We chose 6 by 3 and 12 by 4 as they give integer answers for the side length of the square.)
Once student have had a go at finding the side length of the square using all three methods, set aside some time to discuss which method they preferred, and why. This reflection will help them to develop a toolkit of geometrical problem-solving techniques that they can apply in future situations.
Key questions
Method 1:
If the square has side length $x$, what are the side lengths of the smaller triangles?
How do we know the triangles are similar?
Using the fact that the triangles are similar, can we write any equations involving $x$?
Method 2:
If we draw the triangle on a coordinate grid, with the right angle at the origin, what are the coordinates of the vertices of the triangle?
How can we work out the equation of the hypotenuse?
What would the equation of the diagonal of the square be?
Method 3:
What is the length and width of the original rectangle?
What are the dimensions of the rearranged rectangle?
Can you use the fact that the areas are the same to write an equation?
Possible support
Some students might find it helpful to draw the 6 by 3 and 12 by 4 triangles on squared paper to see the similar triangles and intersecting lines.
Possible extension
Students could consider which other dimensions of triangle will produce a square with a side length that is an integer.
Squirty explores construction of a square in any triangle, and might be a good follow-up challenge.
Compare Areas requires similar geometrical reasoning.
