Square to a rectangle
Kevin has moved some tiles to change the shape of his patio from a square to a rectangle. What are the lengths of the sides of the rectangle?
Problem
Kevin had some square patio tiles which he arranged to make a square.
He decided to change it into a rectangle by making one of the sides 3 tiles shorter, and the other side 4 tiles longer.
To make this change, Kevin only needed to buy one extra tile.
What are the lengths of the sides of the rectangle?
This problem is adapted from the World Mathematics Championships
Student Solutions
Answer: 10 tiles by 17 tiles
Finding an expression for the change in the number of tiles
Original patio:
Image
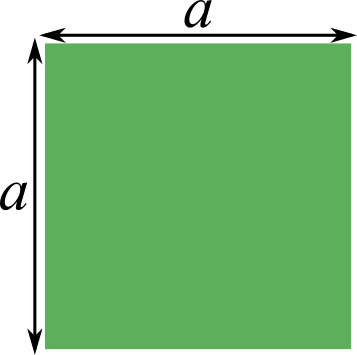
Shorten one side by 3 tiles:
Image
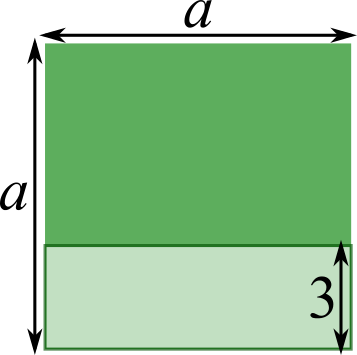
Other side 4 tiles longer:
Image
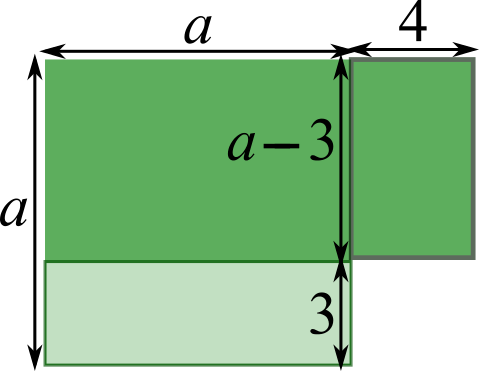
Needed 1 extra tile to make this change, so 4$(a-$3$)$ = 3$a$ + 1, so 4$a-$12 = 3$a$ + 1, so $a-$12 = 1, so $a$ = 13.
13 tiles by 13 tiles square $\Rightarrow$ 10 tiles by 17 tiles rectangle.
Using quadratic expressions for the total area before and after
Before: After:
Image

Image
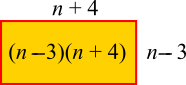
The new arrangement uses one extra tile, so $$\begin{align}n^2+1&=(n-3)(n+4)\\
n^2+1&=n^2+4n-3n-12\\
n^2+1&=n^2+n-12\\
1&=n-12\\
13&=n\end{align}$$
So Kevin had a $13$ tiles by $13$ tiles square, which means that he made a $10$ tiles by $17$ tiles rectangle.
Trying out numbers
The patio used to be a square, so we can test some square numbers to see whether they work.
If the patio started as a 10 tiles by 10 tiles square, then Kevin would have had 100 tiles.
Making one side 3 tiles shorter and the other side 4 tiles longer would have given him a 7 by 14 rectangle.
7$\times$14 = 98, so Kevin would have had 2 spare tiles if he had started off with a 10 by 10 square. But Kevin needed 1 extra tile, so that can't be right.
If the patio started as a 9 tiles by 9 tiles square, then Kevin would have had 81 tiles.
Making one side 3 tiles shorter and the other side 4 tiles longer would have given him a 6 by 13 rectangle.
6$\times$13 = 78, so Kevin would have had 3 spare tiles if he had started off with a 9 by 9 square. That is worse than the 10 by 10 square, because the 10 by 10 square would only have given Kevin 2 spare tiles. So Kevin's patio must have started as a square that was larger than 10 tiles by 10 tiles.
If the patio started as a 12 tiles by 12 tiles square, then Kevin would have had 144 tiles.
Making one side 3 tiles shorter and the other side 4 tiles longer would have given him a 9 by 16 rectangle.
9$\times$16 = 144, so Kevin would have had exactly the right number of tiles if he started off with a 12 by 12 square and wouldn't have needed to buy 1 extra tile.
If the patio started as a 13 tiles by 13 tiles square, then Kevin would have had 169 tiles.
Making one side 3 tiles shorter and the other side 4 tiles longer would give him a 10 by 17 rectangle.
10$\times$17 = 170, so Kevin would need 1 extra tile to make the rectangle - which is right!
So he must have made a 10 tiles by 17 tiles rectangle.
