square overlap
The top square has been rotated so that the squares meet at a 60$^\text{o}$ angle. What is the area of the overlap?
Problem
Image
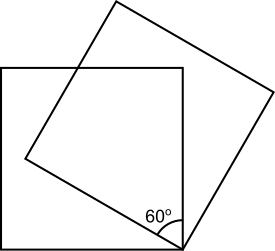
Two squares of side length 2 cm are placed on top of each other so that the angle between the squares is 60$^\text{o}$.
What is the area of the overlap?
This problem is adapted from the World Mathematics Championships
Student Solutions
Splitting the overlap into right-angled triangles

Both of these right-angled triangles have an angle of $30^\text{o}$ adjacent to a $2$ cm side.
The opposite side can be found using the trigonometry, and then the area of each triangle will be $\frac{1}{2}\times$base$\times$height, which will be half of the product of these two sides.
$\tan{30}=\dfrac{\text{opp}}{\text{adj}}=\dfrac{\text{opp}}{2}\Rightarrow\text{opp}=2\tan{30}=\dfrac{2}{\sqrt3}$
So the area of each triangle is $\dfrac{1}{2}\times2\times\dfrac{2}{\sqrt3}$, so the area of the overlap is $2\times\dfrac{2}{\sqrt3}=\dfrac{4}{\sqrt3}$cm$^2,$ or $\dfrac{4\sqrt3}{3}$cm$^2,$ which is approximately $2.31$cm$^2.$
Splitting the overlap into other triangles
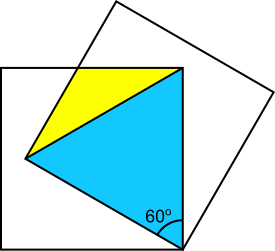
The area of the blue triangle can be found using $\frac{1}{2}ab\sin{c}$, where $c=60^\text{o}$ and $a=b=2,$ because $a$ and $b$ are the sides of the squares.
$\frac{1}{2}\times2\times2\times\sin{60}=\sqrt3,$ so the area of the blue triangle is $\sqrt3$cm$^2$ $\approx1.73$cm$^2.$
Since the blue triangle is isosceles and has a $60^\text{o}$ angle, it must be equilateral. So the 'base' of the yellow triangle (as shown below) must also be $2$ cm. The angles labelled are each $30^\text{o}$ because they make $90^\text{o}$ when added to the angles in the blue triangle.
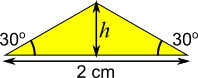
$h$ can be found using trigonometry, and then the area of the yellow triangle will be $\frac{1}{2}\times2\times h=h$cm$^2.$
$\tan{30}=\dfrac{\text{opp}}{\text{adj}}=\dfrac{h}{1}=h,$ so $h=\dfrac{1}{\sqrt3}=\dfrac{\sqrt3}{3}$ $\approx0.58$cm.
So the total area is $\sqrt3+\dfrac{\sqrt3}{3}=\dfrac{3\sqrt3}{3}+\dfrac{\sqrt3}{3}=\dfrac{4\sqrt{3}}{3}$cm$^2$ $\approx2.31$cm$^2.$
Image

Both of these right-angled triangles have an angle of $30^\text{o}$ adjacent to a $2$ cm side.
The opposite side can be found using the trigonometry, and then the area of each triangle will be $\frac{1}{2}\times$base$\times$height, which will be half of the product of these two sides.
$\tan{30}=\dfrac{\text{opp}}{\text{adj}}=\dfrac{\text{opp}}{2}\Rightarrow\text{opp}=2\tan{30}=\dfrac{2}{\sqrt3}$
So the area of each triangle is $\dfrac{1}{2}\times2\times\dfrac{2}{\sqrt3}$, so the area of the overlap is $2\times\dfrac{2}{\sqrt3}=\dfrac{4}{\sqrt3}$cm$^2,$ or $\dfrac{4\sqrt3}{3}$cm$^2,$ which is approximately $2.31$cm$^2.$
Splitting the overlap into other triangles
Image

The area of the blue triangle can be found using $\frac{1}{2}ab\sin{c}$, where $c=60^\text{o}$ and $a=b=2,$ because $a$ and $b$ are the sides of the squares.
$\frac{1}{2}\times2\times2\times\sin{60}=\sqrt3,$ so the area of the blue triangle is $\sqrt3$cm$^2$ $\approx1.73$cm$^2.$
Since the blue triangle is isosceles and has a $60^\text{o}$ angle, it must be equilateral. So the 'base' of the yellow triangle (as shown below) must also be $2$ cm. The angles labelled are each $30^\text{o}$ because they make $90^\text{o}$ when added to the angles in the blue triangle.
Image

$h$ can be found using trigonometry, and then the area of the yellow triangle will be $\frac{1}{2}\times2\times h=h$cm$^2.$
$\tan{30}=\dfrac{\text{opp}}{\text{adj}}=\dfrac{h}{1}=h,$ so $h=\dfrac{1}{\sqrt3}=\dfrac{\sqrt3}{3}$ $\approx0.58$cm.
So the total area is $\sqrt3+\dfrac{\sqrt3}{3}=\dfrac{3\sqrt3}{3}+\dfrac{\sqrt3}{3}=\dfrac{4\sqrt{3}}{3}$cm$^2$ $\approx2.31$cm$^2.$
