Snooker Frames
It is believed that weaker snooker players have a better chance of
winning matches over eleven frames (i.e. first to win 6 frames)
than they do over fifteen frames. Is this true?
Age
16 to 18
Challenge level
Problem
A single game of snooker is called a frame. In the first round of a snooker tournament, the matches are played over eleven frames (so the first player to win six frames wins the match). In the later rounds matches are played over 15 frames. Assume that each player has steady nerves and his chance of winning any frame (irrespective of who starts) is constant.
In the problem Snooker you were asked to find the probability that a player wins a match over fifteen frames, given that his chance of winning any frame is $0.4$. You should now find the probability that this player wins a match over eleven frames.
It is believed that the weaker players have a better chance of winning the matches over eleven frames than they do over fifteen frames. Do your results confirm this or not?
Does this surprise you, or not? Why?
Numerical
investigation: Is it generally the case that more frames
lead to a reduced chance of a weaker player winning? Devise a
spreadsheet which computes the chance of a weaker player winning a
match in which the first player to $1, 2, 3, 4, \dots, 17, 18$
frames wins. The world snooker championship final is taken over the
best of $35$ frames. In order to have at least a $10\%$ chance of
winning such a final, what probability of winning each frame would
you need to have? Plot $\log(n)$ against $P(win)$.
Getting Started
The method for solving this problem is given in the solution to the problem Snooker.
You have to compare the probabilities of winning a match which is the best out of 11 frames with one that is the best out of 15 frames. In the first case the first player to win 6 frames wins the match and in the second case the first to win 8 frames wins the match.
Assume that each player has steady nerves and his chance of winning any frame (irrespective of who starts) is constant.
You can use the results in the problem Snooker for the probability that a player wins a match over 15 frames, given that his chance of winning any frame is $0.4$. All you have to do here is to use a similar method to work out the probability that this player wins a match over 11 frames. It is believed that the weaker players have a better chance of winning the matches over eleven frames than they do over fifteen frames. Do your results confirm this or not?
Student Solutions
Ben has solved this Tough Nut. He did not say which school he comes from.
The probability of winning a 15 frame match was shown in the problem Snooker to be $0.2131$ for a weaker player who has a consistent probability of $0.4$ of winning each single frame. We use the same method here.
To win an 11 frame match the player must be the first one to win 6 frames. He may win 6 games outright or win any 5 of the first 6 games and lose one then win one, or any 5 of the first 7 games and lose 2 then win one, or any 5 of the first 8 games and lose 3 then wins one or any 5 of the first 9 games and lose 4 then win one or any 5 of the first 10 games and lose 5 then win one. The probability is $$p^6 + {6\choose 5}p^5(1-p)p + {7 \choose 5}p^5(1-p)^2p + {8 \choose 5}p^5(1-p)^3p+{9\choose 5}p^5(1-p)^4p+ {10 \choose 5}p^5(1-p)^5$$ For $p=0.4$ and $1-p=0.6$ this becomes $$0.4^6[1 + 6 \times 0.6 + 21 \times 0.6^2 + 56 \times 0.6^3 + 126 \times 0.6^4 + 252 \times 0.6^5 = 0.2465018$$ As $0.2465 > 0.2131$ this result gives evidence that weaker players are more likely to win 11 frame matches than they are to win 15 frame matches.
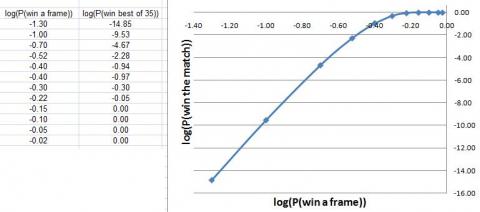
James from Bay House school did a numerical investigation and showed that the chances of winning the tournament were very slight with a 0.4 chance of winning each frame.
Even with a 40% chance of winning each frame, the chance of being the first to 18 frames is less than 1 in 10 million!
Image
We can also look at the chance of being the first to 18 frames for different probabilities of winning each frame.
Image
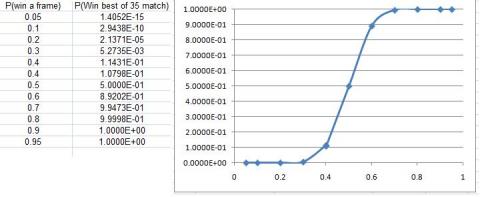
If you plot a log-log graph then you can see a highly linear relationship for values of probability less than about $90$ percent.
Image
Teachers' Resources
Why do this problem?
This problem gives practice in a relatively complicated
probability calculation drawn from a simple situation. It will
require clear visualisation of the possibilities, accurate working
and a good understanding of permutations and combinations. It gives
a good chance to practise calculator skills and the use of the
$^nC^r$ button. The context will allow a discussion concerning the
role of intuition versus calculation in probability.
Possible approach
Before beginning this problem ask students whether they think
that a weaker player is more or less likely to win over a long
match. Can they explain why they believe that this is the case? Are
these arguments completely convincing to the rest of the class. Can
they estimate how likely they believe that the weaker player is to
win over $11$ frames? Perhaps each student could make a guess. The
closest guess without going above wins.
In probability, calculation is used to settle disputes. Before
students solve the problem they will need to be very clear as to
the combinations of matches which lead to a win for the weaker
player. They will then need to write this down in a clear way
before working out the numbers. Spreadsheets or calculators will be
necessary for this. As the calculation is relatively long it will
require good calculator or recording skills successfully to obtain
the answer, even if the route to the answer is conceptually clear
to students. It might provide an opportunity explicitly to practise
the use of calculator keys such as ANS, $^nC^r$ and $!$. Can
students encode the entire calculation in a single line of
calculation?
Once the answer is found, compare with the initial estimates
that the class made. Whose intuition was reliable?
Key questions
Before attempting the computation, can you estimate the chance
of the weaker player winning the match?
If the weaker player only had a 4% chance of winning, what do
you feel would happen to his chances of winning the match as the
number of frames increases?
Which player must win the final frame of the match?
What are the possible final scorelines?
Possible extension
Can students write down a generalisation of the formula used
to show the chance of a player winning a match played over $2n+1$
frames? The numerical investigation will allow students to put
their formula into practice.
Possible support
Reduce the complexity of the calculation: what is the chance
of winning a best of $3$ or a best of $5$ match?