Reverse ages
When is the next time that Brian's age will be the reverse of his father's age?
Problem
On Brian's 14th birthday, his father was 41.
Brian noticed that his age was the reverse of his father's age.
How old will Brian be the next time his age is the reverse of his father's age?
This problem is adapted from the World Mathematics Championships
Student Solutions
Answer: Brian will be 25
Working it out by thinking about the numbers
Image
If Brian's age begins with 1, his father's age should end with 1
Brian's father's age won't end with 1 until Brian is more than 20
If Brian's age begins with 2, his father's age should end with 2
Adding the same number of years to each age
Image
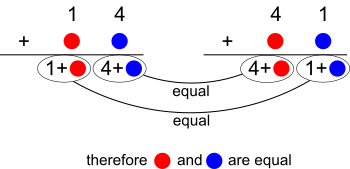
So their ages will be each other's reverses in 11, 22, 33... years time.
Next time is in 11 years, 14 + 11 = 25
Using algebra to find numbers that are each other's reverses and are the correct distance apart
Brian's age written $ab$, Brian's age is $10a+b$
Father's age written $ba$, Father's age is $10b+a$.
$41-14 = 27$ so Brian's father is $27$ years older than Brian.
$$\begin{align}10a+b+27&=10b+a\\
10a+27&=10b+a-b\\
10a-a+27&=10b-b\\
9a+27&=9b\\
a+3&=b\end{align}$$
| $a$ | $b$ | Brian's age | Father's age |
|---|---|---|---|
| 1 | 4 | 14 | 41 |
| 2 | 5 | 25 | 52 |
| 3 | 6 | 36 | 63 |
| etc. |
