Pool shot
Problem
Suppose we have a pool table without pockets and with side lengths $a$ and $2b$. The ball is placed at point A.
1) Find four different angles for the ball to get back to the same point A.
2) Find four possible angles for the ball to go to the point D.
3) This is a bit more challenging question. Find four different angles in each situation 1) and 2) if we have the same pool table but with pockets. The ball starts to move from the point A which is just in front of the pocket.
State any assumptions that you make.
Getting Started
Try to use symmetry to make a situation much easier.
Student Solutions
1) $\alpha$ could be equal to $\frac{\pi}{2}, tan^{-1}(\frac{a}{2b}), tan^{-1}(\frac{a}{3b}), tan^{-1}(\frac{a}{4b})$.
2) The suitable values of $\alpha$ could be $\frac{\pi}{2}, tan^{-1}(\frac{a}{2b}), tan^{-1}(\frac{a}{4b}), tan^{-1}(\frac{a}{8b})$.
You can check these angles by drawing diagrams. Actually there is an infinite number of possible angles.
3) In this situation we need to be careful that the ball will not go through any other hole before it goes through required one.
For the 2) case possible answers are the same.
For the 1) case use symmetry to help to understand what happens. We can add a number of pool tables like blocks and imagine that the ball is moving in a straight line. If the trajectory line goes through any point A, B, C, D, E, F it means that the ball stops at this point because it will be in the hole.
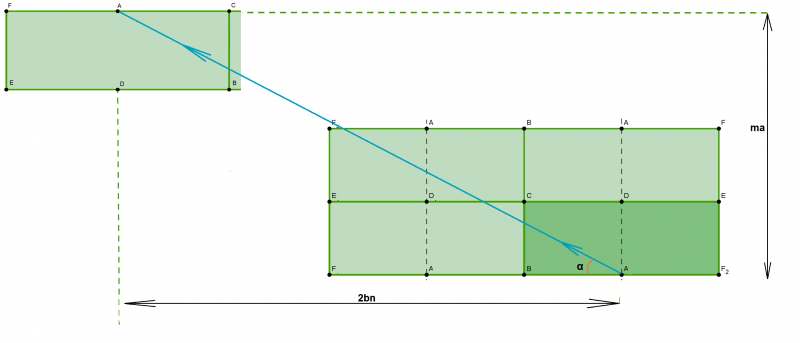
Suppose that the ball is moving along a line such that $\tan(\alpha) = \frac{ma}{2nb}$ where $m$ and $n$ are natural numbers. We see from the picture that $m$ and $2n$ must be even numbers in order for the ball to get back to a point A. Write $m = 2k$ and $2n$ is already even. $$\tan(\alpha) = \frac{2ka}{2nb} = \frac{ka}{nb}$$ Thus, we will go through the point (bn, ka). If $n$ and $k$ are both even then simplify again until you get that one of them is odd.
This means that the ball can't be hit in such a way that it will go back to the same position.
