Matching triangles
Can you sort these triangles into three different families and explain how you did it?
Problem
These triangles belong to three different families.
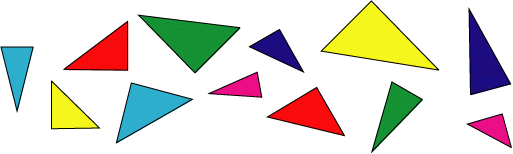
All the triangles in the same family are the same shape.
But they may not be the same size or the same way up.
Can you sort them out and explain how you did it?
You could print off pictures of the triangles (here as a Word document or here as a pdf), then cut them out and sort them practically. Or if you prefer, you could use the interactivity below to try out your ideas:
Getting Started
Try concentrating on one triangle at a time. Perhaps you can imagine moving it around in your head so that it is a different way up?
You could print off this sheet of the triangles, then cut them out to sort them. (The first page is in colour, the second in black and white.)
Student Solutions
This problem was more complicated than it first appeared! We had this solution sent in from Dhruv, who is homeschooled in the UK:
Dhruv has looked at the angles within each triangle, and has also labelled the three groups according to whether they are equilateral, isosceles or scalene. Well done Dhruv, you're correct that the triangles in the middle group have angles which are all less than 90 degrees! The triangles on the left do each have an angle of 90 degrees, but they aren't all scalene. Can you see which ones belong in the group on the right instead?
Harry from Copthorne Prep School in the UK sent in a picture of his three families of triangles, with some thoughts:
First Harry thought of sorting the triangles by colour but noticed that would give more than the three families specified. He then remembered he’d heard of an equilateral triangle that had equal sides and identified 4 from the selection that had equal sides. Harry then looked at the remaining triangles and identified a group of tall, thin triangles. On closer inspection they had two sides equal in length.
The final four therefore had to be the third family. They didn’t appear to have any sides of equal length.
We discussed whether length of the sides was the only ‘same’ thing about the equilateral triangles. Harry remembered that the internal angles must add up to 180 degrees and the angles all looked the same in this triangle meaning they’d be 60 degrees each. It wasn’t so easy to be able to say what the angles on the other types would be, but they would all always total 180 degrees.
Harry looked into what names the other types of triangle families might have and learned the triangle with two sides the same is called Isosceles and the triangle with no sides the same is called a Scalene triangle.
Good ideas, Harry! These three groups are the families that we were thinking of, but I'm not sure that the triangles in the group on the left are equilateral triangles. How could I check?
Emily from St James School said:
I think that these triangles are sorted into right-angled scalene, right-angled isosceles and non-right-angled isosceles.
There are no equilateral triangles because they do not ever have any right angles and none of those triangles had the same sides and angles.
Well done Emily, those are the correct labels for the three families!
Which way round would those three labels go on Harry's diagram?
Teachers' Resources
Matching Triangles
These triangles belong to three different families.
All the triangles in the same family are the same shape.
But they may not be the same size or the same way up.
Can you sort them out and explain how you did it?
You could print off pictures of the triangles (here as a Word document or here as a pdf), then cut them out and sort them practically. Or if you prefer, you could use the interactivity below to try out your ideas:
Why do this problem?
This activity is a good one to try with young children once they are familiar with the properties of a triangle. Often, they associate the name 'triangle' with a shape in a particular orientation and this problem is an excellent way to challenge this assumption. Other children may dismiss all three-sided shapes as triangles without looking at their other attributes. The activity will require pupils to look carefully at each shape and scrutinise its properties.
Possible approach
You could start by asking the group to tell you what they know about triangles. You could then ask one child to draw a triangle on the board and ask someone else to draw a different triangle. Invite the group to talk about what is the same and what is different about them. In this way, the discussion will include shape, size and orientation, but you could draw some triangles yourself to bring out certain aspects.
Next you could project the interactivity onto the interactive whiteboard, or show them the triangles on these sheets. (The first page has the triangles in colour, the second in black and white so that it can be photocopied.) Introduce the task and encourage the group to work in pairs with a set of cut-out triangles so that they are able to talk through their ideas with a partner. Listening to their justifications can reveal a lot about their understanding of similar triangles, even though this terminology is not used.
You can draw the class together for mini plenaries, as appropriate, perhaps to share misunderstandings or ways of working. You may wish to use the interactivity for drawing attention to particular triangles (it does not allow you to rotate the triangles). Once the majority of pairs have grouped their triangles in some way, invite the class to wander around the room looking at the arrangements. What do they notice? Do they have any questions? The interactivity could be used again to re-create a solution.
Key questions
Possible extension
Children could draw their own families of triangles and label the differences and similarities.
Possible support
Use one of these sheets so that the triangles can be cut out, then rotated and placed on top of one another. (The first page has the triangles in colour, the second in black and white.)