Magic Letters
Problem
Magic Letters printable worksheet
The problem is explained below, but you may wish to go to the Getting Started page to watch a video of the NRICH team presenting the initial challenge.
Charlie has made a Magic V with five consecutive numbers:

It is a Magic V because each 'arm' has the same total.
Alison drew this magic V:

Charlie said "That's really just the same Magic V as mine!"
What do you think Charlie meant?
There are six more Magic Vs that are the same as Charlie's - can you find them all?
Click below to check you have.
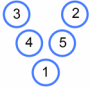
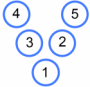
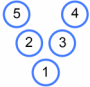
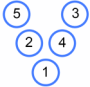
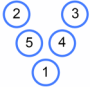
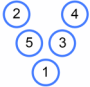
Can you find all the possible Magic Vs that use the numbers $1$ to $5$ and are different from Charlie's?
How can you be sure you have found them all?
What happens if you use the numbers from $2$ to $6$? Or from $3$ to $7$? Or $4$ to $8\dots$?
Here is a spreadsheet to help you investigate Magic Vs made from five consecutive numbers.
Can you describe an efficient strategy to find all Magic Vs for any given set of numbers?
Can you use your strategy to find all the possible Magic Vs that use the numbers $987, 988, 989, 990, 991$?
Can you find a Magic V where each arm has a total of 60? Or 1000? Or...?
Can you find more than one?
Can you find a Magic V for any arm total?
Charlie and Alison drew some more letters, to see if they could make them magic - that is, using consecutive numbers to make equal arm totals.
| Magic L? | Magic N? | Magic W? |
Image

|
Image

|
Image

|
Investigate some of these Magic Letters in the same way that you explored Magic Vs.
What general conclusions can you reach?
You can use this spreadsheet to explore.
Click here for a poster version of Magic Vs.
Getting Started
What do you notice about the number at the base of the V in all your magic Vs?
Here is a video of the NRICH team presenting the initial challenge. You could just watch the start to check that you understand the problem, or you may like to pause the video and work on the task at various points.
Student Solutions
This was an interesting problem that was open to a lot of interpretation, so many of you came up with new and inventive ways of answering the question, or answered questions we hadn't even asked - it's great to see that you all enjoyed the challenge!
Many people gave ideas as to why Charlie might think that Alison's Magic V was the same as his. Shreya, from Claremont High School, wrote:
Charlie's V had 3, 4 and 1 down one side and 5, 2 and 1 down the other side, so each side added up to 8. Alison's V also had the numbers from 1 to 5 arranged in such positions so that one side of the V added up to 8. Therefore, she had the same magic V as Charlie had!
Ben, from Wilson's Grammar School, had the same thoughts. Ayngharran and Rohan, also from Wilson's, thought something a little more specific. Ayngharran said:
Charlie used the same numbers as Alison, with the same 'middle' number 1, and the same numbers on each side but just in a different order. So the pairs 3, 4 and 5, 2 are the same in both cases.
Zoya, from St. Hilda's C of E Primary School, said:
You will know if you have found all the Magic Vs adding up to a certain number, as each Magic V will always have eight ways of rearranging the arms to still add up to the same total.
Elliott, from Solihull School, found a different Magic V with the same numbers:
| 2 | 1 | |||
| 3 | 4 | |||
| 5 |
Ben also found a similar Magic V. Some people made interesting comments about what sorts of Magic Vs might exist with these numbers. Abopakr, from Globe Academy, said:
The base can only be an odd number if you are using numbers 1 - 5, because if you put an even number at the base there will be only one even number not at the base. So it has to be an odd number at the base. Whichever is the most common kind of number - even or odd - is the kind that goes at the base.
Michelle, also from Globe Academy, had the same idea, and so did Utkarsh, from Sancta Maria International School. Murat and Callum, from Globe Academy, said:
You need to put the lowest number on the same side as the highest number.
Adam, from Collis Primary School, made the following Magic V and commented:
| 4 | 5 | |||
| 3 | 2 | |||
| 1 |
All you do is start at the bottom, then zigzag up and right, then left, then up and left, then right.
That's a nice systematic way of constructing a Magic V - well spotted! (I wonder whether we can make bigger Magic Vs in this way?)
Kavi, from Wilson's, suggested:
To construct a Magic V with the numbers 2-6, just take a Magic V with the numbers 1-5 and raise each number by 1. Then each arm will sum to 3 more.
Maciej, from Wilson's, gave us the following example:
| 4 | 3 | |||
| 5 | 6 | |||
| 2 |
An anonymous student in the UK gave the following example:
| 7 | 9 | |||
| 8 | 6 | |||
| 5 |
Ben solved our 987-991 puzzle in the same way:
| 988 | 987 | |||
| 989 | 990 | |||
| 991 |
Some people came up with different types of Magic Vs. For example, Daniel, from Wilson's, solved our '1000' puzzle in this way:
What I did was start with a magic V I already knew:
| 2 | 1 | |||
| 3 | 4 | |||
| 5 |
Each arm had a total of ten. I then multiplied each number in the V by 100.
| 200 | 100 | |||
| 300 | 400 | |||
| 500 |
Interesting - this doesn't use consecutive numbers, but it's still a Magic V.
Shavindra, from Wilson's, gave us a formulaic way of finding a Magic V with each arm summing to N (as long as N is divisible by 3):
| (N/3) + 1 | (N/3) + 2 | |||
| (N/3) - 1 | (N/3) - 2 | |||
| (N/3) |
Ellis, from Westfield Middle School, gave us a Magic L, and a comment:
| 1 | ||
| 3 | ||
| 4 | ||
| 5 | 2 | 6 |
The number in the corner must be odd. After you've done one, you can jumble the numbers on each arm.
Finally, Rebecca and Angus came up with this observation:
If you take the middle number of the five consecutive numbers for a Magic V and multiply it by 3, it will give you the middle number of the range of totals you could get for that magic V.
Also, there are only 3 consecutive totals for each V depending on which number is on the bottom of the V.
For example, for numbers 12-16, the middle number is 14.
14 times 3 is 42, so the range of totals is 41 to 43.
Excellent! Thanks to everyone for all your contributions.
Teachers' Resources
Why do this problem?
Possible approach
Here is a video of the NRICH team presenting the challenge.
Draw 5 circles on the board in a V shape, and give students mini-whiteboards or paper.



- As the numbers from 1 to 5 add up to an odd total, the repeated number at the bottom must be odd.
- If we put a 2 or a 4 at the bottom, we'd have an odd total left over to split between the two arms, so it can't be done equally.
- If there was an even number at the bottom, there'd be three odds and one even left, so they can't be split to make the same total on each side.
Possible support
Possible extension
