How steep is the slope?
On the grid provided, we can draw lines with different gradients. How many different gradients can you find? Can you arrange them in order of steepness?
Problem
How Steep is the Slope? printable sheet
How Steep is the Slope? printable grid
The gradient of a line tells us how far up or down we go when we take one step to the right:

On a grid like the one below we can draw lines through two points with different gradients.
Check you agree that the blue line is one of several that could be drawn with a gradient of 2 and the red line is one of several that could be drawn with a gradient of $-\frac{2}{3}$
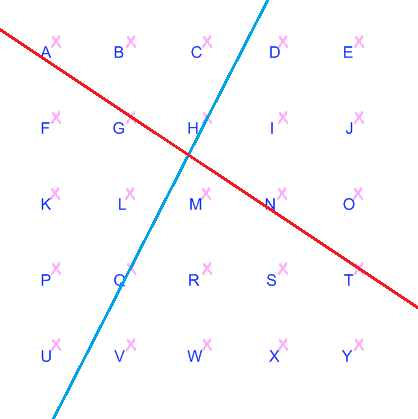
Picture some more lines with different gradients. You may want to use this sheet to record your working.
How many different gradients can you find? Can you find them all?
Arrange them in order of steepness and list the points each line passes through.
Getting Started
Position your ruler on U. Which point should you join to in order to get the steepest line possible? The next steepest? And the next?
Now think about where you could fix one end of your ruler and move the other end in order to make all the possible negative gradients.
Student Solutions
Jordan from Paul H. Cale Elementary listed 24 gradients that could be made on the grid after coming up with a systematic method to find them and correctly identified that the gradient of a vertical line is undefined (as it requires division by zero).
Once I understood the problem I printed out the work page and started just drawing random lines, this did not work so I looked at your hint and tried it the way it said to (make all the lines you can that connect to another point from U) and, it worked!! Once I got the hang of it, it went really fast!! I didn't even have to figure out the negative ones because I knew that they would be the same as the positive ones, just negative.
All the possible positive ones are:
| $4$ | $\frac{1}{3}$ |
| $3$ | $\frac{1}{4}$ |
| $2$ | $0$ |
| $1 \frac{1}{2}$ | |
| $1 \frac{1}{3}$ | |
| $1$ | |
| $\frac{3}{4}$ | |
| $\frac{2}{3}$ | |
| $\frac{1}{2}$ |
and the negative ones....
| $-4$ | $-\frac{1}{3}$ |
| $-3$ | $-\frac{1}{4}$ |
| $-2$ | $-0$ |
| $-1 \frac{1}{2}$ | |
| $-1 \frac{1}{3}$ | |
| $-1$ | |
| $-\frac{3}{4}$ | |
| $-\frac{2}{3}$ | |
| $-\frac{1}{2}$ |
The Gradient for a straight vertical line is undefinable.
Jordan lists both 0 and 0- as gradients - is there a difference?
Samantha from Aylesbury High School gave a very clear explanation of how all the different possible gradients could be identified
In a), I know I have found all of the positive gradients because, starting at point U, I have drawn lines to every other point. Some lines have the same gradient, but because they start from the same point they become the same line, but passing through several points. If I were to start from any point on the grid other than Y and draw a line from it to another point that line would have the same gradient as one which started from Y (or Y on the grid b).
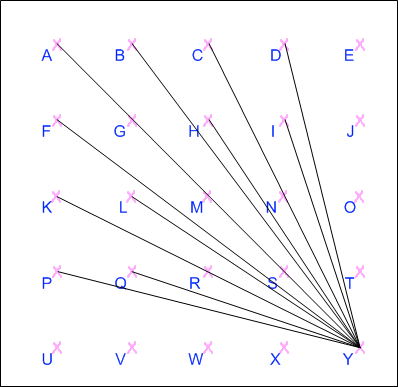
In b), I know I have found all of the negative gradients because, starting at point Y, I have drawn lines to every other point. Some lines have the same gradient, but because they start from the same point they become the same line, but passing through several points. If I were to start from any point on the grid other than Y and draw a line from it to another point that line would have the same gradient as one which started from Y (or U on the grid a). I have excluded the lines with a gradient of 0 and 8 because they are both on a).
These are all 24 of the gradients shown on the two diagrams, and the points they pass through:
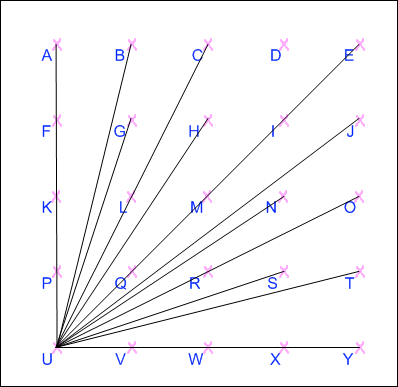
In a), I know I have found all of the positive gradients because, starting at point U, I have drawn lines to every other point. Some lines have the same gradient, but because they start from the same point they become the same line, but passing through several points. If I were to start from any point on the grid other than Y and draw a line from it to another point that line would have the same gradient as one which started from Y (or Y on the grid b).
In b), I know I have found all of the negative gradients because, starting at point Y, I have drawn lines to every other point. Some lines have the same gradient, but because they start from the same point they become the same line, but passing through several points. If I were to start from any point on the grid other than Y and draw a line from it to another point that line would have the same gradient as one which started from Y (or U on the grid a). I have excluded the lines with a gradient of 0 and 8 because they are both on a).
These are all 24 of the gradients shown on the two diagrams, and the points they pass through:
| Gradient | Points it passes through |
|---|---|
| $\infty$ | AFKPU |
| $4$ | BU |
| $3$ | GU |
| $2$ | CLU |
| $1.5$ | HU |
| $1 \frac{1}{3}$ | DU |
| $1$ | EIMQU |
| $0.75$ | JU |
| $\frac{2}{3}$ | NU |
| $0.5$ | ORU |
| $\frac{1}{3}$ | SU |
| $0.25$ | TU |
| $0$ | UVWXY |
| $-0.25$ | PY |
| $-\frac{1}{3}$ | QY |
| $-0.5$ | KRY |
| $-\frac{2}{3}$ | LY |
| $-0.75$ | FY |
| $-1$ | AGMSY |
| $-1 \frac{1}{3}$ | BY |
| $-1.5$ | HY |
| $-2$ | CNY |
| $-3$ | IY |
| $-4$ | DY |
Ryli from Aylesbury High School came up with a excellent method for deducing how many different gradients could be constructed on a 4 by 4 grid, looking at all possible combinations of steps and then discounting those which were repeated.
There are 24 different gradients.
You can go between 1 and 4 units (inclusive) horizontally (e.g. A-E) and between 1 and 4 units (inclusive) vertically (e.g. A-U) in any combination:
$$ \begin{array} & \frac{1}{1} & \frac{2}{1} & \frac{3}{1} & \frac{4}{1} \\ \frac{1}{2} & \frac{2}{2} & \frac{3}{2} & \frac{4}{2} \\ \frac{1}{3} & \frac{2}{3} & \frac{3}{3} & \frac{4}{3} \\ \frac{1}{4} & \frac{2}{4} & \frac{3}{4} & \frac{4}{4} \\ \end{array} $$There are sixteen combinations (4x4), but gradients are found by the horizontal being divided by the vertical so some of them cancel down to be the same as others (e.g. 1/2=2/4). Taking out the repeated ones gives:
$$ \begin{array} & \frac{1}{1} & \frac{2}{1} & \frac{3}{1} & \frac{4}{1} \\ \frac{1}{2} & & \frac{3}{2} & \\ \frac{1}{3} & \frac{2}{3} & & \frac{4}{3} \\ \frac{1}{4} & & \frac{3}{4} & \\ \end{array} $$There are 11 combinations left, but each one can be positive or negative, so there are actually 22 gradients.
We now have 2 more things to consider. Although 0 cannot be divided by anything, you can divide anything by 0 to give 0 as a gradient on a straight vertical line. This adds 1 more posible gradient. Likewise you can have infinity ($\infty$) as your gradient on a straight line horizontally, so this is the 24th gradient.
Teachers' Resources
Why do this problem?
This problem offers an introduction to gradients within a simple environment that allows students to explore all the important aspects of gradient. This is a suitable preparation for students to move on to:
- Straight line graphs (for example Parallel Lines)
- Gradients of perpendicular lines (for example At Right Angles)
- Exploration of Pythagoras' Theorem (for example Tilted Squares)
Possible approach
These printable resources may be useful: How steep is the slope,
Grid Worksheet.
Share with the class the definition of gradient given at the start of the problem. Resist the temptation to give a 'rule' for working out gradients, as methods should emerge in the discussions that follow.

Use these questions as an opportunity to share different strategies for working out the gradients.
Hand out copies of this sheet and ask students to work in pairs on the main problem:
"How many different gradients can you find? Can you find them all?"
"Arrange them in order of steepness and list the points each line passes through."
Expect students to work systematically so that they are in a good position to justify their findings.
Circulate while students are discussing the problem, and towards the end of the session invite pairs with interesting approaches to share their conclusions with the rest of the group.
Abigail Mantaj from Diocesan School for Girls in Auckland, New Zealand, was inspired by the original problem to create this task for her students.
Key questions
What does it mean for a line to have positive or negative gradient?
A standard method for working out gradients is to divide the vertical displacement by the horizontal displacement. What is the connection between this algorithm and the definition of gradient given at the start of the problem?
Possible support
Students who are struggling to work systematically could be given the prompt, explained in the Hint, to fix one end of their ruler at an extreme point on the grid, and slowly move the other end to create lines with different gradients.
Possible extension
Students could move on to At Right Angles to consider the gradients of perpendicular lines, or on to Parallel Lines and Perpendicular Lines to investigate the relationship between the equations of parallel and perpendicular lines on a co-ordinate grid.
