Equilateral Pair
Weekly Problem 39 - 2016
In the diagram, VWX and XYZ are congruent equilateral triangles. What is the size of angle VWY?
In the diagram, VWX and XYZ are congruent equilateral triangles. What is the size of angle VWY?
Age
11 to 14
Challenge level
Image
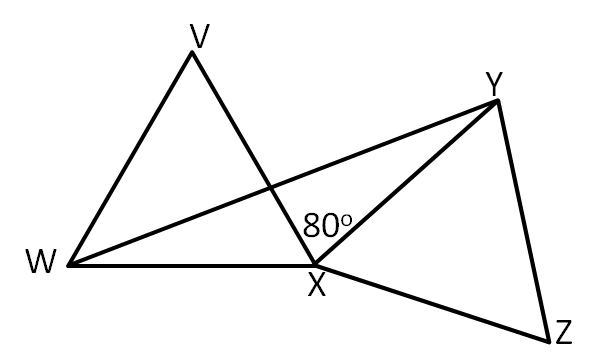
What is the size of $\angle VWY$?
If you liked this problem, here is an NRICH task that challenges you to use similar mathematical ideas.
Since $VXW$ is an equilateral triangle, $\angle VXW = 60^\circ$. Therefore $\angle WXY = \angle WXV + \angle VXY = 60^\circ + 80 ^ \circ = 140^\circ$.
Since the equilateral triangles are congruent, $WX = XY$, so the triangle $WXY$ is isosceles. Therefore, $\angle YWX = \angle XYW = \frac{1}{2}\left(180^\circ - \angle WXY\right) = \frac{1}{2}\left(180^\circ - 140^\circ\right) = 20^\circ$.
Then, since $VXW$ is equilateral, $\angle VWX = 60^\circ$. Then, $\angle VWY = \angle VWX - \angle YWX = 60^\circ - 20^\circ = 40^\circ$.