Using area

The diagram below shows the square with area 72 cm$^2$ with the diagonal in red. Then 4 copies of the square are stuck together. Notice that the diagonals form another square, which is also shown on its own.

 

Image

The square formed by the diagonals occupies half of the area of the 4 blue squares, so it has the same area as 2 blue squares, which is 72 + 72 = 144 cm$^2$.

So a square with side length equal to the red diagonal has area 144 cm$^2$, which means the diagonal must be 12 cm, since 12 $\times$ 12 = 144.

Using Pythagoras' Theorem

The area of the square is $72$ cm$^2$, so theside length is $\sqrt{72}$. This is shown on the diagram below, which also shows the diagonal, marked $d$.

 

Image

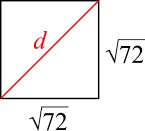
Applying Pythagoras' Theorem gives $$\begin{align}\sqrt{72}^2+\sqrt{72}^2&=d^2\\

\Rightarrow 72+72&=d^2\\

\Rightarrow 144&=d^2\\

\Rightarrow 12&=d\end{align}$$ (since $d$ must be positive).