Christmas chocolates

How could Penny, Tom and Matthew work out how many chocolates there are in different sized boxes?

Age

11 to 14

Challenge level

Exploring and noticing Working systematically Conjecturing and generalising Visualising and representing Reasoning, convincing and proving

Being curious Being resourceful Being resilient Being collaborative

Penny, Tom and Matthew were each given mint chocolates in a hexagonal box:

Penny ate $10$ chocolates and then quickly worked out that there must have been $61$ chocolates at the start.

Tom ate $20$ chocolates and then also managed to work out very quickly that there were originally $61$ chocolates:

Matthew ate $24$ chocolates and could also see very easily that he must have started with $61$ chocolates:

Can you see how each child managed to work out that there were $61$ chocolates in the full box?

You may find these chocolate box templates useful.

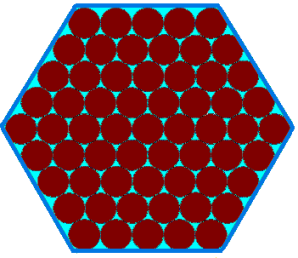
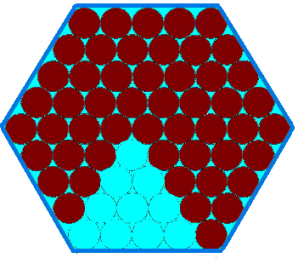
Penny, Tom and Matthew have been promised a larger box of chocolates as a Christmas present from their grandmother. The box will have $10$ chocolates along each edge, instead of just $5$.

How would each child work out how many chocolates the larger box will contain?

Can you describe any other ways to work it out?

Here are some more questions you might like to consider:

For which sizes of chocolate box will the three children be able to share the chocolates equally?
For which sizes of chocolate box will the boys be able to share the chocolates equally?
Can you describe how each child would work out the number of chocolates in a box with $n$ chocolates along each edge?

This problem demonstrates that multiple, different methods can be used to approach a particular question. In addition, you are encouraged to devise a general formula for the number of chocolates in any hexagonal box. In doing this, it then becomes very straightforward to work out the number of chocolates there are, however large the box is.

Working out general formulae is not only fun and satisfying, but is also very useful; it provides a quick way to extend the original set of data, without having to work out every consecutive point. Scientists, Mathematicians, Engineers, Accountants...they all find general formulae very helpful, and make use of them regularly.

In the Christmas Chocolates problem, the formula can be extended to any size of box, because the differences are uniformly spaced. However, sometimes we do not have "perfect" information. For example, an experiment may yield slightly different results each time (this is why we do repeat experiments), but we cannot do an infinite number of trials. Thus we settle on an appropriate number, and then use the data collected to see if there is a pattern. From this, perhaps with more experiments, we may be able to devise a general formula. This can then be used to make predictions, and then experiments can be used to test these. The formula may then be refined, and then new predictions made, and so on. Remember though that the formula may not be true for all conditions, unlike the chocolate problem. For example, the formula may only apply within a given temperature range. Nevertheless, having a formula is very useful; it not only makes calculations more efficient, but can also yield great insight into the properties of the problem.

Lots of great solutions were submitted, so thank you to everyone for this! Many students noticed the shapes that were made once Penny, Tom, and Matthew had eaten some chocolates. They then realised that several of these shapes could fit into the whole hexagonal box. This is useful as it provides a convenient way of grouping the chocolates together, and therefore quickens calculations. The patterns can then be used to extend to other box sizes, as several students did.

Courtney, from Penrhos College, submitted this solution. It is lovely: clear, and illustrated. Lauren, from Tudhoe Grange, also submitted a good solution. This solution is colourful, and demonstrates the different shapes created by Penny, Tom, and Matthew very clearly. Adam, from Wilson's School explained:

Penny worked out that there were $61$ chocolates by using triangles of $10$ chocolates. Penny would have found that she could find six of these triangles. $6\times10=60$, but there is still the chocolate in the middle of the box, so we add this on, which makes $61$.

Tom would have used parallelograms of $20$ chocolates; these parallelograms are "four by five". He could find three of these parallelograms. $3\times20=60$, but again there is still the chocolate in the centre so there would be $61$ chocolates in total.

Matthew ate all the chocolates on the outside, which makes $24$ chocolates. As there are 24 chocolates on the outside it must be $(5-1)\times6$. This is because there are six sides, and if you multiplied six by five you would count six chocolates twice. Each layer, moving inwards, decreases by six. So, to work out how many chocolates there are in the box the sum is:

$[(5-1)\times6]+ [(4-1)\times6]+ [(3-1)\times6]+ [(2-1)\times6]+1$, which equals 61.

Another way to work it out would be to add the rows up.

Luke, from Wilmslow High School, used the alternative method suggested by Adam. In his solution, he added the rows of the hexagon up to find out the total number of chocolates in the box.

Monty, from The Perse School, and Claudia & Martha from Impington Village College noticed that Penny and Tom's methods were extremely similar; Tom ate twice as much as Penny, so the only difference between the caluclation is a factor of two!

Philip, from Wilson's School extended the methods used by Penny, Tom, and Matthew to work out the number of chocolates in a box with ten along each edge:

If the sides of the box measure $10$ chocolates:

Penny would find out that the box would have $271$ chocolates by realising that there were six triangles made up of $45$ chocolates each, plus one extra chocolate in the middle.

Tom would work out that there were $271$ chocolates in the box because he would realise that the box can be divided into three parallelograms, made up of $90$ chocolates each, plus one extra chocolate.

Matthew would work out that there were $271$ chocolates in the box because he would realise that the outer layer of chocolates would be made up of $54$ chocolates and each layer afterwards would have a decrease of $6$ chocolates, plus one extra chocolate in the middle: $54+48+42+36+30+24+18+12+6+1 = 271$

You can begin to see a pattern; the hexagonal box can always be divided into six triangles, or three parallelograms, or can be stripped down layer by layer like Matthew did. This is true whatever size the box is (as long as it has six sides). This important characteristic can then be used as a starting point for developing a general formula for the number of chocolates in the box. Philip from Gosforth High School used the pattern:

If each child was given a chocolate box with $10$ along each side, the number of chocolates in the box would be $271$. This would be because the number along the bottom of the triangle (like Penny created) is always one less than the number along each side, therefore the base of the triangle would be nine chocolates wide. This would also make the number going up the side nine, which in turn would make each triangle have $45$ chocolates in it (the triangle number of nine). As there is always one chocolate which is not inlcuded in any of the triangles, you add this to the sum of $45\times6$ ($6$= the number of these triangles in the hexagon) to get $271$.

Following on from this, several people submitted correct formulae for the number of chocolates in a box with n along each side. These include: Adam, Philip, and Elliot from Wilson's School, Thomas from Perse Upper School, Rajeev from Fair Field Junior School, and Hannah & Phil (they did not submit a school). Hannah and Phil explained how they worked out a formula:

We felt that Penny's method was most effective (for us), so we tried solving for the quantity of chocolates of a hexagonal box, with a side length of 10 chocolates. We tried coming of up with a formula to calculate the number of chocolates, using Penny's method.

So we went back and analysed the equation $(10\times6)+1=61$, and soon realized those numbers represented this: [(Number of chocolates per triangle) x 6] + 1 = total number of chocolates. Then we tried finding a formula for how to achieve the number of chocolates per triangle. Soon we realized that it consisted of rows, each one less than the one underneath:

1 O

2 OO

3 OOO

4 OOOO

5 OOOOO

This reminded us of previous problems we have dealt with based on this principle, such as finding the sum of the numbers from 1 through 10. The formula was: $( ½n)\times(n-1)$.

Then we tested this formula to see if it applied for finding the number of chocolates per triangle: $( ½n)\times(n-1)$

Substitute n=$5$ into the formula: $( ½(5)\times(5-1))= 2.5\times4= 10$.

The formula did work.

So then we were able to put the whole formula together, for the whole box of chocolates, based on Penny's method.

We had: $[( ½n)\times(n-1)\times6]+ 1$. This then simplified to: $[3n(n-1)] +1$.

Now we could solve the problem with a hexagon of side length 10 chocolates:

$[3n (n-1)] +1$

Substitute n=$10$ into the formula:

$[3(10)\times(10-1)] +1= (30\times9) +1= 270 + 1= 271$.

A box of chocolates with side length 10 would have 271 chocolates inside. When analysing this formula, we realized, that, unfortunately, there is no size the chocolate box can be that would allow the three children to share the chocolates among themselves equally. The number of chocolates would have to be divisible by $3$, and the formula shows that the number of chocolates will always be $1$ more than a multiple of $3$.

Kevin, Leif, Oliver, Nisha, Olivia, Thomas, and Henry from Highgate School explained the latter point made by Hannah and Phil (about sharing the chocolates equally). They used Matthew's method as an example:

To find the number of chocolates in one layer (like Matthew did) you multiply the number of chocolates (minus one) on each side by six, which means that this number is a multiple of six. It is therefore also a multiple of three. Every layer surrounding the middle chocolate is also a multiple of six (and therefore of three), so when you share out the chocolates between the three of them the last one (the one in the middle) will be left over.

So there is no size of box which means that the three of them can share the chocolates out equally.

They then applied the same principle to work out the size of chocolate box the boys will be able to share out equally (i.e. between two people):

The number of chocolates in each layer (here we are referring to the layers created by Matthew) is a multiple of six, and so it is also an even number. So, when you add all the layers together and then add the middle chocolate, the total will be an odd number. So there is no size of box the two boys can share out equally.

In fact, as Philip from Wilson's School pointed out:

Whatever the number of chocolates in the box, the children will never be able to share them out equally.

What's the moral of this, then? Well, if you want to share a box of chocolates equally between two or three people, it should not be a hexagonal box like the one in the question. You could, of course, not share it equally... and have more for yourself... Even better, give more to somebody else!

Thank you to everyone who submitted solutions to this tasty chocolatey problem. If you enjoyed this (and we know that you did!), have a go at Summing Squares and Picture Story. These problems are nice extensions of this chocolate problem. If you would like to try a similar, related problem for more practice, try Picturing Triangle Numbers, Mystic Rose, or Handshakes.

Why do this problem?

Many students are accustomed to using number patterns in order to generalise. This problem offers an alternative approach, challenging students to consider multiple ways of looking at the structure of the problem. The powerful insights from these multiple approaches can help us to derive general formulae, and can lead to students' appreciation of the equivalence of different algebraic expressions.

Possible approach

This problem might follow on nicely from Picturing Triangle Numbers, Mystic Rose, and Handshakes.

Display this image of a full size $5$ box of chocolates, and ask students to work out how many chocolates there are, without speaking or writing anything down. Compare solutions and share approaches.

Mention that mathematicians like to find efficient methods which can be used not only for simple cases but also when the numbers involved are very large. Explain that the pictures of Penny's, Tom's and Matthew's partially-eaten chocolates could be used by a mathematician as a starting point for finding an efficient method for counting the total number of chocolates.

Click here for the three pictures.

Hand out these chocolate box templates and ask students to show how the images of the partially-eaten chocolates can be used to calculate the total.

Ask students to report back, explaining the methods which have emerged.

Then ask students to use all three methods, along with any methods they devised for themselves, to work out the number of chocolates in a size $10$ box, and verify that all methods agree.

Challenge students to express each method for finding the number of chocolates in any size of box, perhaps introducing some algebra and the idea of a size $n$ box if appropriate.

Bring the class together to share findings. Compare the different "formulae" which have emerged, and ask students to explain why they are equivalent.

Key questions

How does each image help you to count the total number of chocolates quickly?

Can you demonstrate the equivalence of different algebraic expressions?

Possible extension

The problems Summing Squares and Picture Story lead to formulae for some intriguing sequences through analysis of the structure of the contexts.

Possible support

The problem Seven Squares gives lots of simple contexts where formulae emerge by looking at structure rather than number sequences.

Or search by topic

Number and algebra

Geometry and measure

Probability and statistics

Working mathematically

Advanced mathematics

For younger learners