Brothers and sisters
Can you work out how many brothers and sisters are in the family?
Problem
A boy has the same number of sisters as brothers.
Each of his sisters has only half as many sisters as brothers.
How many brothers and sisters are in the family altogether?
If you liked this problem, here is an NRICH task which challenges you to use similar mathematical ideas.
This problem is adapted from the World Mathematics Championships
Student Solutions
Answer: 4 brothers and 3 sisters
Working it out starting from small numbers
Image
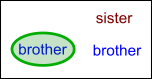
Start from the brother - he must have at least one brother and one sister.
Image

What about the sister? Add another sister and brother so that she has twice as many brothers as sisters.
Image
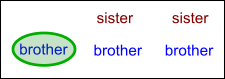
Need to add another brother so that the brother has the same number of brothers and sisters
Image
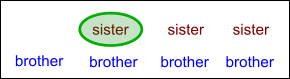
Need to add more so that the sister has twice as many brothers as sisters
Image
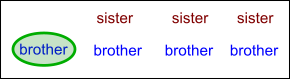
This works for the brother too.
Using algebra
Let $b$ represent the number of brothers in the family and $s$ represent the number of sisters in the family.
Each brother has $b-1$ brothers and $s$ sisters.
Each sister has $b$ brothers and $s-1$ sisters.
The boy has the same number of brothers as sisters, so $b-1=s$.
Each sister has half as many sisters as brothers, so $s-1=\dfrac{1}{2}b$.
Solving by substitution or elimination (see below), $b=4$ and $s=3$, so there are $7$ siblings in total.
Solving by substitution
$s-1=\dfrac{1}{2}b$, so $2s-2=b$ (by multiplying by 2).
Substituting $b=2s-2$ into $b-1=s$ gives $2s-2-1=s$, so $2s-3=s$, so $s=3$.
Substituting $s=3$ into $b-1=s$ gives $b=4$.
Therefore there are $7$ siblings in total.
Solving by elimination
Subtracting $s-1=\dfrac{1}{2}b$ from $s=b-1$ gives $s-(s-1)=b-1-\left(\dfrac{1}{2}b\right)$.
This simplifies to $s-s+1=b-\dfrac{1}{2}b-1$, so $1=\dfrac{1}{2}b-1$.
Adding 1 to both sides, $2=\dfrac{1}{2}b$, and multiplying by 2 gives $4=b$.
Substituting $b=4$ into $b-1=s$ gives $s=3$.
Therefore there are $7$ siblings in total.
