Highest and Lowest
Problem
How about trying with numbers 1, 2, 3, 4, 5 and 6?

Student Solutions
We had just a few submissions to this challenge.
Brodie & Tully from St Patrick's School Macksville NSW in Australia sent in the following;
Highest
(4-3+5)x6=36
Lowest
6/3+4-5=1
(4-3+5)/6
Highest-1, 2, 3, 4, 5, 6
(3+4)/(2-1)x5x6=210
Lowest
(4-3)x2/1+(5-6)=1
M, T and G (Y3) from Monteney Primary School, Sheffield wrote;
M, T and G say that to make the greatest possible total you need to "multiply all the numbers together but not one" Multiplying all of the numbers 2, 3, 4, 5 and 6 together generate a total of 720.
2 x 3 = 6
6 x 4 = 24
24 x 5 = 120
120 x 6 = 720
If you add one to your total you get a total of 721.
720 + 1 = 721
M, T and G said that you add one to make the biggest total, because if you multiplied your total by one then your answer of 720 wouldn't change.
Ashkan from Gorsefield Primary wrote;
The highest I found was:
3 - 4 + (5 x 6) = 29.
I did 5 x 6 which = 30. Then I did 3 - 4 which is -1. Finally I did -1 + 30 = 29.
The lowest I found was:
3 - 4 + (5 / 6) = 0.167
I did 5 / 6 = 0.833. Then I did 3 - 4 = -1. Finally I did -1 + 0.833 = 0.167.
Kestrel class at Churchfields, the Village School in Wiltshire, wrote:
We decided that we had to keep the numbers in order.
At first we could use the operations as many times as we wanted.
Our highest score was 360
3 x 4 x 5 x 6 = 360 (I wonder why you didn't use the 2 as well...)
Our lowest score was -117.
3 – (4 x 5 x 6) = -117
At first we experimented with division before we realised that negative numbers were lower.
Then we decided to see what would happen if we could only use each symbol once (but we kept the numbers in order).
Our highest score was 34.5.
(3 ÷ 4 + 5) x 6 = 34.5
Our lowest score was -51.
3 – ((4 + 5) x 6) = - 51
Thank you, Kestrel class. What a good idea to decide on particular 'rules', like having the numbers in order, or using each operation once.
Thank you for all these different solutions. You may like to think about the ideas in each solution and whether you can improve on them.
Teachers' Resources
Why do this problem?
Possible approach
The children can vary the order themselves either working in pairs or individually. After a short period of independent work ask some of the children to explain their thinking to the others before continuing to see what the highest and lowest possible solutions are.
Having tried this challenge, many children will be able to explore further some of the attributes associated with the four rules of number and place value.
Key questions
How do you know that this is the biggest possible answer?
How do you know that this is the smallest possible answer?
Possible extension
Possible support
Zios and Zepts
Problem
Zios and Zepts printable sheet
On the planet Vuv there are two sorts of creatures. The Zios have 3 legs and the Zepts have 7 legs.

The great planetary explorer Nico, who first discovered the planet, saw a crowd of Zios and Zepts. He managed to see that there was more than one of each kind of creature before they saw him. Suddenly they all rolled over onto their backs and put their legs in the air.
He counted 52 legs. How many Zios and how many Zepts were there?
Do you think there are any different answers?
Getting Started
Drawing some pictures or using sticks/counters for legs might help.
Do you have any idea approximately how many Zios and Zepts there might be to make $52$ legs?
You could try choosing a certain number of Zios and a certain number of Zepts, then count their legs. Do you need more legs or fewer to have a total of $52$?
Student Solutions
Jack from Whangaparaoa School in New Zealand
3 x 1 = 3 52 - 3 = 49 divided by 7 = 7
3 x 8 = 24 52 - 24 = 28 divided by 7 = 4
3 x 15 = 45 52 - 45 = 7 divided by 7 = 1
Shivoham from Robin Hood Junior School sent in the following:

From Hamilton South Public in Australia we had a few submitted solutions:
Henry:
Yes there are different ways to make 52, what I did was I had 7 Zepts and 1 Zios which makes 52 legs. But you can also make 52 legs with 4 x 7 Zepts = 28 legs and 3 x 8 = 24 so 28 + 24 = 52. So this shows there is more than 1 way to answer this question.
Caylum:
52 Legs would make 4 Zepts and 8 Zios
Finn:
In solving this problem of zepts and zois, there can be numerous answers to this problem. Some of the solutions include- 1 lot of zois and 7 lots of zeptsI worked this out by the olution- 52/7= 7 with 3 left over there for on of the solutions is 1 lot of zois and 7 lots of Zepts. Another solution I came up with was- 15 lots of zois and 1 lot of zepts I worked this solution out by swapping the numbers around from solution one- 52/3=15 with 7 left over there for another one of the solutions is 15 lots of zois and 1 lot of zepts. As you can see there are more than one answer I have demonstrated only one though.
Matilda.
I know my 3 times table found that 3 times 15 = 45 and 45 is 7 less that 52.
7 Times 7 is 49 which is 3 less than 52.
Jett :
I added up the legs by doing the Zepts legs and the Zios legs at the same time so
7+3=10 10+3+7=20 20+7=3=30 30+7+3=40 40+3+3+3+3=52
My other way was 7+7+7+7+7+7+7=49, 49+3=52
First answer 8 Zios 4 Zepts.
Second answer 1 Zios 7 Zepts. So there are 2 answers to the Zios and Zepts question.
Advika from Wimbledon Chase Primary School in England
There are 4 Zepts and 8 Zios
At first I tried to do it in ratio but it didn't work.I would write out the timetables of three and seven and then find out numbers that give a sum of fifty-two. Ratio does not work because (seven is to three )-the ratio- are not equal in one portion.My words may be rather. You could also approximate fifty two and use times tables to help you work
the main answer out. I could not find out any corresponding ways to get fifty two and would be grateful to get another answer that doesn't match mine. Below,I have uploaded a file that you may want to consider seeing as it should help you if you get stuck.I hope it is of help to you. Please consider my working out as I could not think of any other way to
come across the answer and would be happy to come across the method if
there is any. I hope this has come to use of you if any use.

Amaan from Christ Church School England wrote:
7x4=28 4 Zepts - 3x8=24 8 Zios
I got this answer by trying to go up systematically in the 7 times table. First of I tried finding out how many Zios there were.Firstly,I took away 14 away from 52 because of the description in the clue. The answer to this sum was 38.From the answer of 38 I tried dividing 38 from 3.But there was no full answer. Next I tried 21,52-21=31. So once again my answer was not in the 3 times table. The next number in the 7 times table was 28. So once again took took my answer 28 away from 52.52-28=24.I then found out 8x3=24.So that was the answer to how many Zios there were and because 28 is the answer to 7x4 that told me the answer to how many Zepts there were.
Anaya from GEMS Willington Academy-AlKhail in the UAE:
As mentioned one zios has 3 legs and one zepts has 7 legs and the scientist found total of 52 legs. We need to find the number of zios and zepts in the planet.
HERE IS THE SOLUTION
1 Zios = 3 legs
8 zios have = 3 x 8 = 24 legs
1 zepts = 7 legs
4 zepts have = 7x4=28 legs
So total no of legs =24+28=52
So the answer is There are 8 NUMBER of zios and 4 NUMBER of zepts in the planet.
Great! You have worked hard on getting solutions and there are some good explanations as to how you went about finding solutions.
Teachers' Resources
Why do this problem?
This problem focuses on numbers that are multiples of 3 and 7 and offers a motivating context in which to develop instant recall of them. It also provides an opportunity for learners to discuss alternative strategies and to consider the merits of each.
Possible approach
Children enjoy considering strange forms of life in imaginary planets and these weird creatures with odd numbers of legs should appeal to them. You could begin by telling the story in more detail and introducing a simpler version of the problem, for example, if Nico saw 6 legs what creatures might he have seen? And how about 14 legs? Or 13 legs? Ask them to work in pairs, perhaps on mini-whiteboards, to think about these first challenges. As they work, observe different ways of recording and thinking about the problem which you can share with the whole group.
At the end of the session learners could share not only their solutions but also their methods. Some may have used a trial and improvement approach, either with the materials provided or using pictures; some may have written lists of multiples of 3 and 7 in a very systematic way and then made totals. Children could consider whether some strategies were more effective than others, although of course a strategy that is effective for one child may be very confusing for another. Having the freedom to approach this problem in any way is key here, but in talking to others, some pupils might change the way they work, which is interesting in itself.
Key questions
Possible extension
Learners could investigate other possible numbers of legs of a group of Zios and Zepts. Perhaps some can be done in more than one way. How many ways can be found for each target number? The investigations can go on and on!
Possible support
Starting with lower totals and using practical equipment to support them will help some children. They may like to write down all the multiples of 3 and 7 as an aid. Some children might benefit from trying the Starfish Spotting problem first which involves just multiples of 2 and 5, and lower totals.
This Pied Piper of Hamelin
Investigate the different numbers of people and rats there could have been if you know how many legs there are altogether!
Problem
"The Pied Piper of Hamelin'' is a story you may have heard or read. This man, who is often dressed in very bright colours, drives the many rats out of town by his pipe playing - and the children follow his tune.
Suppose that there were $100$ children and $100$ rats. Supposing they all have the usual number of legs, there will be $600$ legs in the town belonging to people and rats.
But now, what if you were only told that there were $600$ legs belonging to people and rats but you did not know how many children/rats there were?
The challenge is to investigate how many children/rats there could be if the number of legs was $600$. To start you off, it is not too hard to see that you could have $100$ children and $100$ rats; or you could have had $250$ children and $25$ rats. See what other numbers you can come up with.
Remember that you have to have $600$ legs altogether and rats will have $4$ legs and children will have $2$ legs.
When it's time to have a look at all the results that you have got and see what things you notice you might write something like this:
a) $100$ Children and $100$ Rats - the same number of both,
b) $150$ Children and $75$ Rats - twice as many children as rats,
c) $250$ Children and $25$ Rats - ten times as many children as rats.
This seems as if it could be worth looking at more deeply. I guess there are other things which will "pop up'', to explore.
Then there is the chance to think about the usual question, "I wonder what would happen if ...?''
Getting Started
If you had one fewer rat, what could you replace it with to keep the number of legs the same?
How are you keeping track of what you have done?
Student Solutions
We had a good number of solutions sent in, thank you. Here we will feature those of you who've looked at many possibilities. From George at Linton Heights Junior SchoolӬ we had the following:
a) $0$ rats and $300$ children
b) $1$ rat and $298$ children
c) $5$ rats and $290$ children
d) $10$ rats and $280$ children
e) $25$ rats and $250$ children
f) $50$ rats and $200$ children
g) $100$ rats and $100$ children
h) $125$ rats and $50$ children
i) $150$ rats and $0$ children
From Patrick at Manorcroft Primary School we had this good explanation, well done:Ӭ
There are $148$ different combinations of child and rat because I figured out that you could replace $1$ rat with $2$ children (because one rat has twice as many legs as a child) so the maximum possible children to rats are $298$ children to $1$ rat and the maximum possible rats to children is $149$ rats to $2$ children. If you take $1$ from $149$ to get $148$ possiblities.
Year $5Ӭ$ pupils from St Ambrose Catholic Primary School said;
Ӭ
There are many possible answers to this question, to find out how many children, you can start with the number of rats. You can go from $1$ rat to $149$ rats to work out how many children. The rule is: multiply by $4$, take away from $600$, divide by $2$.
For example, $1$ rat= $298$ children because $1$x$4=4 600-4=596, 596$ divided by $2=298$.
If we start with the number of children the rule is: multiply by $2$, take away from $600$, divide by $4$.
For example, $2$ children $ = 149$ rats because $2$x$2=4$, $600-4= 596, 596$ divided by $4= 149$.
There are some patterns that we noticed, such as: If you take $1$ away from the amount of rats, it adds $2$ to the amount of children. For example: $86$ children, $107$ rats; $106$ rats, $88$ children.
Matthew from Calcot Junior School also discovered this general rule. He said:
There is a formula which is however many rats you have, double that number and take it off 300. Your result is how many children there are.
The total number of legs is 600. Each child has 2 legs, each rat has 4. So the total number of legs can be written as:
Legs = Children x 2 + Rats x 4
That's very helpful, Matthew. By 'children', I think Matthew means the number of children, and 'rats' means the number of rats.
Because we know the number of legs, we can write:
600 = Children x 2 + Rats x 4
Then if we know either children or rats, we can use this to work out the missing one. For example, if there are 100 Children:
600 = 100 x 2 + Rats x 4
600 = 200 + Rats x 4
400 = Rats x 4
Rats = 100
So Rats = (Legs - 2 x Children) $\div$ 4
If you know legs and rats, to work out children:
Children = (Legs - 4xRats) $\div$ 2
This is extremely well explained, thank you Matthew. Matthew calculated all the possible answers, which you can see here. Thank you for sharing this with us, Matthew.
JamesӬ, who calls his school "BG"Ӭ sent in the following good explanation:
When working out the pattern for children/rats, I thought it would be a good idea to start with either everything as children or everything as rats. I decided to start with everything as children, which would be $300$ children and $0$ rats, since $600$ halved is $300$.
Then, I knew if I wanted to get all possible solutions, I'd need to come up with a pattern. My pattern was adding some of the children's legs, to the rats each time. But of course, since rats have twice as many legs as children, that wouldn't work, so I took away two children for every rat I added, as shown in the pattern below:
| Children | Rats | Legs |
| $300$ | $0$ | $600 + 0$ |
| $298$ | $1$ | $596+ 4$ |
| $296$ | $2$ | $592 + 8$ |
| $294$ | $3$ | $588 + 12$ |
I did that on and on, until I was certain, that every time, the legs would total up to $600$. By looking at the number of children, and noticing that it started at $300$, and got $2$ fewer each time, I divided $300$ by $2$, and then added on the $1$ possibilty, with $0$ children, to work out there would be $151$ possible solutions for this.
These solutions are really good. Well done, keep submitting solutions to other activities.
Teachers' Resources
Why do this problem?
Possible approach
Key questions
How many legs do your rats have?
Possible extension
Setting different target numbers of legs offers the chance to explore multiples of 2 and 4 and how they are related. Each target number will have a range of possible solutions. Encourage the children to generalise about how the numbers of rats and people are related.
Another avenue for extension woul be to look at animals with other numbers of legs and perhaps three types of different-legged animals at the same time - eg. birds, spiders and pigs. This option links with Noah.
Possible support
Some children may find the large numbers being considered in the presentation of the problem too high to make sense of so start them off with lower targets such as 20 or 30 legs. Noah is a similar problem involving fewer legs. Some toys or pictures representing the different animals may help some pupils to get started. Modelling clay bodies with straw legs can also be very helpful. Children could be given 20 lengths of straw and work on sharing them between people and rats as a way in to dealing with the larger numbers in a more abstract way.
Four goodness sake
Use 4 four times with simple operations so that you get the answer 12. Can you make 15, 16 and 17 too?
Problem
Write down the number $4$, four times.
Put operation symbols between them so that you have a calculation.
So you might think of writing $4 \times 4 \times 4 - 4 = 60$
BUT use operations so that the answer is $12$
Now, can you redo this so that you get $15$, $16$ and $17$ for your answers?
Need more of a challenge? Try getting answers all the way from $0$ through to $10$.
Student Solutions
Thank you for the large number of interesting solutions that came in. Of course your answers depended upon what you decided was 'allowed'. For the first part, getting an answer of $12$, we had this variety of ideas:
$44+4\div4$
$(4-4/4)$$\times4$
$4+4+√4 + √4$
$4$ x $4 - √4 - √4$
From Year $3$ and $4$ Mathematics Extension Programme at Lumen Christi Catholic Primary School in Australia we had this very good solution:
To work out the solution to the problem we first tried using the four operations ($+, -, \times, \div$). This helped us find some of the solutions.
We then realised we would need to think about the order of operations to get different solutions. So we used our knowledge of brackets and BODMAS to find other solutions.
Finally we thought about other mathematical operators we could use to help us find more solutions.
We worked out that $4^2 = 16$; $4^3 = 64$ and $√4 = 2$ (square root $4$).
This information helped us find the remaining solutions except the answer.
Below are the solutions for the Year $3$ and Year $4$ Mathematics Extension Programs. Each group of students worked separately on the tasks, but have found some similar solutions.
Our goal is now to find all the solutions from $0 - 100$.
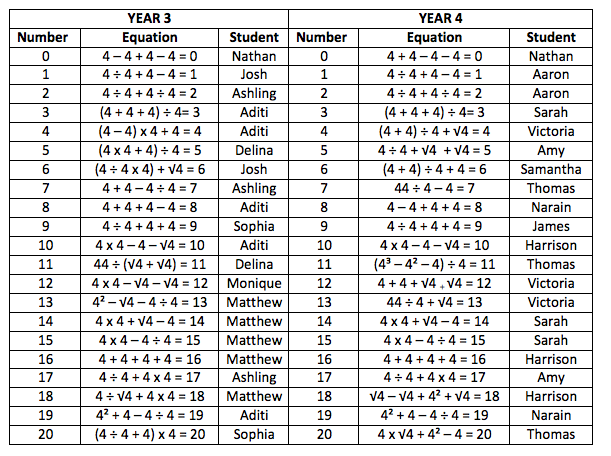
Rosie Goodleigh C of E Primary SchoolӬ sent in the following which was accompanied by similar solutions from Paige, George, Tom, Eva and Jessica.
$0$. First I added $4+4$ which made $8$. Then $-4$ which made $4$ and then$-4$ again which uses four $4$'s and makes $0$
$1$. First I did $4$ divided by $4$ which equals $1$ so then $+4$ and then $-4$ which makes $1$.
$2$. In brackets do $4$ divided by $4$, do this once more, both of them should
equal $1$ each,add both 1s together which equal $2$.
$3$. First do $4+4+4=12$. Subsequently divide $12$ by $4$ which equals $3$.
$4$. First take $4$ away from $4=0$. $0$x$4=0$,then add $4$ which equals $4$.
$5$. First times $4$ by $4 =16$ $16 + 4 =20$. Divide $20$ by $4=5$.
$6$.First add $4$ and $4 =8$. Divide $8$ by $4$ equals $2$. $2 + 4=6$.
$7$. In brackets divide $4$ by $4=1$. Then add $4$ and $4=8$ $- 1 =7$.
$8$. Add $4$ and $4$ x $4=32$. Divide $32$ by $4= 8$.
$9$. Divide $4$ by $4=1$. Add $4$ and $4=8$ $+ 1 = 9$.
$10$. In brackets there is the square root of $4$ which is $2$. Add $4$ and $4$ and $4
=12$ $-2=10$.
$12$. In brackets do $4$ divided by $4=1$. $4 - 1 = 3$. $3$x$4=12$.
$15$. In brackets do $4$ divided by $4 =1$. Do $4$ times $4$ then minus the $1$ to
equal $15$.
$16$. Add $4$ and $4$ and $4$ and $4 =16$.
$17$. In brackets divide $4$ by $4 =1$. Times $4$ by $4$ then add $1 =17$.
Sam from The Lantern Primary School sent in a really good alternative set of answers as follows.
The first ones I did I found quite easy. I used the four simple operations.
$1=(4 \div 4) \times (4 \div 4)$
$12=(44+4) \div 4$
$3=(4+4+4) \div 4$
$6=((4+4) \div 4)+4$
$7=(44 \div 4) −4$
$8=(4 \times 4) −4−4$
$9=(4 \div 4)+4+4$
$15=(4 \times 4) − (4 \div 4)$
$16 = 4+4+4+4$
$17 =(4 \times 4)+(4 \div 4)$
Then I came to a problem. I couldn't do 4, 5 and 10. Then I realised that I had only used the simple operations, addition, subtraction, multiplication and division. Then I remembered about square root, factorial and powers. An example of factorial is:
$4!=4$×$3$×$2$×$1$
The first square root I tried was 4, then I realised I needed a few more. Taking these into account, I was able to do the last few problems.
$4 = \sqrt(4+4+4+4)$
$5 = \sqrt(4!+(4 \div 4)^4)$
$10 = \sqrt(4!+(4 \div 4) \times 4)$
When I checked through my answers, I realised I had forgotten to put brackets in, so I added them in in the appropriate places to make the solutions clearer.
Thank you all for your excellent ideas which I think used so much of your mathematical knowledge and skills.
Teachers' Resources
Why do this problem?
Possible approach
This is one of those problems that is harder than it looks. You could start off by presenting the question just as it is in the text. Alternatively start off by looking at all the different answers that can be gained by using just two $4$s and operation symbols first and building up to three and then four.
The children can see what answers they come up with and challenge one another to match the same solution. Challenging the children to find various numbers as solutions is interesting.
Key questions
How can you get the lowest possible answer?
Tell me about the way you are finding answers.
Can you see what target numbers you can make?
Are there any numbers that you can't make using four fours and various operations?
Possible extension
Challenge the children to make all the numbers to $64$ with four $4$s. What are the gaps? Can we fill any of them by allowing some other operation or an additional four?
The children can also set their own extension challenges.
Possible support
