Birthday cake Candles
This challenge involves calculating the number of candles needed on birthday cakes. It is an opportunity to explore numbers and discover new things.
Age
7 to 11
Challenge level
Problem
Image
Baby Ben is turning one. His parents are planning to celebrate his first birthday with a special cake which will have one candle on it.
They think about future birthdays too, when they will need more and more candles.
They are curious to know how many candles they will need to buy for his birthday cakes, up to and including his eleventh birthday...
They think about future birthdays too, when they will need more and more candles.
They are curious to know how many candles they will need to buy for his birthday cakes, up to and including his eleventh birthday...
If candles last less than a year, how many candles will they need for Ben's cakes, up to and including his eleventh birthday?
How do you know?
What if the candles are able to last for longer than one year?
How many candles will they need for his cakes, up to and including his eleventh birthday now?
How did you work this out?
How do you know?
What if the candles are able to last for longer than one year?
How many candles will they need for his cakes, up to and including his eleventh birthday now?
How did you work this out?
Getting Started
You could use some sort of equipment to represent the candles e.g. lolly sticks, pencils...
Would drawing help?
Would drawing help?
Student Solutions
We had a number of solutions sent in from the Peak School in Hong Kong:
Victoria K and F wrote...
Lasting one year:
1st 2nd 3rd 4th 5th 6th 7th 8th 9th 10th 11th
1c 2c 2c 3c 4c 6c 7c 8c 9c 10c 11c = 66 candles
Since the candles only last one year, Ben's parents will have to buy new candles every year.
Lasting longer than one year:
1st 2nd 3rd 4th 5th 6th 7th 8th 9th 10th 11th
1c 1c 1c 1c 1c 1c 1c 1c 1c 1c 1c = 11 candles
Since the candles last longer than one year, Ben's parents will have to only buy one candle each year, since Ben gets one year older each year.
Similar solutions came from Anna and Alyssa, Zach, Haider, Nicholas, and Ian and Arthur.
We also had several solutions from Winns Primary School in London. Roxy wrote:
Ben's parents need to buy the amount of candles of his age. Every time they need to buy more. I wrote down the amount of candles each year until I got to 11 and then I counted them up and got 66, that is the answer. What if Ben was born on a leap year?
I think the answer is 66 because they have the same amount candles as the age that Ben is turning for 11 years straight. Therefore, you need to add all the numbers from 1 through 11. As it says including his 11th birthday. Now on to the what if question...
From my perspective, I think each candle lasts 2 yrs.
Also from the same school, we had solutions from Reece, Alex, Hester, Ayman, Samson, Jessie, Jathusha, Che and Hierthika.
We also received solutions from Joe, Ayobami, Hamsini, Ava, Zackary, Aoife, Sakina, Gracie, Millie and Niamh at Nene Valley Primary School. Jessica, Finlay and Suzanna sent in this picture:
Image
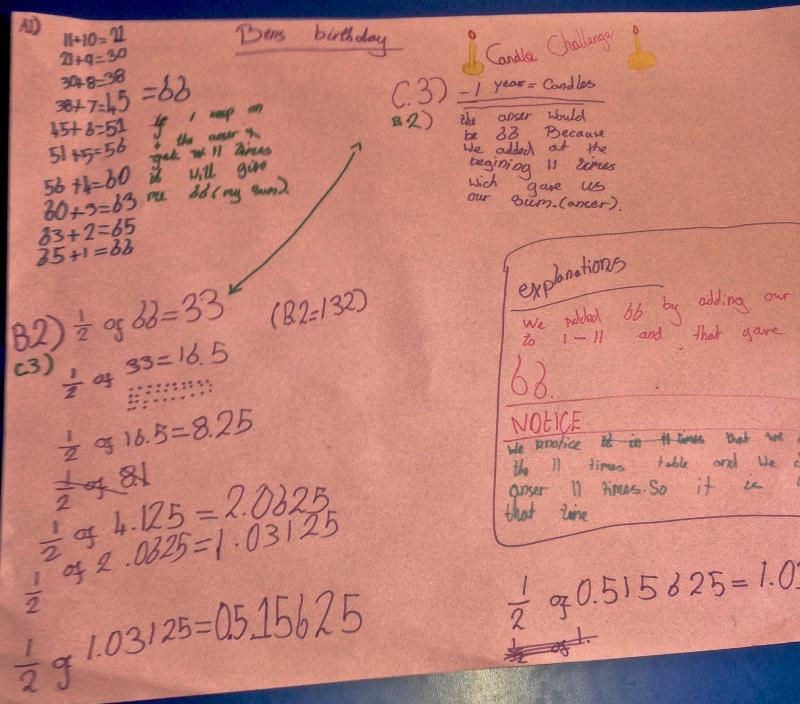
Aarti from the International School Utrecht (ISU) in the Netherlands wrote:
To solve this problem I used my addition skills. I added the numbers, 11, 10, 9, 8, 7, 6, 5, 4, 3, 2, 1, which equals to 66. For baby Ben's every birthday, his parents will need to get a number of candles that is the same number as baby Ben's age. And that is why I used addition to solve this problem.
Thank you, too, to Fraser and Lissa, Aarnavi, Sophi and Antek from Kingsfleet School in Felixstowe who also sent in solutions.
Many of your solutions included further questions for everyone to consider:
Pavithan asks "What if Ben had an older brother?"
Ben asks "What if Ben ate one-and-a-half cakes (6 up) (double candles)?" ,"What if they went until he died(87)?",
Milena asks "What if the candles had to go up to Ben's 16th birthday?","What if the candles lasted 5 years?" , "What if Ben had a twin sister named Benny?"
Tharshagini asks "What if they had more than one child?"
Alex asks "What if Ben had an older brother called Jay and he was 7, surprisingly he was born on the exact same day and the exact same time and they had to buy candles for him too? How many candles would you need?"
Adam asks "What if Baby Ben had a big brother called Charlie who was born a day after, but at the same time and he was 5 years old on Baby Ben's birthday. How many more candles until his 16th birthday would their parents need to buy? Explain why."
Sara asks "What if baby Ben had a brother three years older than him and a sister two years younger than him? How many candles would they need? What if Ben died at 9 years?"
Linna asks "What if baby Ben had a candle with his age on it?"
Aaron asks "What if Ben had a 13-year-old brother who was born on February 29 and all the candles in their house lasted for 1 year? What if Ben was a triplet?"
Blessing asks "What if the candles lasted up to five years? What if they last up to ten years? What if Ben had a twin?"
Ben asks "What if Ben was born on a leap year?
Syd asks "What if Ben is one of a triplet?
Sarah asks "What if he had a twin?"
Thank you for those "What if . . . ?" questions! I wonder whether anyone worked on the solutions to any of them?
We had nearly 80 solutions come in for this task so we apologise for only showing a sample of those here. Well done all of you.
Teachers' Resources
Birthday Cake Candles
Image
Baby Ben is turning one. His parents are planning to celebrate his first birthday with a special cake which will have one candle on it.
They think about future birthdays too, when they will need more and more candles.
They are curious to know how many candles they will need to buy for his birthday cakes, up to and including his eleventh birthday...
They think about future birthdays too, when they will need more and more candles.
They are curious to know how many candles they will need to buy for his birthday cakes, up to and including his eleventh birthday...
If candles last less than a year, how many candles will they need for Ben's cakes, up to and including his eleventh birthday?
How do you know?
What if the candles are able to last for longer than one year?
How many candles will they need for his cakes, up to and including his eleventh birthday now?
How did you work this out?
How do you know?
What if the candles are able to last for longer than one year?
How many candles will they need for his cakes, up to and including his eleventh birthday now?
How did you work this out?
Why do this problem?
This activity uses a familiar non-mathematical situation to spark learners' mathematical curiosity. The fact that they may not immediately know a way of getting to a solution will encourage exploration of the counting numbers and calculation strategies, which in turn provides an opportunity for development of deep understanding.Possible approach
The activity needs little introduction but it can be brought to life with cake (or a model of one!) and some candles to enact the 'story'. It may be helpful to talk through three birthdays, adding candles appropriately, before setting children off on the challenges.As they work on the first part of the task, look out for different ways that learners are recording what they are doing. You could use a mini-plenary to share some of these, which will support those children who might be struggling to get going.
In the second part of the task, learners will have to make assumptions about how long a candle lasts. Again, a mini-plenary could be useful to share some of their thoughts. Make it clear that there will be many answers to this part of the problem, depending on how long they think a candle will last, so it is important for children to communicate their assumptions.
(The 'Further note' section at the bottom of this page offers further support in nurturing curiosity in the classroom more generally.)
Key questions
Can you tell me how you are working this out?Tell me about your answers.
Possible extension
Pupils who have explored binary numbers (which use just zeros and ones) as well as the usual decimal numbers, could explore the use of candles like those shown below and explore how to make the numbers from one to eleven.Image
Over time the shape of the zeros would change but what if the candles could be used just twice, how many ones and how many zeros would you need altogether?
Possible support
Encourage learners to use practical equipment to represent the candles. (Try not to pre-empt who might benefit from the equipment, wait for learners themselves to request it.)Further note
You may be interested in the following talks given by Professor Susan Engels, which focus on encouraging curiosity and are available on YouTube:The Rise and Fall of Curiosity - the extract from 23.50 to 37.15 on adult encouragement and teacher behaviour is particularly worth viewing
The Hungry Mind: The Origins of Curiosity - the extract from 8.22 to 12.29 on children asking questions is especially useful.