Robotic rotations
Problem
Here is an interactivity that allows you to create rotation patterns.
Move the blue dots to create a shape and use the slider to choose an angle of rotation.
Once you've explored the interactivity, take a look at the patterns below and see if you can recreate them. If you like, you can print out the patterns.




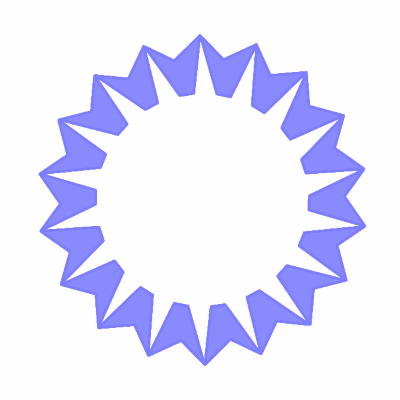



How did you work out which angle to rotate by each time?

To make this picture, Charlie drew a kite and then told the robot to rotate it through an angle of $144^{\circ}$.
Alison used an angle of $216^{\circ}$ and got exactly the same pattern!
What other angles could they have used?
Can the other patterns be made using more than one angle?
What is the link between the angle and the number of copies of the image in the pattern?
Getting Started

It might be a good idea to start by working out the rotational symmetry of each image...
For example, this picture has rotational symmetry of order 4. That means there are four copies in a full $360^{\circ}$ turn. So each rotation must be...
Student Solutions
Ashlynn from ISF Academy in Hong Kong created all of the patterns:

For this picture, Ashylnn described which angles could have been used:
The smallest angle is 72$^\circ$
Multiples of 72$^\circ$ can also generate the same pattern
Yes. In this picture, a rotation angle of 20$^\circ$ generates 18 shapes.
Other angles that are multiples of 20 (but not multiples of 40, 60) can generate the same pattern. These are 100$^\circ$, 140$^\circ$, 220$^\circ$, 260$^\circ$
Notice that this works because 18$\times$20$^\circ$ = 360$^\circ$, which is a full circle. So after 18 rotations of 20$^\circ$ (so 18 shapes), we get back to where we started from.
To make fewer copies of the shape, we need an angle with a multiple of 360 earlier in its times table. For example, above, 5$\times$72$^\circ$=360$^\circ$, so 72$^\circ$ only generates 5 copies of the shape.
5$\times$144 = 720 = 2$\times$360, so again after 5 copies rotating through 144$^\circ$, you get back to where you started. That is why multiples of 72$^\circ$ work.
However, Ashlynn pointed out that some multiples of 20$^\circ$ generate 18 copies of the shape, but those which are multiples of 40$^\circ$ do not.
This is because, although 18$\times$40$^\circ$ = 720$^\circ$ which is 2 full circles, 9$\times$40$^\circ$ = 360$^\circ$. So after just 9 copies, the robot gets back to where it started from and begins repeating the same shapes on top of each other.
Teachers' Resources
Why do this problem?
This problem appears at first to be about angles and rotations, but as students explore more deeply, they may be surprised to discover links to factors, multiples and primes. The images and interactivity provide an enticing hook to stimulate students' curiosity.
Possible approach
Begin by showing students this image (PowerPoint Slide) 
"Have a look at this image. What do you notice?"
Give students a short time to look at the image in silence, and then invite them to discuss with their neighbour.
"How do you think the image might have been created?"
Again, give students some thinking time and discussion time, and then share ideas as a class.
If computers are available, this would be a good time to introduce the interactivity.
"The image was created using this Rotation Robot. Work with a partner to explore the interactivity, and see if you can recreate the image."
As students make a start on the challenge, hand out this worksheet with eight more patterns for them to recreate.
While students are working, circulate and listen for pairs who are developing strategies or who have particularly useful insights. Once most students have successfully recreated most of the patterns, bring the class together and invite pairs to share their strategies and insights. Then draw students' attention to the last part of the task:
"I wonder if we can find a link between the angle and the number of copies of the image in the pattern... In a while, I am going to create a shape and choose an angle. I want you to be able to predict how many copies of the shape will appear."
Give students some more time to explore this using the interactivity. Then in the final plenary, you could challenge them with a few angles and ask them to predict and explain how many copies there will be:
"$160^{\circ}$?"
"There will be nine copies, because the highest common factor of 160 and 360 is 40, and 40 goes into 360 nine times."
"$305^{\circ}?$
"There will be 72 copies because the HCF of 305 and 360 is 5, and 5 goes into 360 72 times."
Key questions
How did you work out which angle to rotate by?
Which patterns can be created using more than one angle?
Are there "families" of angles that make a particular rotational symmetry?
How can you categorise the families?
What is the link between the angle and the number of copies?
Possible support
You may wish to try Attractive Rotations with your students before working on this problem.
Possible extension
Challenge students to explain why the number of copies is related to the highest common factor of the angle of rotation and $360^{\circ}$. Stars would be a good problem to investigate alongside this.
