Difference Sequence
When will 2000 appear in this sequence?
Age
14 to 16
Challenge level
Problem
The first term in a sequence is 2016 and the second term is 2017.
Every other term is given by the difference between the two terms before it. So for example, the third term is the difference between 2016 and 2017, which is 1.
The $n^\text{th}$ term is equal to 2000; what is the value of $n$?
This problem is taken from the World Mathematics Championships
Student Solutions
Answer: the 28th term
Image
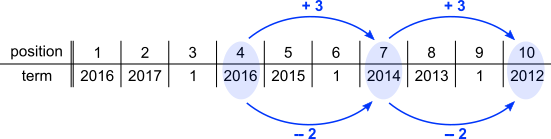
Need to $-$2 six more times to get to 2000
So need to +3 six more times to get the position
10 +3$\times$6 = 28