Pythagoras' dream

The triangle is isosceles, and the hypotenuse is the longest side, so the other two sides must be the same length.

Using a diagram

Image

Because the triangle is isosceles, the two angles that are not right angles must be equal - so they must each be 45$^\text{o}.$

This means that lots of copies of the triangle will fit into the squares:

Image

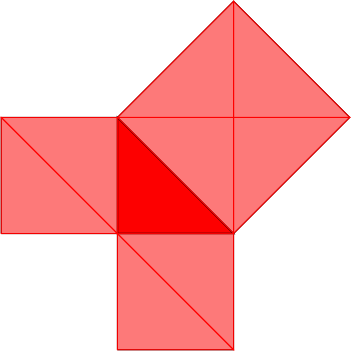
So 8 copies of the triangle have total area 72 cm$^2,$ which means that the area of the triangle is 72 cm$^2\div$8 = 9 cm$^2.$ 

Using Pythagoras' Theorem

Since the two shorter sides are equal, the sides can be labelled as $a,$ $a$ and $c:$

Image

The areas of the squares on the sides of the triangles add up to $72$ cm$^2,$ so, working in cm, $a^2+a^2+c^2=72\Rightarrow 2a^2+c^2=72$

Applying Pythagoras' Theorem, $a^2+a^2=c^2\Rightarrow 2a^2=c^2.$ Substituting this into the first equation gives $c^2+c^2=72\Rightarrow 2c^2=72\Rightarrow c^2=36$ (so $c=6$).

So $2a^2=c^2$ becomes $2a^2=36\Rightarrow a^2=18$

Finding $a$

$a^2=18\Rightarrow a=\sqrt{18}$. The area of the triangle is given by $\frac{1}{2}a\times a=\frac{1}{2}a^2$.

$\frac{1}{2}(\sqrt{18})^2=\frac{1}{2}18=9,$ so the area of the triangle is $9$ cm$^2.$

Finding the area directly

The area of the triangle is given by $\frac{1}{2}a\times a=\frac{1}{2}a^2$.

$a^2=18\Rightarrow \frac{1}{2}a^2=9,$ so the area of the triangle is $9$ cm$^2.$