Question of Three Sides

Using Pythagoras' Theorem on two parts of the triangle

The area of a triangle is $\frac{1}{2}$base$\times$height, so taking the base of the triangle as the side of length $22,$ drawing on the height splits the triangle into two right-angled triangles:

Image

  

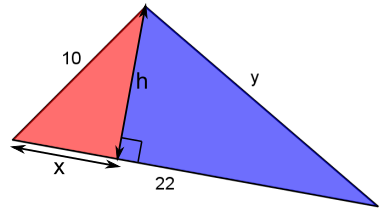
$\frac{1}{2}\times22\times h=88$

$\Rightarrow 11\times h=88$

$\Rightarrow h=8$

The base of the red triangle can be found using Pythagoras' theorem, and then we will know the base of the blue triangle (since they add up to $22$), so we can use Pythagoras' Theorem again to find $y.$

$x^2+8^2=10^2$

$\Rightarrow x^2 =10^2-8^2 = 36$

so $x=6$

So the base of the blue triangle must be $16.$

So $8^2+16^2=y^2$

$\Rightarrow 320=y^2$

$\Rightarrow y=\sqrt{320},$

which is equal to $8\sqrt{5}$ or $17.89$ (2 d.p.).

Using trigonometry

In the diagram below, the triangle has been labelled to make substitution into the trigonometric formulae easier.

Image

 

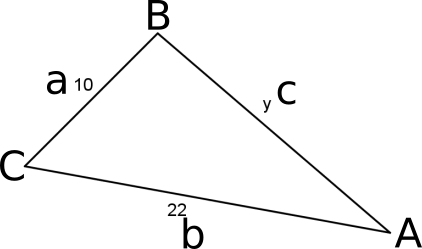
Using the formula for the area of a triangle $\frac{1}{2}ab\sin{C}$, $\frac{1}{2}\times10\times22\times\sin{C}=88\Rightarrow 110\sin{C}=88\Rightarrow\sin{C}=0.8,$ so $C=53.13^\text{o}.$

Then using the cosine rule $c^2=a^2+b^2-2ab\cos{C},$ $$\begin{align}&y^2=10^2+22^2-2\times10\times22\times\cos{53.13^\text{o}}\\

\Rightarrow&y^2=100+484-440\times0.6\\

\Rightarrow&y^2=320\\

\Rightarrow&y=\sqrt{320}=8\sqrt{5}\approx17.89\end{align}$$