Winding vine
A vine is growing up a pole. Can you find its length?
Problem
A pole is 28 metres high and has a constant circumference of 3 metres.
A vine has grown from the bottom to the top of the pole, winding around the pole 7 times.
How long is the vine?
This problem is adapted from the World Mathematics Championships
Student Solutions
Unwrapping the vine from the pole
Imagine unwrapping the vine from the pole, keeping the top of the vine at the same height as the top of the pole, as shown.

Then when the vine is fully unwound, it will form the hypotenuse of a right-angled triangle.
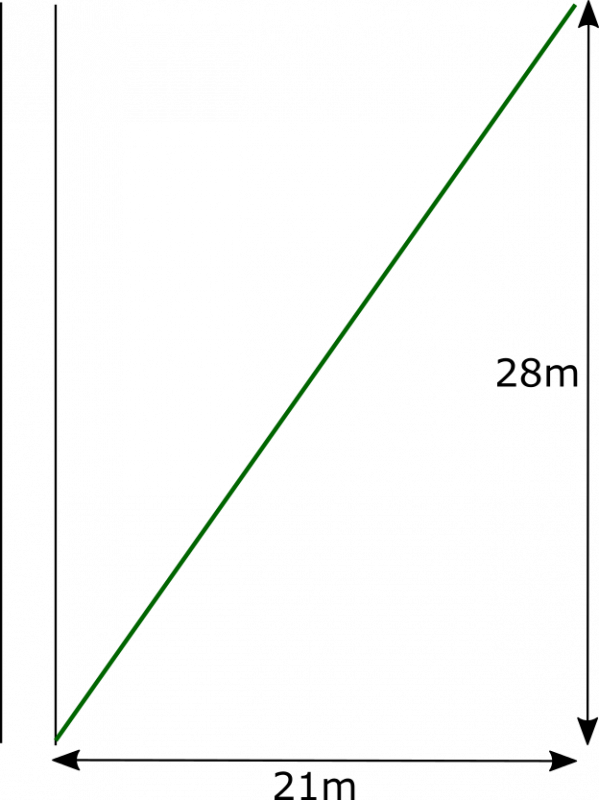
The height of the triangle is the same as the height of the pole, 28 metres. The base of the triangle is 7 times the circumference of the pole, 7$\times$3 = 21 metres.
Applying Pythagoras' Theorem, the length of the vine is $\sqrt{28^2+21^2}=35$ metres.
Unwrapping the surface of the pole
Imagine unwrapping the surface of the pole, as shown below, to give a 3 metre wide strip with seven right-angled triangles on it. The height of each triangle will be 28$\div$7 = 4 metres.
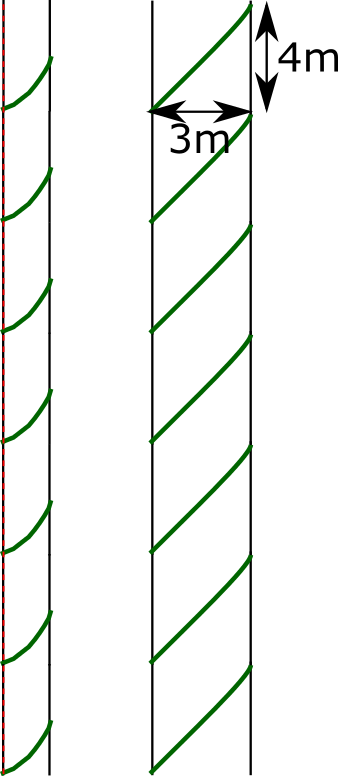
Applying Pythagoras' Theorem, the length of each section of the vine is $\sqrt{3^2+4^2}=5$ metres.
So the total length of the vine is 7$\times$5 = 35 metres.
Imagine unwrapping the vine from the pole, keeping the top of the vine at the same height as the top of the pole, as shown.
Image

Then when the vine is fully unwound, it will form the hypotenuse of a right-angled triangle.
Image

The height of the triangle is the same as the height of the pole, 28 metres. The base of the triangle is 7 times the circumference of the pole, 7$\times$3 = 21 metres.
Applying Pythagoras' Theorem, the length of the vine is $\sqrt{28^2+21^2}=35$ metres.
Unwrapping the surface of the pole
Imagine unwrapping the surface of the pole, as shown below, to give a 3 metre wide strip with seven right-angled triangles on it. The height of each triangle will be 28$\div$7 = 4 metres.
Image

Applying Pythagoras' Theorem, the length of each section of the vine is $\sqrt{3^2+4^2}=5$ metres.
So the total length of the vine is 7$\times$5 = 35 metres.
