Pentagon Construction
Follow the instructions to create a regular pentagon. Can you prove it is indeed regular?
Problem
Here are a set of instructions for a construction. You could use pencil, ruler and compasses and draw it on plain paper, but you might like to use dynamic geometry software such as GeoGebra, which is free to download and use. If you have never used GeoGebra before, you might like to read this article first.
It may help to know the following identities:
\begin{eqnarray} \cos 72^\circ &=& \frac{\sqrt{5}-1}4\\
\cos 144^\circ &=& \frac{-1-\sqrt{5}}4
\end{eqnarray}
- Draw a circle with centre $A$ passing through a point $B$.
- Draw the line through $A$ and $B$. Mark the point where this intersects the circle again as $C$
- Draw in the line perpendicular to $AB$ passing through the point $A$. Mark the intersections with the circle as $D$ and $E$.
- Find the midpoint of $AB$ and mark it as $F$.
- Draw the circle with centre $F$ that passes through $D$. Mark the intersections with the line passing through $A$ and $B$ as $G$ and $H$.
- Draw the circle with centre $D$ that passes through $G$. Mark the intersections with the original circle as $I$ and $J$.
- Draw the circle with centre $D$ that passes through $H$. Mark the intersections with the original circle as $K$ and $L$.
- Join the vertices $D$, $I$, $K$, $L$ and $J$.
Can you prove that this construction forms a regular pentagon?
It may help to know the following identities:
\begin{eqnarray} \cos 72^\circ &=& \frac{\sqrt{5}-1}4\\
\cos 144^\circ &=& \frac{-1-\sqrt{5}}4
\end{eqnarray}
Getting Started
To show that the construction yields a regular pentagon, you might find it useful to use cartesian coordinates. Take A to be (0,0) and B to be (1,0). Can you work out the coordinates of the other points?
Can you use Pythagoras to work out any of the lengths in your diagram?
How might the identities for $\cos 72^{\circ}$ and $\cos 144^{\circ}$ help?
Can you use Pythagoras to work out any of the lengths in your diagram?
How might the identities for $\cos 72^{\circ}$ and $\cos 144^{\circ}$ help?
Student Solutions
Vignesh and Amrit from Hymers College in the UK sent in proofs which use the same reasoning.
This is Vignesh's diagram, with the names of the points added.
Image
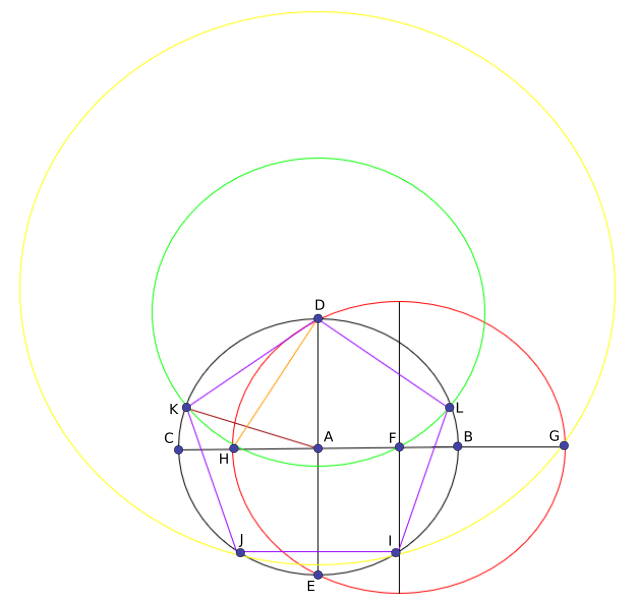
This is the beginning of Amrit's proof:
Let the radius of the circle $C_1$ (black in Vignesh's diagram) with centre at point $\text A$ passing through point $\text B$ be $1.$
Vignesh made a different choice:
$R_0 = \text{AB}$ (radius of black circle)
Let $R_0 = x$
Vignesh calls the distance $\text{AB}$ $x$, because it is unknown, but Amrit says it is $1.$ Changing the length $\text{AB}$ will rescale the diagram, but it won't affect whether the pentagon is regular, so it doesn't matter how long $\text{AB}$ is. This is why it is safe for Amrit to choose that $\text{AB}=1.$ Vignesh also chose this length at the beginning of the constuction, because it starts with two points $\text{A}$ and $\text{B}$ - and Vignesh had to choose how far apart to put them.
Using $1$ instead of $x$ makes the proof simpler, so here is the rest of Amrit's proof:
Image
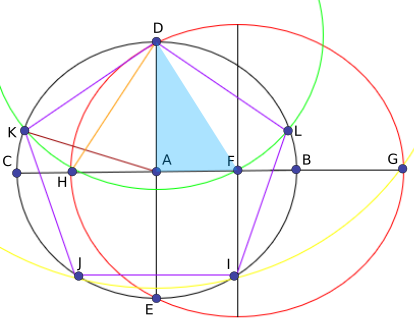
$\text{DF}^2=\text{FA}^2+\text{DA}^2$
Since point F is the midpoint of the radius $\text{AB},$ $\text{FA}=\frac{1}{2}.$ Since $\text{DA}$ is a radius, $\text{DA}=1.$ Substituting these values into the above equation gives$\text{DF}=\dfrac{\sqrt5}{2}$
Then, I saw the right angled triangle $\text{ADG}$. By Pythagoras' theorem,
Image
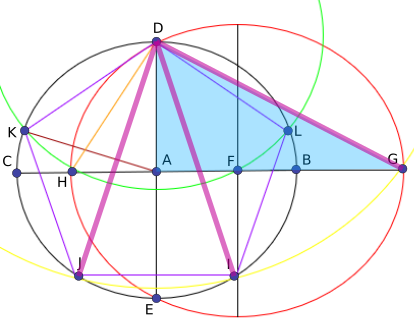
$\text{DG}^2=\text{DA}^2+\text{AG}^2$
But $\text{AG}=\text{GF}+\text{FA}$And, since $\text{DF}$ and $\text{GF}$ are both radii of the circle with centre $\text F$ passing through the point $\text D$ (red), $\text{GF}=\text{DF}=\frac{\sqrt5}{2},$ so $\text{AG}=\frac{\sqrt5}{2}+\frac{1}{2}=\frac{\sqrt5+1}{2}.$ $\text{DA}$ is again equal to $1.$ Substituting these values into the above equation, we obtain
$\text{DG}=\dfrac{\sqrt{10+2\sqrt5}}{2}$
The next right angled triangle I observed was triangle $\text{AHD.}$ By Pythagoras' theorem,
$\text{DH}^2=\text{AH}^2+\text{DA}^2$
Image
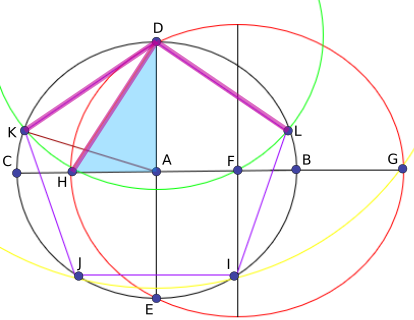
And $\text{HF}=\text{DF}=\frac{\sqrt5}{2}$, since they are both radii of the circle with centre $\text F$ that passes through $\text D$ (red). So $\text{AH}=\frac{\sqrt5}{2}-\frac{1}{2}=\frac{\sqrt5-1\ }{2}.$ $\text{DA}$ is again equal to $1.$ Substituting these values into the above equation, we obtain
$\text{DH}=\dfrac{\sqrt{10-2\sqrt5}}{2}$
$\text{DL = DK = DH,}$ since these line segments are all radii of the circle with centre $\text D$ passing through $\text H$ (green).Image

$\angle \text{ DAL}=\arccos{\left(\dfrac{LA^2+DA^2-DL^2}{2\left(LA\right)\left(DA\right)}\right)}$
$\text{LA = DA}=1,$ since these line segments are both radii of the circle $C_1$ (black), and it was deduced above that $\text{DL = DH}=\dfrac{\sqrt{10-2\sqrt5}}{2}.$ Substituting these values into the above equation, we obtain$\angle \text{ DAL}=\arccos{\left(\dfrac{\sqrt5-1}{4}\right)}=72^\circ$
Now, triangles $\text{LAD}$ and $\text{DAK}$ are congruent. This is because $\text{LA = KA}$ (radii of [black] circle), $\text{AD = DA}$ (common side of both triangles) and $\text{DL = DK}$ (deduced above), so the triangles are congruent by the SSS theorem. In particular, this means that$\angle\text{ DAK}=\angle\text{ DAL}=72^\circ$
Image
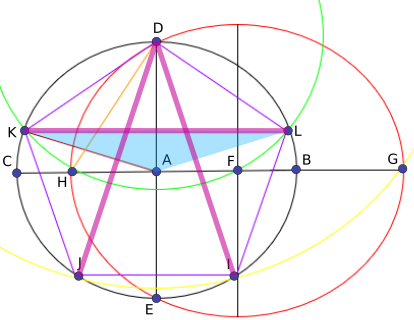
$\angle\text{ LAK}=\angle\text{ DAL}+\angle\text{ DAK}=144^\circ$
From the cosine rule, we can find the length of $\text{LK}:$
$\text{LK}=\sqrt{\text{LA}^2+\text{KA}^2-2\left(\text{LA}\right)\left(\text{KA}\right)\cos{\left(\angle \text{ LAK}\right)}}$
$\text{LA = KA}=1,$ since they are the radii of the circle $C_1$ (black), and we know that $\cos{\angle\text {LAK}}=\cos{144^\circ}=\frac{-1-\sqrt5}4.$ Substituting these values into the above equation, we obtain
$\text{LK}=\dfrac{\sqrt{10+2\sqrt5}}{2}$
So $\text{LK = DI = DJ.}$ We see then that triangle $\text{ALK}$ is congruent to triangles $\text{DAI}$ and $\text{DAJ}$ by the SSS theorem, since one side of one triangle is equal to another side of the other two triangles ($\text{LK = DI = DJ}$), and the other two sides of one triangle are equal to the other two sides of the other two triangles, because they are all radii of the circle $C_1$ (black).
In particular then, $\angle\text{ DAJ}=\angle\text{ DAI}=144^\circ.$ Also,
$\angle\text{ JAI}=360^\circ-\left(\angle\text{ DAJ}+\angle\text{ DAI}\right)$
So $\angle\text{ JAI}=72^\circ.$
Now, $\angle\text{ LAI}=\angle\text{ DAI}-\angle\text{ DAL}=72^\circ.$ Therefore, $\angle \text { KAJ}=360^\circ-\left(\angle \text{ DAK}+\angle \text{ DAL}+\angle \text{ LAI}+\angle \text{ JAI}\right)=72^\circ.$
Therefore, by the SAS congruence axiom, the triangles $\text{AIJ, AJK, AKD, ADL, ALI}$ are all congruent to each other and, in particular, $\text{IJ = JK = KD = DL = LI}$ and all the angles in one of the above triangles are equal to the angles in the corresponding four triangles, therefore $\angle\text{ I}=\angle\text{ J}=\angle\text{ K}=\angle\text{ D}=\angle\text{ L},$ where these angles are the interior angles of the shape $\text{IJKDL,}$ making this shape a regular pentagon.
It is also possible to prove that the construction gives a pentagon using coordinate geometry:
Using Cartesian coordinates with $A$ as the origin and $B$ at $(1,0)$.
Then, $D$ is at $(0,1)$, since it is the same distance away from $A$ as $B$ is but on a perpendicular line. $F$ is the midpoint of $AB$, so is at $(\frac 12,0)$.
Pythagoras' Theorem tells us that the distance $DF = \sqrt{1^2 + (\frac 12)^2} = \frac{\sqrt{5}}{2}$. Therefore $G$ is at $(\frac{1+\sqrt 5}2,0)$ and $H$ is at $(\frac{1-\sqrt 5}2,0)$.
Then, $DG = \sqrt{1^2 + \left( \frac{1+\sqrt 5}2 \right)^2} = \sqrt{1+\frac{6+2\sqrt 5}{4}} = \frac{\sqrt{10+2\sqrt{5}}}{2}$.
Then, looking at the equations of the two circles (black and yellow), we have:
\begin{eqnarray}
x^2 + y^2 &=& 1\\
x^2 + (y-1)^2 &=& \frac{5+\sqrt 5}2 \\
\end{eqnarray}
Subtracting one from the other yields:$$\begin{eqnarray}
y^2-(y-1)^2&=&1-\frac{5+\sqrt 5}{2}\\
\Rightarrow y^2-\left(y^2-2y+1\right) &=&1-\frac{5+\sqrt 5}{2}\\
\Rightarrow 2y-1 &=& 1-\frac{5+\sqrt 5}{2}\end{eqnarray}$$This has solution:$$y = -\frac{1+\sqrt 5}{2} = \cos 144^\circ$$
So $x =\pm \sin 144^\circ$, since $\left( \pm\sin 144^\circ \right) ^2 + \cos ^2 144^\circ = 1$
To use right-angled triangles, use the sine and cosine graphs to see how $\sin 144^\circ$ and $\cos 144^\circ$ compare to angles less than $90^\circ$:
Image
Image
$\sin 144^\circ = \sin (90^\circ-(144^\circ-90^\circ)) = \sin 36^\circ,\cos 144^\circ = -\cos(180^\circ-144^\circ)=-\cos36^\circ$
So the points $I$ and $J$ can be plotted as shown:
Image
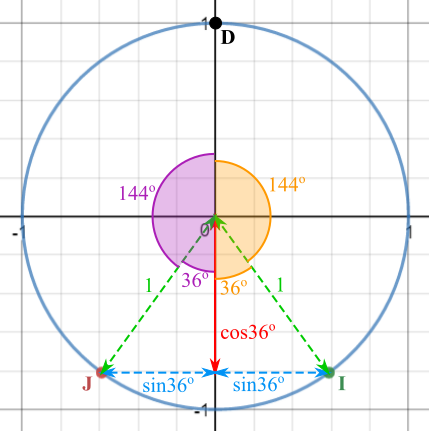
Meanwhile, $DH = \sqrt{1^2 + \left( \frac{1-\sqrt 5}2 \right)^2} = \sqrt{1+\frac{6-2\sqrt 5}{4}} = \frac{\sqrt{10-2\sqrt{5}}}{2}$.
Then, looking at the equations of the two circles, we have:
\begin{eqnarray}
x^2 + y^2 &=& 1\\
x^2 + (y-1)^2 &=& \frac{5-\sqrt 5}2 \\
\end{eqnarray}
Subtracting one from the other yields:$$2y-1 = 1-\frac{5-\sqrt 5}{2}$$This has solution:$$y = \frac{\sqrt 5-1}{2} = \cos 72^\circ$$
So $x =\pm \sin 72^\circ$, since $\left( \pm\sin 72^\circ \right) ^2 + \cos ^2 72^\circ = 1$
So the points $K$ and $L$ can be plotted as shown:
Image
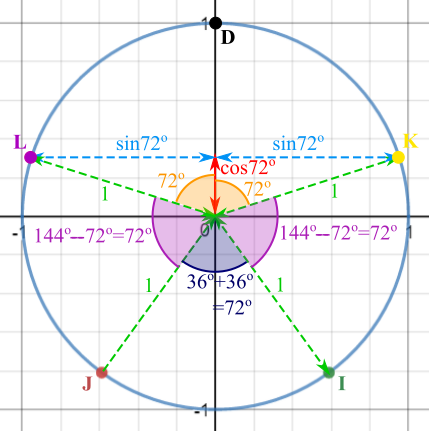
The shape $DLJIK$ is made up of five congruent isosceles triangles, so it is a regular pentagon.
Teachers' Resources
Why do this problem?
This problem provides an excellent opportunity for students to practise using dynamic geometry tools such as GeoGebra as well as introducing an interesting construction they may not have seen before. The proof that the construction yields a regular pentagon is quite challenging and will require students to persevere through a tricky blend of coordinate geometry and trigonometry.
Possible approach
This problem works best if students have access to their own devices with GeoGebra. For students who are complete novices, the article Using GeoGebra would provide useful pre-session reading. Once they have followed each step to complete their pentagon, they may find it useful to print it out in order to annotate it with what they know and what they can deduce. The goal is to calculate the relevant angles based on lengths that can be deduced using coordinate geometry.
Key questions
If it is a regular pentagon, which angles need to be $72^{\circ}$ or $144^{\circ}$?How do the identities $\cos 72^\circ = \frac{\sqrt{5}-1}4$ and
$\cos 144^\circ = \frac{-1-\sqrt{5}}4$ help?
