Inscribed Semicircle
Weekly Problem 43 - 2017
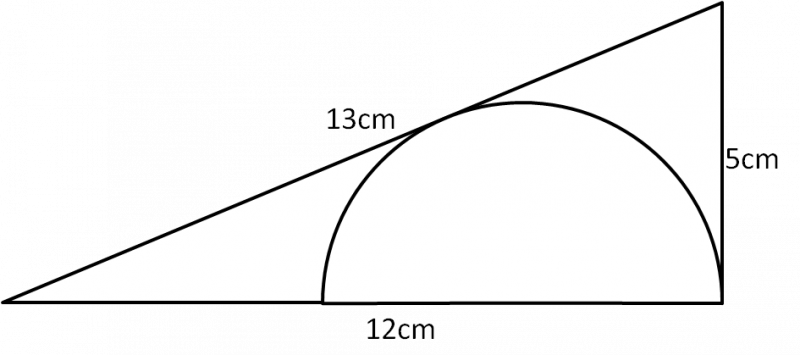
The diagram shows a semicircle inscribed in a right angled triangle. What is the radius of the semicircle?
The diagram shows a semicircle inscribed in a right angled triangle. What is the radius of the semicircle?
Problem
The diagram below shows a right angled triangle with sides of length $5\text{cm}$, $12\text{cm}$ and $13\text{cm}$. What is the radius of the inscribed semicircle whose base lies on the side of length $12\text{cm}$?
Image
If you liked this problem, here is an NRICH task that challenges you to use similar mathematical ideas.
Student Solutions
There are a number of different possible ways of solving this problem, which are presented below.
Solution 1
Image
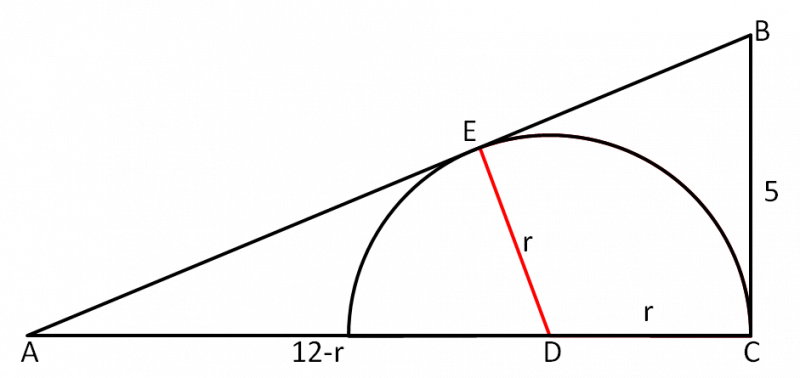
Since the semicircle touches the line $AB$, it is tangent there, so $\angle DEA = 90^\circ$. But then $\angle EAD = \angle CAB$, as these are the same angle. This means the triangles $ADE$ and $ABC$ are similar.
If the radius of the semicircle is $r$, then $DE = DC = r$ and $AD = 12-r$. Then, similarity says that:
$\frac{AB}{BC} = \frac{AD}{DE}$
Therefore:
$\frac{13}{5}=\frac{12-r}{r}$
Clearing the denominators gives:
$13r = 5(12-r)$
Expanding the brackets and collecting like terms gives:
$18r = 60$
Therefore, $r = 3\frac{1}{3} \text{cm}$.
Solution 2
Again, write $r$ for the radius of the semicircle.
Image

As in solution 1, we can prove that $ADE$ is similar to $ABC$. Since the semicircle touches the line $AB$, it is tangent there, so $\angle DEA = 90^\circ$. But then $\angle EAD = \angle CAB$, as these are the same angle. This means the triangles $ADE$ and $ABC$ are similar.
Then, since $\angle DCB$ is a right angle, also, $CB$ and $EB$ are both tangents of the semicircle. Since they intersect, $EB = CB = 5$, so $AE = 13-5=8$.
Then, similarity says:
$\frac{DE}{AE}=\frac{BC}{AC}$
Therefore:
$\frac{r}{8}=\frac{5}{12}$
Multiplying by $8$ gives:
$r = \frac{40}{12} = 3\frac{1}{3}$
This means the semicircle has radius $3\frac 13 \text{cm}$.
Solution 3
This approach uses Pythagoras' theorem, rather than similarity, to solve the problem. Write $r$ for the radius of the semicircle.
Image

Since $AB$ is tangent to the semicircle at $E$, $\angle AED$ is a right angle.
Also, as $BC$ is tangent to the semicircle at $C$, as $\angle ACD$ is a right angle, the lengths $BC$ and $BE$ are the same length, so both are $5\text{cm}$.
This then means that $AE = 8$, $DE = r$ and $AD = 12-r$.
Now, Pythagoras' theorem says that, since $\angle AED$ is a right angle:
$AD^2 = AE^2 + DE^2$
That is:
$(12-r)^2 = 8^2 + r^2$
Expanding the brackets gives:
$r^2 - 24r+144 = r^2 + 64$
Then, cancelling terms gives:
$24r = 80$
Therefore, $r = \frac{24}{80} = 3\frac 13$, so the radius of the semicircle is $3\frac 13\text{cm}$.
