Isometric Rhombuses
Weekly Problem 31 - 2016
The diagram shows a grid of $16$ identical equilateral triangles. How many rhombuses are there made up of two adjacent small triangles?
The diagram shows a grid of $16$ identical equilateral triangles. How many rhombuses are there made up of two adjacent small triangles?
Problem
Image
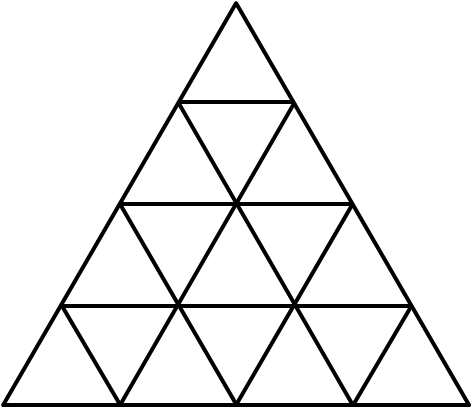
The diagram shows a grid of $16$ identical equilateral triangles.
How many rhombuses made up of two adjacent small triangles are there?
If you liked this problem, here is an NRICH task which challenges you to use similar mathematical ideas.
Student Solutions
There are a number of different ways of solving this problem.
Image

The number of vertical rhombi can be seen by looking at the possible positions of the top triangle. The following six rhombi are then apparrent.
The problem has rotational symmetry, so the other two directions will also give six rhombi each.
This means that the total number of rhombi is $3 \times 6 = 18$.
Image
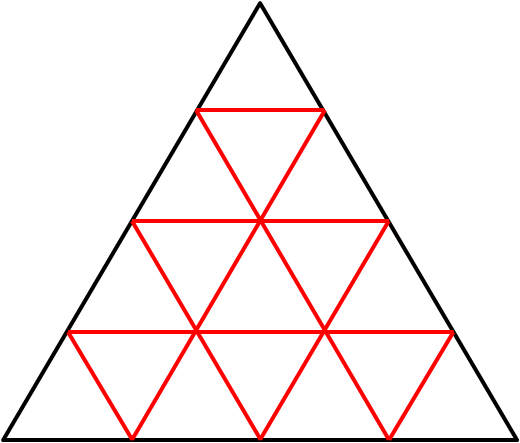
Each rhombus that is formed has exactly one of the interior edges (marked in red) contained within it. Moreover, each interior edge corresponds to one rhombus, consisting of the triangles on either side. There are $18$ interior edges, so $18$ rhombi that can be formed.
Image
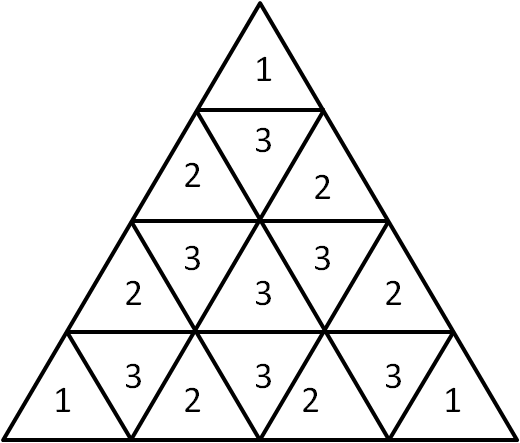
For each of the small triangles, the number of rhombi that contain it can be counted, as shown in the diagram on the right. However, this counts each rhombus twice (once for each triangle it contains). Therefore the total obtained ($36$) must be halved, giving a total of $18$ rhombi.
