Eulerian
Weekly Problem 37 - 2014
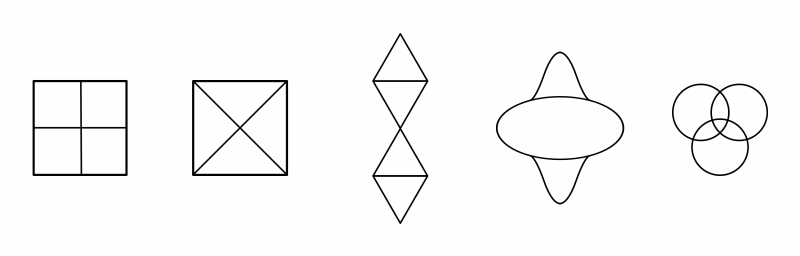
Which of the five diagrams below could be drawn without taking the pen off the page and without drawing along a line already drawn?
Which of the five diagrams below could be drawn without taking the pen off the page and without drawing along a line already drawn?
Problem
Which of the five diagrams below could be drawn without taking the pen off the page and without drawing along a line already drawn? Can you explain your reasoning?
Image
If you liked this problem, here is an NRICH task that challenges you to use similar mathematical ideas.
Student Solutions
For it to be possible to draw a figure without taking the pen off the paper and without drawing along an existing line, there must be either no points or exactly two points in the figure at which an odd number of lines meet.
To see why this must be the case, let us consider one point where lines meet and let us also suppose that we neither start nor end to draw the figure at that point. Since we are not allowed to draw along an existing line and as we 'enter' such a point from one direction, we need to leave it along a different line. So an even number of lines meet at such a point.
Image
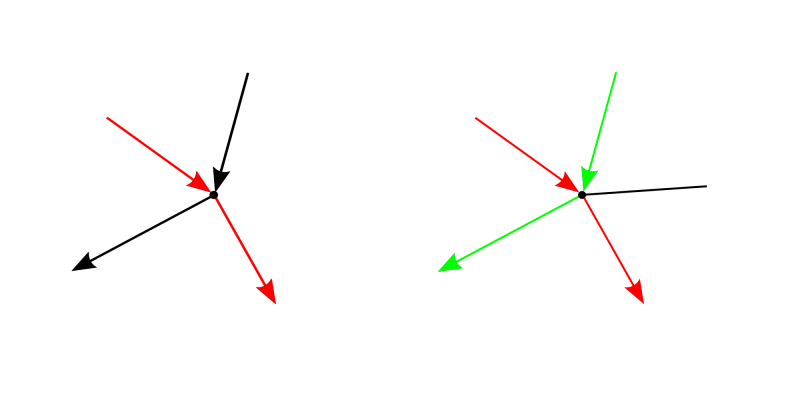
If the starting and the end point coincide then this is also a point at which an even number of lines meet. Otherwise, both the starting and the end point are points at which an odd number of lines meet as we leave (enter) this point once without entering (leaving) the point.
We can now check that only the last picture satisfies these conditions and indeed, one can draw it without taking the pen off the paper and without drawing along an existing line.
