Three sets of cubes, two surfaces

How many models can you find which obey these rules?
Age
7 to 11
Challenge level
filled star filled star filled star
Exploring and noticing Working systematically Conjecturing and generalising Visualising and representing Reasoning, convincing and proving
Being curious Being resourceful Being resilient Being collaborative

Problem

This can be used as a follow-on from Two on Five.

 
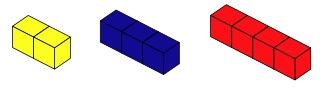
You have interlocking cubes of three different colours - 2 of one colour, 3 of another colour and 4 of the third colour.
It could look like this;
Three sets of Cubes, Two Surfaces


This is slightly different from Two on Five but is seen as an extension for some pupils. You might like to go there first!

The nine cubes are to be connected in the usual way with the following rules being applied. 

The two yellow cubes are not allowed to touch the wall or floor surfaces

The Three blue cubes must touch one surface only, the wall or the floor

The four red cubes must touch both wall and floor surfaces

 

Here are two examples that obey the rules;

 

 
Three sets of Cubes, Two Surfaces
 
See what others you can find.
How many will there be?
At some point ask yourself "I wonder what would happen if I ...?"