Let's Investigate Triangles

Vincent and Tara are making triangles with strips from the class construction set.

They have strips of three different lengths: 4 holes, 7 holes and 11 holes.

There are plenty of strips of each length. 

Vincent makes a triangle with two strips that are length 7 and one strip that is length 4, like this: 

Image

Tara makes a triangle with three strips that are each length 11, like this:

Image

 

How many different triangles can you make using strips that are length 4, length 7 and/or length 11?

You can use the interactivity below to try out your ideas if you do not have a construction set.

Can you find three strips which cannot be made into a triangle when you use them together?

Why is it impossible to make a triangle from those three strips?

Why do this problem?

This problem allows children to make important discoveries about triangles through representing the sides of triangles with strips from a construction kit. They will have the opportunity to find out which combinations of lengths cannot be made into triangles, and why this is so.

If learners have access to the physical resources, they may also discover how rigid triangles are, and that three fixed lengths can only be arranged in one way (unlike other polygons such as quadrilaterals where the angles can be changed).

Ideally, this investigation would be carried out using a construction set and therefore requires plenty of equipment and space. However, the interactivity will enable all children to access the mathematics to some extent, even without the hands-on resources. 

Possible approach

You may like to show one of the triangle images to the class and ask them what they see, and what they would like to ask. Give learners time to talk in pairs before sharing their thoughts with the whole group. Some of the observations may not be mathematical, but it is important all comments are valued at this stage.

Build on what is said to introduce the problem, encouraging children to work in pairs on making different triangles with lengths 4, 7 and 11. (They will benefit from talking through their ideas with a partner.) If you do not have access to a construction set, learners could use the interactivity in pairs on a tablet or computer. You may want to demonstrate how to change the length of the strips and how to rotate each strip first.

In a mini plenary, you could discuss how they are keeping track of the triangles they are making, and you may like to draw attention to any pairs who are recording in some way. For example, children may sketch the triangles, or record just using the length of the strips used.

You could invite pairs to record a triangle they have made on the whiteboard for everyone to see, and that way you will gradually build up a class solution (this will be made easier if the class agrees on a way of recording as a group first). Emphasise that when another solution is added to the board, it is everyone's responsibility to check it, and to make sure it is not a repeat of one already there.

In the plenary, you can look at the class solutions altogether. It might be useful to record each solution on a separate sheet of paper so they can be moved around and ordered, to help reveal any that are missing. In this way, the group can be sure that all possiblities are found. As the discussion proceeds, make a note of any combinations of three rods that don't make a triangle. To conclude the lesson, invite learners to have a go at explaining why three particular rods cannot make a triangle. They may manipulate strips a bit like this:

Image

This shows that the red and blue rods are not long enough to make a triangle with the green rod. Some children may have a 'feel' for this idea of relative length without needing to use the strips to convince themselves and others.

Key questions

Have you made any triangles using this length of strip?

Have you made any triangles with all the sides the same length?

Have you made any triangles with all the sides different lengths?

Are you sure you/we haven't made one like that already?

Why do you think you can't make a triangle with those three strips?

Possible support

Some learners might find it helpful to use just two lengths to start with. (Make sure that the longest one is shorter than two of the others put together lengthways e.g. strips which are 4 holes and 6 holes long.) You can make four different triangles with these. You can then add a third length.

 

Possible extension

Children could be encouraged to find a way to show that they have discovered all of the possible triangles using four different lengths. Some learners might like to have a go at the task Sticks and Triangles, which explores the construction of triangles further, culminating in a full generalisation.

Sorting Logic Blocks

Image

For this task, you'll need some blocks of different shapes and colours, or you could print off and cut out the shapes on this sheet.

Choose a rule, like 'only have four-sided shapes' or 'only have large shapes'.

Challenge someone else to work out your rule.

They can do this by choosing a shape for you to say either "Yes, that obeys my rule and is in my set" (you then put it over on the left) or "No, this does not obey my rule and so is not in my set" (you then put it over on the right).

How did they decide which shapes to choose?

Did they get quicker at finding out the rule?

What was the smallest number of shapes they needed to try?

Could you make some more shapes to add to the set? What would you make and why?

Tell us about some of the rules you chose and how you decided which shapes to try.

Why do this activity?

This activity can be used to build up children's confidence with the language associated with 2D shape. 

Possible approach

You can do this activity as a whole class, teacher-led activity, by creating shapes on the interactive whiteboard. Choose a particular property and drag a shape which meets that criteria to the left of the screen underneath a title of something like 'These shapes are in my set', or simply 'Yes'. (Alternatively, you could print off, laminate and cut out shapes from this sheet and stick them to the board.)

The first time you do this it's a good idea to conduct it in silence. Children take turns to come to the front and select a shape. Without saying anything, you drag it to the appropriate side of the screen. When they think they know your rule they could write it down on their whiteboards.

Once they become familiar with the game, you can interrogate them about their choices.  'Why did you choose that one?'; 'I'll put that one here - what does that tell you?'.

You could then give out sets of the shapes to pairs of children for them to try themselves (if you have 'logiblocks' these are ideal, otherwise print off shapes from the sheet). In this way, you can use the same activity to highlight different properties of shapes so it can be returned to many times over the course of an academic year.

Key questions

Why did you choose that one?

I'll put that one here - what does that tell you?

Which would be a good one to choose now? Why?

Possible support

For a class that is less confident, you could scaffold their learning by writing several different rules on the board. They then know that your rule is one of those and can choose accordingly. Children could make up their own rule for you to write.

Possible extension

Challenge children to use as few shapes as possible to test their ideas. 

Matching Triangles

These triangles belong to three different families.

 

Image

 

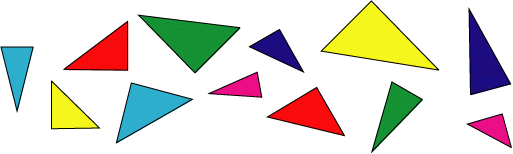
All the triangles in the same family are the same shape.

But they may not be the same size or the same way up.

 

Can you sort them out and explain how you did it?

You could print off pictures of the triangles (here as a Word document or here as a pdf), then cut them out and sort them practically. Or if you prefer, you could use the interactivity below to try out your ideas:

 

Why do this problem?

This activity is a good one to try with young children once they are familiar with the properties of a triangle. Often, they associate the name 'triangle' with a shape in a particular orientation and this problem is an excellent way to challenge this assumption. Other children may dismiss all three-sided shapes as triangles without looking at their other attributes. The activity will require pupils to look carefully at each shape and scrutinise its properties.

Possible approach

You could start by asking the group to tell you what they know about triangles. You could then ask one child to draw a triangle on the board and ask someone else to draw a different triangle. Invite the group to talk about what is the same and what is different about them. In this way, the discussion will include shape, size and orientation, but you could draw some triangles yourself to bring out certain aspects.

Next you could project the interactivity onto the interactive whiteboard, or show them the triangles on these sheets.  (The first page has the triangles in colour, the second in black and white so that it can be photocopied.) Introduce the task and encourage the group to work in pairs with a set of cut-out triangles so that they are able to talk through their ideas with a partner. Listening to their justifications can reveal a lot about their understanding of similar triangles, even though this terminology is not used.

You can draw the class together for mini plenaries, as appropriate, perhaps to share misunderstandings or ways of working. You may wish to use the interactivity for drawing attention to particular triangles (it does not allow you to rotate the triangles). Once the majority of pairs have grouped their triangles in some way, invite the class to wander around the room looking at the arrangements. What do they notice? Do they have any questions? The interactivity could be used again to re-create a solution.

Key questions

Possible extension

Children could draw their own families of triangles and label the differences and similarities.

Possible support

Use one of these sheets so that the triangles can be cut out, then rotated and placed on top of one another. (The first page has the triangles in colour, the second in black and white.)