Tessellating triangles

Can you make these equilateral triangles fit together to cover the paper without any gaps between them? Can you tessellate isosceles triangles?

Age

7 to 11

Challenge level

Exploring and noticing Working systematically Conjecturing and generalising Visualising and representing Reasoning, convincing and proving

Being curious Being resourceful Being resilient Being collaborative

Problem

Equilateral triangles have three sides the same length and three angles the same. Can you make them fit together to cover the paper without any gaps between them? This is called 'tessellating'.

What about triangles with two equal sides? These are isosceles triangles.

Can you tessellate all isosceles triangles?

Now try with right angled triangles. These have one right angle or 90 degree angle.

Some triangles have sides that are all different. Can you tessellate these?

Could you tessellate any triangle?

Teachers' Resources

Tessellating Triangles

Equilateral triangles have three sides the same length and three angles the same. Can you make them fit together to cover the paper without any gaps between them? This is called 'tessellating'.

What about triangles with two equal sides? These are isosceles triangles.

Can you tessellate all isosceles triangles?

Now try with right angled triangles. These have one right angle or 90 degree angle.

Some triangles have sides that are all different. Can you tessellate these?

Could you tessellate any triangle?

Why do this problem?

This problem encourages children to use the right vocabulary when talking about shape properties. They will begin to understand that, for a shape to tessellate, the angles where they come together are important.

Possible approach

The most useful resource for this investigation would be a large number of cut-out triangles, either paper/card or plastic. Children may also find dotty/squared paper useful.

Encourage the pupils to talk about what they are doing, perhaps with a partner, and report their findings back to the class frequently. Try to question the children in such a way so as to lead them to explore this, perhaps by drawing their attention to a particular part of the tessellation. For example, use lots of coloured tiles to build a pattern like this:

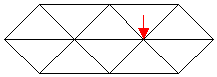
At the point marked by the arrow you could try asking questions like:

If I took out one of the triangles, how do I know which way it fits in to make the tessellation?
Why won't it fit if the triangle is rotated?

This should prompt your pupils into considering the angles within the individual shape itself, which can be extended to discussion about the sum of the angles at the point shown. This work can make a lovely display!

Possible extension

You could also challenge the children to make tessellating patterns using more than one type of shape.

Possible support

If there are children who are finding this activity difficult, give them plenty of time to manipulate the shapes practically.

Or search by topic

Number and algebra

Geometry and measure

Probability and statistics

Working mathematically

Advanced mathematics

For younger learners