Doesn't add up
In this problem we are faced with an apparently easy area problem, but it has gone horribly wrong! What happened?
Problem
Doesn't Add Up printable sheet
Here is an 8 by 8 square that has been divided into four pieces:
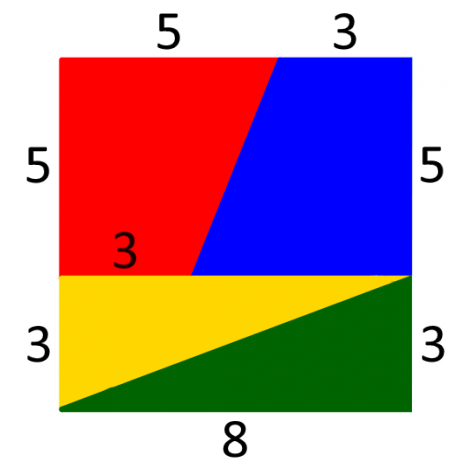
Here is a rectangle made from the same four pieces:
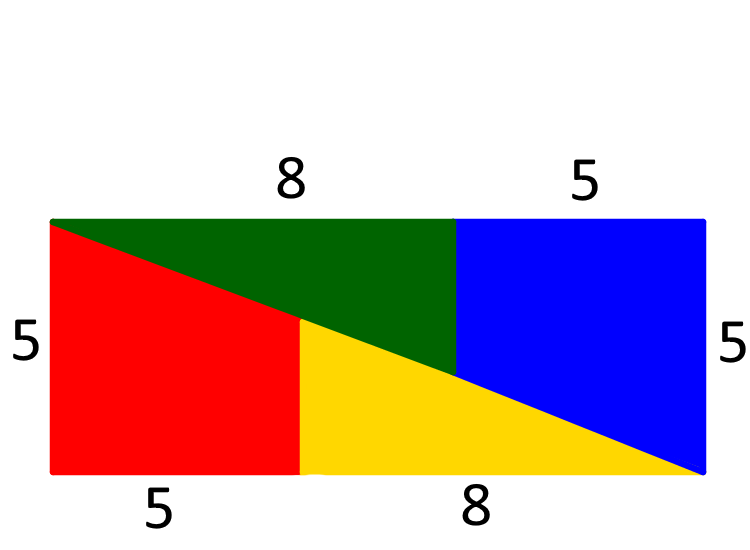
You might like to try cutting out the shapes from a piece of paper.
It seems that the same pieces can make two shapes with different areas!
Can you explain where the extra area comes from?
Here are some questions you might like to consider:
Can other squares be split up and rearranged to make rectangles with a different area?
Are there other square/rectangle pairs where the areas differ by 1 square unit?
Is there a pattern in the sizes of squares that can be arranged in this way?
Getting Started
- Cut an 8 by 8 square into the four pieces and rearrange them as shown
- What is the area of each piece ?
Student Solutions
Patrick from Woodbridge School offered the following insight to this problem, as did Chip from King's Ely School.
The difference arises because there is a difference in the line gradient between the trapezium and the triangle. The sloping line of the trapezium has a gradient of $\frac{2}{5} = 0.4$ , whereas the hypotenuse of the triangle has a gradient of $\frac{3}{8} = 0.375$.
This means that the lines made when you join the triangle and trapezium together are not straight, so there is a small amount of space, as shown on my diagram. This space adds up to the extra $1^2$cm.
An anonymous solver also wrote: The area of the original square was $64$ square units and the area of the rectangle was $65$ square units. What appears to be a diagonal of the rectangle is not in fact a straight line and the extra unit of area comes from the long thin parallelogram in the middle of the rectangle.
You can confirm this by making a careful scale drawing for yourself on graph paper. The long thin parallelogram occurs because the slopes of the trapezia are not the same as explained by Patrick and Chip: the slopes of the triangles. For each trapezium the slope is in the ratio of 2 up to 5 across and for each triangle the slope is in the ratio of 3 up to 8 across. | Image
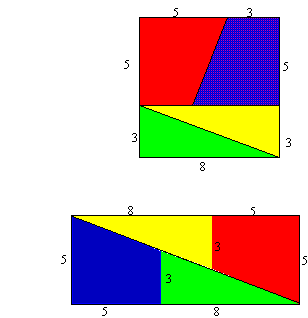
|
Finally, Susie from DEECD Victoria offered an example of other numbers that work (Do they have the same properties as the numbers used for the lengths in the problem?). She said:
Teachers' Resources
This problem is available as a printable worksheet: Doesn't Add Up.
Why do this problem
This problem forces attention on what happens when we calculate area by dissection into known shapes and the care we should take in our justification. In problem solving diagrams can often be misleading, and not just diagrams we draw for oursleves. There is a need to question and check that the representation is meaningful and conveys the message accurately. This problem brings that idea to the fore.
Possible approach
You may be interested in our collection Dotty Grids - an Opportunity for Exploration, which offers a variety of starting points that can lead to geometric insights.
Many students will need to dissect the 8 by 8 square into the four pieces to begin to get a feel for the possible source of the discrepancy. Although students want to know the answer (that's what motivates the problem-solving effort) it is the process of finding a route to an answer which provides the real long-term benefit.
Once someone says out loud where to look for the discrepancy the problem is over.
To avoid this, encourage students who are ready to develop a convincing argument to share and then to move on to the challenge of creating similar problems. When ready, move to a whole group discussion emphasising the need for convincing arguments. The extension activity also gives scope to those who see what is happening quickly.
A possible context for this problem is the so-called "infinite chocolate trick" which has appeared in a number of online videos.
Key questions
- What assumptions have been made?
- What is the area of each piece?
- How would you convince someone else?
Possible support
Explore the area calculation for a Parallelogram and then for a Trapezium by dissection.
Possible extension
Do you recognise the numbers involved in this problem? Can you account for why this problem works with those numbers?
Try the problem Lying and Cheating.
