Biren approached it like this:

The question asks you to prove that $a+b=c$.

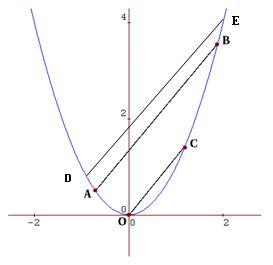
You already know that the two lines are parallel.This tells us that they have the same gradient.

To work out the gradients we should try to imagine we draw a right angled triangle under both lines.

We then divide the change in the $y$ direction by the change in the $x$ direction.

The change in the $x$ direction of the line $AB$ is equal to $b-a$ (small letters refer to the co-ordinates).

The change in the $y$ direction is equal to $$b^2 - a^2$$(these letters also refer to the co-ordinates).

Thus the gradient of line $AB$ is equal to: $$\frac {b^2 - a^2}{b - a} = \frac {(b-a)(b+a) }{ b-a} = b+a$$ The change in the $y$ direction of the line $OC$ is $c^2$ The change in the $x$ direction is equal to $c$. Thus the gradient of the line $OC$ is $$\frac {c^2}{c} = c$$

We know that both lines, $AB$ and $OC$ are parallel, and so they must have the same gradient.

So $b+a = c$

Alex extended the result as follows: Let $d$ and $e$ be the $x$ coordinates of D and E respectively. As OC is parallel to DE, the relationship of OC to DE is the same as it was to AB, therefore $c=d+e$ just as $c=a+b$. The $x$ coordinates of the midpoints of AB, DE and OC and all the same, because $$\frac{(a+b)}{2 }= \frac{(d+e)}{2} = \frac{c}{2}.$$ The straight line $x = \frac {c}{2}$ passes through the midpoints of all the parallel lines.

Others approached the problem in a slightly different way:

Let $y = mx + n$ be the equation of the line going through the points $A(a, a^2 )$ and $B(b, b^2 )$.

At $A$, $a^2 = ma + n$

At $B$, $b^2 = mb + n$

From this: $$a^2 - b^2 = ma - mb = m (a - b).$$ Dividing by $(a-b)$: $$m = \frac {a^2 - b^2}{a - b} = \frac {(a + b)(a - b)}{a - b} = a + b$$ The line $OC$ passes through the origin and the point $C(c, c^2 )$.

Because it passes through the origin, its equation is of the form: $$y = ux$$ Because it is parallel to the line passing through $A$ and $B$, $u = m$, so its equation is: $$y = (a + b)x$$ Since it passes through $C$: $$c^2 = (a + b)c$$ or: $$c = a + b.$$