Copyright © University of Cambridge. All rights reserved.
'Follow the Numbers' printed from https://nrich.maths.org/
Show menu
Why do this problem?
This activity is one which is accessible to a very wide range of children. It also opens out the possibility of pupils asking “I wonder what would happen if . . .?” Pupils' curiosity may be easily aroused while finding exploring further. It enables them to enjoy 'playing with' numbers, and discover peculiar yet interesting
things that can happen! It could be used to raise some pupils' self esteem in mathematics, whilst still giving scope for others to explore further and further showing their resilience and perseverance.
Possible approach
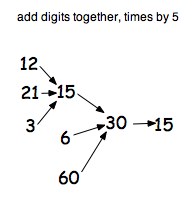
Ask pupils to choose a number then tell them the rule: add the digits and multiply by two. Follow the rule a few times so that the children are happy with it. For some pupils that act of suggesting the starting number helps them feel able to participate. Once the rule has been applied then of course you could try a new starting number.
Give children the chance to work in pairs or small groups. Introducing the need to record what you get could be approached after a short time so that "numbers are not lost"!
This activity may be suitable for 'simmering' over a period of several days or weeks. You could dedicate some wall space to it so children could post up their 'journeys' for particular starting numbers. It could then become a whole class investigation.
Key questions
Whilst they are working on their own or in small groups you can ask them to tell you anything that they notice.
Can you find attractive ways of displaying their numbers for others to see?
What would you like to call this group of numbers that you've discovered?
Have you got to an end? If so how do you know?
What could you try now that you seem to have got to the end of that journey of numbers?
Possible extension
Make sure that every number from $1$ to $99$ has been used to start the journeys, sticking to the same rule. Pupils should be able to ask themselves "I wonder what would happen if I ...?", so they can change one part of the rule.
When two or more have been completed these can then be compared and pupils can be encouraged to make predictions about future results.
Possible support
Plenty of digit cards will help children who find recording more difficult.
For the highest-attaining
When quite a few have been completed, encourage pupils to compare them carefully. What is similar, different and the same? Some might like to show the sequences using their choice of appropriate software. They could then derive hypotheses relating to the sequences and test them.
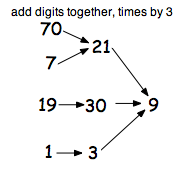 and
and