Lots of Biscuits!
Help share out the biscuits the children have made.
Problem
On Tuesdays Green Class sometimes do baking.
One Tuesday Miss King said that William's group were going to make biscuits. There were four children in the group besides William. They are Ali, Jess, Karni and Danny.
William, Jess, and Karni were going to work together to make biscuits with chocolate chips in them.
Ali and Danny were going to make ginger cookies.
When Miss King took Ali and Danny's tray of biscuits from the oven they looked like this:
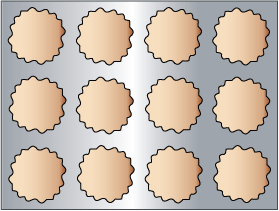
How many biscuits did they make?
"Let's share them out!" cried Danny, "There are lots of biscuits! We'll get lots each, won't we?"
If they shared them between just Ali and Danny, how many would they get each?
"What about Will, Jess, and Karni?" objected Ali, "They should get some too."
Just then Miss King took the other tray of biscuits from the oven. They looked like this:
How many biscuits did the three of them make?
"Ooh! They smell good!" exclaimed William.
"Can we eat them now?" asked Karni.
"We will have to cut some of them up, if we are going to divide them between us fairly," said Jess thoughtfully.
If they shared them between the three of them, how many would they get each?
Ali looked at both trays. He counted all the biscuits. How many were there altogether?
They decided to share all the biscuits between all five of them. How many did they get each?
"What about Miss King?" Danny asked suddenly. "She might like some biscuits too."
Can they share all the biscuits equally between the five children and Miss King?
How many more biscuits could they bake so that they could share them fairly without cutting any of them up?
Getting Started
Could you find some 'pretend' biscuits to help you with this problem?
Or could you draw a picture?
Student Solutions
Finlay from Stalyhill Junior School answered the final part of this problem, which asked how many more biscuits the children would need to bake so that they could share them equally with Miss King too. Finlay said:
They would need to make $4$ more biscuits. They would then get $4$ biscuits each as $24$ divided by $6$ is $4$.
Well done, Finlay. Emerson and Hamish from St Peter's added a bit more detail:
If you add all the biscuits up you get $20$. There are six people so you need to find the multiple of $6$ that is closest to $20$ but that is also above it. The answer is therefore $24$. $24-20=4$ Therefore they need to bake $4$ more biscuits.
Ed from St Peter's sent in answers to all parts of the problem. He said:
Ali and Danny baked $12$ biscuits. If the biscuits were shared between Ali and Danny, they would receive $6$ each.
Will, Jess and Karni baked $8$ biscuits. If the biscuits were shared between Will, Jess and Karni they would receive $2 \frac{2}{3}$ each.
There were $20$ biscuits baked all together. If they shared all the biscuits between the $5$ children , each child would receive $4$ bisuits.
Yes, they can share all the biscuits between all the children and Miss King. Each person would receive $3\frac{1}{3}$ biscuits. The children would have to bake $4$ more biscuits to make $24$ in total and therefore each person receiving $4$ biscuits each.
Thank you, Ed. It's a shame you didn't tell us how you worked out this solution.
Teachers' Resources
Lots of Biscuits!
On Tuesdays Green Class sometimes do baking.
One Tuesday Miss King said that William's group were going to make biscuits. There were four children in the group besides William. They are Ali, Jess, Karni and Danny.
William, Jess, and Karni were going to work together to make biscuits with chocolate chips in them.
Ali and Danny were going to make ginger cookies.
When Miss King took Ali and Danny's tray of biscuits from the oven they looked like this:
How many biscuits did they make?
"Let's share them out!" cried Danny, "There are lots of biscuits! We'll get lots each, won't we?"
If they shared them between just Ali and Danny, how many would they get each?
"What about Will, Jess, and Karni?" objected Ali, "They should get some too."
Just then Miss King took the other tray of biscuits from the oven. They looked like this:
How many biscuits did the three of them make?
"Ooh! They smell good!" exclaimed William.
"Can we eat them now?" asked Karni.
"We will have to cut some of them up, if we are going to divide them between us fairly," said Jess thoughtfully.
If they shared them between the three of them, how many would they get each?
Ali looked at both trays. He counted all the biscuits. How many were there altogether?
They decided to share all the biscuits between all five of them. How many did they get each?
"What about Miss King?" Danny asked suddenly. "She might like some biscuits too."
Can they share all the biscuits equally between the five children and Miss King?
How many more biscuits could they bake so that they could share them fairly without cutting any of them up?
Why do this problem?
This activity is an opportunity for young children to solve a practical problem that involves sharing into groups of two and five, and even six. By introducing the problem within the context of baking, children might surprise you with the level of mathematics they can handle. Having high expectations of learners is a key attitude and this challenge allows children to work at their own level. Some might be keen to discuss cutting up biscuits, whereas others might be happy to have some 'left over', or some for another day, for example. You may be able to bake biscuits with the children so that they have the 'real thing', alternatively you could make salt dough cookies or cookies out of plasticene/play dough. Or, you could simply introduce the context as a story and allow the children to create their own representations.
Possible approach
Key questions
Possible extension
Encourage learners to find more than one answer to the final part of the problem and then even to generalise: some children might be able to articulate that if they bake more biscuits in sixes, then they will always be able to share them out fairly.
Possible support
Some children might find useful this sheet which has the pictures of the two trays of biscuits on it.