Beam me up
Problem
The curvature, $\kappa$, of a circle of radius $r$ (given by the equation $y=\sqrt{r^2-x^2}$) is defined to be $\frac{1}{r}$. The curvature of a more general curve $y=f(x)$ varies from point to point, and equals the curvature of the circle which just matches the 'bend' of the curve at that point. It has the formula
$$\kappa = \frac{y''}{(1+y'^2)^{\frac{3}{2}}}\;.$$
The angle $\psi$ by which a point on a curve has deviated from a straight line is $\psi = \int{\kappa}ds$. i.e. the angle deviated is the sum of the curvature up to that point. Think about this formula. Does it make sense? Just think about curvature for circles to begin with.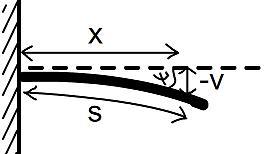
In structures, we generally expect the deflections of solid objects to be small. (Think of how little a bridge moves up and down when you walk on it, relative to its length.) So we can usually approximate the distance along a curved beam, $s$, as the linear distance, $x$, i.e. $\delta s \approx \delta x$.
Can you work out a formula for the vertical deflection (displacement) $v$ of a point on the curve, in terms of $\psi$ and $x$?
What about $v$ in terms of $\kappa$ and $x$?
Getting Started
Understanding the formula $\psi = \int \kappa ds$
Consider a beam which is a perfect arc of a circle
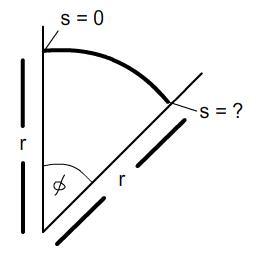
Calculate $\int \kappa ds$ along this section. Can you relate $\phi$ to $\psi$?
Finding $v$ in terms of $\kappa$ and $x$
Consider a very small section of the beam, curved length $\delta s$ and vertical deflection $- \delta v$. Can you express $\delta v$ in terms of the other quantities?
Student Solutions
Like Mach Attack, this question provides an opportunity to derive an interesting engineering formula for yourself. You might see it quite quickly, but don't be too concerned if you don't.
Understanding the formula $\psi = \int \kappa ds$
Carrying on from the hint, $\int_{s=0}^{\phi r} \kappa ds = \int_{0}^{\phi r} \frac{1}{r} ds = \phi$, the angle swept out at the centre of the circle. If we then draw a straight line from $s=0$ to $s=\phi r$, we find that $\phi = \psi$, where $\psi$ is the angle the beam has deviated from a straight line. Thus the formula is proved for circles. For a more general curve, as we zoom in around a point, we can approximate the curve better and better by a circle, until in the limit of zooming in to a single point it matches exactly.
Find $v$ in termsof $\kappa$ and $x$
Deriving the formulae in this problem is all to do with looking at small limits, i.e. considering a distance $\delta s$ along the curve which is very small. This is a common trick in engineering, and at the end we will integrate over all of these small sections to get the full result.
When $\delta s$ is small, we can approximate the situation by a triangle:
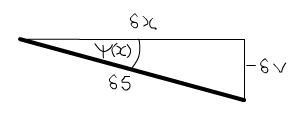
[Note about the minus sign in the above diagram: this is simply convention that vertical displacement is positive up, but curvature and angle are positive when the beam is bent down, such that the top of the beam is in tension.]
Thus $- \delta v = \delta s \sin \psi (x)$.
For small deflections, such as we are considering, $\psi$ is small so $\sin\psi \approx \psi$ and $\delta x = \delta s \cos \psi \approx \delta s$. Thus $ - \delta v \approx \delta x \psi (x)$.
To get total deflection we need to sum all these small deflections along the length of the beam, which is where integrating comes in. We find $v = - \int{\psi}dx$
And since $\psi = \int{\kappa}dx$, we find the formula $v = - \int{\int{\kappa}}dx dx$. You could alternatively write this as $\kappa = -\frac{d^2v}{dx^2}$.
You can apply this formula to a real problem in Euler's Buckling Formula.
