Links to the University of Cambridge website
 Links to the NRICH website Home page
Links to the NRICH website Home page

 Links to the NRICH website Home page
Links to the NRICH website Home page

Nurturing young mathematicians: teacher webinars
30 April (Primary), 1 May (Secondary)
30 April (Primary), 1 May (Secondary)
Or search by topic
Number and algebra
Geometry and measure
Probability and statistics
Working mathematically
For younger learners
Advanced mathematics
Weighing Scales
Age 14 to 16
ShortChallenge Level 





- Problem
- Solutions
Answer: 2
A pyramid weighs $p$, a sphere weighs $s$ and a cube weighs $c$.
 $2p + s = c$
$2p + s = c$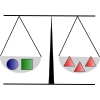 $s + c = 3p$, but $c=2p+s$ so: $$\begin{align}s+(2p+s)&=3p\\
$s + c = 3p$, but $c=2p+s$ so: $$\begin{align}s+(2p+s)&=3p\\\Rightarrow\ 2s+2p&=3p\\
\Rightarrow\ 2s&=p\end{align}$$
This problem is taken from the UKMT Mathematical Challenges.
You can find more short problems, arranged by curriculum topic, in our short problems collection.
You may also like
Two and Two
How many solutions can you find to this sum? Each of the different letters stands for a different number.
First Forward Into Logo 6: Variables and Procedures
Learn to write procedures and build them into Logo programs. Learn to use variables.
First Forward Into Logo 8: More about Variables
Write a Logo program, putting in variables, and see the effect when you change the variables.