Links to the University of Cambridge website
 Links to the NRICH website Home page
Links to the NRICH website Home page

 Links to the NRICH website Home page
Links to the NRICH website Home page

Nurturing young mathematicians: teacher webinars
30 April (Primary), 1 May (Secondary)
30 April (Primary), 1 May (Secondary)
Or search by topic
Number and algebra
Geometry and measure
Probability and statistics
Working mathematically
For younger learners
Advanced mathematics
LCM Sudoku
Age 14 to 16
Challenge Level 





- Problem
- Getting Started
- Student Solutions
- Teachers' Resources
Dan, Rohin, Sam and Sam from Grindon Hall Christian School sent in this solution:
First we worked out the letters in the left hand column which were v and c. We worked out that it could only be 7 and 4 because they were the only two numbers that were below 10 that had an LCM of 28. We saw that c=7 and v=4 because v was on a row where it had to be a multiple of 8.
Next, we worked out the middle row. We did this by using the 7 that we got earlier, and worked out that the only number that would give an LCM of 56 would be 8, so w=8. We then filled in the v, c and w squares.
Next, we worked out that a and b were 9 and 5 - because they are the only numbers from 1-9 to give an LCM of 45. We worked out that b had to be 9 because 5 does not go into 36 (6th row). So a=5 and b=9 and we filled in all the a and b squares. On the bottom row we needed an LCM of 30. We already knew a=5, so that meant that m had to be 6.
Next, we worked out that e=2 by looking at the second column. We already had the 9 and the 5 and we needed an LCM of 90 - 2 was the only number that worked in this situation from the ones we had left. In the seventh column we needed an LCM of 252 and we already had 9, 7 and 4 which have an LCM of 252, so the missing variable had to be 1, so n=1. We then filled in the e and n squares. After that the only remaining letter was d and the only number we had left was 3, so d=3. That worked because the LCM of 5,2,1 and 3 gave us 30 on the seventh row.
The resulting grid was a regular Sudoku, and we filled the numbers in using the normal rules.
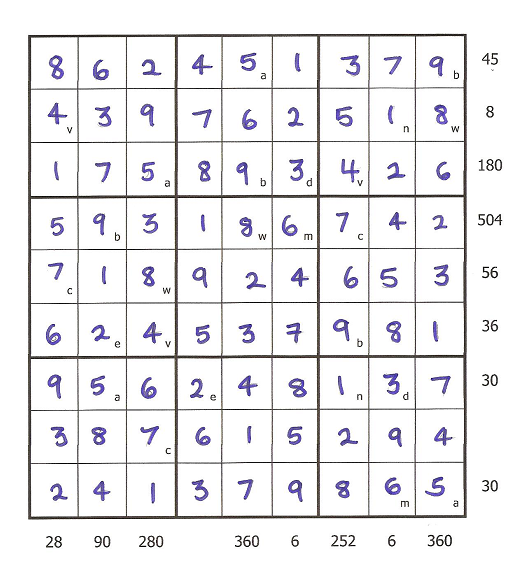
Rhea from Loughborough High School solved this problem in a different way:
First, I started by looking for two rows or columns which had at least one letter in common. I started with ones which only had 2 letters:
a and b = LCM 45 (top row)
a and m = LCM 30 (bottom row)
The only two numbers between 1 and 9 which have an LCM of 45 are 5 and 9. Likewise, the only two numbers which have an LCM of 30 are 5 and 6. As 5 is the common number and a is the common letter, I knew that:
a = 5, and I deduced from this that b = 9 and m = 6.
I filled in these numbers and looked for rows and columns which had the letters a,b or m as well as other numbers:
a,b and e = LCM 90 (second row from the left)
I can calculate that e has to be 2, because if it was 1,3 or 9- the LCM of the three numbers whould be 45. I filled in all the e's in the sudoku grid and then looked at the second row from the right:
n,d and m = LCM 6
We know that m = 6 and e = 2; the only remaining factors of 6 are 3 and 1 so-
n/d = 3/1 i.e. I didn't yet know which letter was which number.
After this, I looked at the row which had 56 as its LCM (the very middle row) and had two letters- c and w. I knew that these letters must be 7 and 8 because they are the only numbers between 1 and 9 which have an LCM of 56. The letter c also lies in the first column on the left, which has an LCM of 28. As 7 is a multiple of 28 and 56 and 8 isn't, I could conclude that:
w = 8
c = 7 From this I could also conclude that:
v = 4 (in the first column), as the LCM of 4 and 7 is 28.
After these figures were filled in, I was left with two numbers- 1 and 3, and two letters- n and d. When I looked at the second row to the top, where 4, 8 and n had an LCM of 8, I concluded that n = 1 and d = 3 because 8 is not a multiple of 3 but is a multiple of 1.
I know that I got all the numbers right because I was able to fill in the sudoku.
And here is a link to that sudoku.