Three spinners
These red, yellow and blue spinners were each spun 45 times in
total. Can you work out which numbers are on each spinner?
Problem
This is a number spinner. When you spin it, it can land on any number from one to ten.
Image
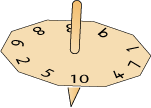
Here are three more number spinners with ten numbers on them. But you cannot see what the numbers are.
Image
I spun the red one $15$ times and got these numbers:
Image

Image

Image

Then I did the same things again twice over. I got these numbers, but forgot to note which numbers came from which spinner:
Image
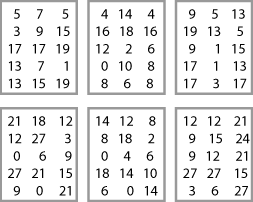
Can you work out which spinner generated each list? How did you do it?
We could call the numbers on the first spinner "Numbers from one to ten".
Can you think of a title for the set of numbers on the red spinner?
Can you think of titles for the sets of numbers on the blue and the yellow spinners?
Getting Started
What do the numbers on each card have in common with each other?
Can you see another card or cards with some of the same numbers?
Student Solutions
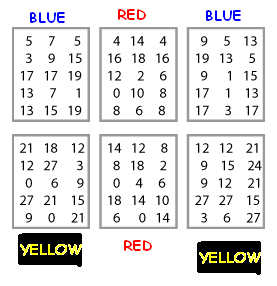
Andrew from Quarry Bay School sent us an image to show which numbers had come from each spinner:
Image
Jake from Seaford Primary described how he tackled this problem:
First I wrote down all the numbers from the red list starting with the smallest. All the numbers were in the $2$ times table so I wrote the list again with the missing numbers in.Then I did the same with the blue and yellow list.
I looked at the other six lists. Some had only $2$ times tables so I said they were from the red spinner. Some had only $3$ times tables so I said they were from the yellow spinner. Some had only odd numbers so I said they were from the blue spinner.
Ellie did a similar thing:
First of all I wrote down all the numbers you knew were on the red, yellow and blue spinners, as in the tables.I then checked these over with the tables with no matching colours and saw which ones fitted each.
I filled in the missing numbers which were not in the first three tables and came up with $10$ numbers for each colour and titles as well.
RED - even numbers from $0$ to $18$
BLUE - odd numbers from $1$ to $19$
YELLOW - multiples of three from $0$ up to $27$
Well done also to Jonathan from New Ford Primary, children from Stourport Primary and pupils from Dr Challenor's Grammar School who all sent very clear explanations of the ways they solved this problem.
Teachers' Resources
Why do this problem?
This problem challenges children to find a relationship between sets of numbers and introduces them to the idea of random events. It provides a reason to be able to scan a list which is something that some learners find quite difficult.
Possible approach
This problem could follow a series of lessons on number properties, or it could be used prior to some number work to assess your children's knowledge. In order to have a go at it, they will not need to have encountered probability before.
The activity might be best introduced orally, with just the lists of numbers displayed on the board. It would also be useful for pairs to have a copy of the resource sheet which contains all nine lists. Try not to say too much about what they might do to solve the problem -
leave them to develop their own method in their pairs. Warn them that you will expect them to explain what they did.
In a plenary discussion, focus on how the children came to their conclusions. Challenge them to explain why each spin of, for example, the yellow spinner, didn't produce exactly the same numbers.
Key questions
What can you tell me about these numbers?
What do these numbers have in common?
Which other card has these numbers?
Possible extension
Some learners can be asked to make spinners of their choice and write their own problem. Is there anything they can do to make the challenge as hard as possible? This would be a good discussion point afterwards.
Possible support
Using the resource sheet , some children might find it useful to cut out the lists of numbers and physically put the similar ones together.
