Train Routes
This train line has two tracks which cross at different points. Can
you find all the routes that end at Cheston?
Age
5 to 7
Challenge level
Problem
Here we have a train line that has different routes from Appleby to each of the main stations of Barkton, Cheston and Dorby, because there are two crossing-over lines passing through Barkton and Cheston.
The smaller stations at Yanbridge, Zogborough, Worsby, Xanly, Underton and Vilham just have a single track running through them:
Image
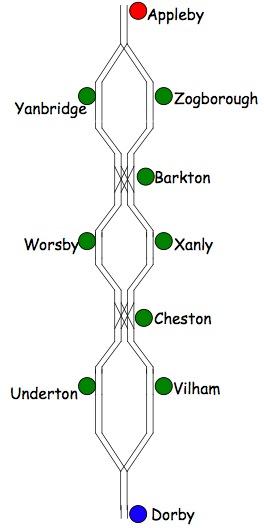
What are the different routes that
you can have to end at Cheston?
What are the different routes that
you can have to end at Dorby?
You might like to then invent your own routes that may go further
than this one and then answer similar questions that you can think
up. Getting Started
You might like to just use the initial letters for the stations.
Remember that at Barkton and Cheston you can either go straight through on the same line or cross over to the other line.
For example, you can be coming from Zogborough through Barkton to Xanly or you can be coming from Zogborough through Barkton to Worsby.
How will you know you've got all the routes?
Will you need to record the different routes in some way?
Student Solutions
We had a well presented solution from Sarah. She showed it as follows:
Two routes to Cheston:
Appleby - Zogborough - Barkton - Xanly - Cheston
Appleby - Yanbridge - Barkton - Worsby - Cheston
Two routes to Dorby:
Appleby - Zogborough - Barkton - Xanly - Cheston - Vilham - Dorby
Appleby - Yanbridge - Barkton - Worsby - Cheston - Underton - Dorby
This next solution came from Year 5 Maths Group in Parklands Primary School. They wrote:
We worked out the first answer as being 4 routes to Cheston by following the tracks which means that there is one crossover at Barkton.
The routes are
AZBXC,
AYBWC,
AYBXC
and AZBWC.
The different routes to Dorby: We already knew that there were 4 routes to Cheston and because there are two single tracks VD and UD we added these routes to the 4 existing routes to Cheston, making 8.
THEREFORE EVERY 2 SINGLE LINE STATIONS DOUBLES THE AMOUNT OF EXISTING ROUTES. EVERY CROSSOVER STATION MULTIPLIES THE AMOUNT OF ROUTES BY 4.
Andrew, Jason, James, Ibrahim, Verity and Rianna.
What fantastic reasoning! Thank you.
There were also solutions from Thomas, Leahye and Rowan, and a team solution sent in by pupils from Patcham Junior School. Well done to you all.
Teachers' Resources
Train Routes
Here we have a train line that has different routes from Appleby to each of the main stations of Barkton, Cheston and Dorby, because there are two crossing-over lines passing through Barkton and Cheston.
The smaller stations at Yanbridge, Zogborough, Worsby, Xanly, Underton and Vilham just have a single track running through them:
Image
What are the different routes that you can have to end at Cheston?
What are the different routes that you can have to end at Dorby?
You might like to then invent your own routes that may go further than this one and then answer similar questions that you can think up.One of the main reasons for this challenge is to find ways of approaching such a problem. When introducing it to the class, it would be good to put the problem into a context, perhaps by talking first about local journeys. You could ask the children if they have ever seen maps of train/bus/tram/underground routes drawn in stations or on the walls of the vehicles themselves. It would be good to discuss how the maps are "models" for the routes taken - they are simply a way to put the routes on paper to make it easy to understand.
You could then show your pupils the route in the problem and ask a few questions about it so that you are sure they understand the way it is drawn. For example, if I came from Appleby, what station would the train stop at after Barkton? (This could be Worsby or Xanly.) You might like to begin the first part of the problem as a whole class, asking them how they will know when they have all the different routes. How will they record the routes that they find? This is a good opportunity to model having a system. Your class might suggest several routes from Appleby to Cheston, and you could pair them up so that the two which both visit Yanbridge, for example, are together. In this way, even if this time they have not found them systematically in the first place, the children can see how they can develop a system next time.
Children might like to try the investigation Simple Train Journeys after having a go at this problem, which uses similar ideas but in a more open context.