Semicircle in a semicircle
The diagram shows two semicircular arcs... What is the diameter of the shaded region?
Age
14 to 16
Challenge level
Problem
The diagram shows two semicircular arcs, PQRS and QOR . The diameters, PS and QR , of the two semicircles are parallel; PS is of length 4 and is a tangent to semicircular arc QOR .
What is the area of the shaded region?
Image
If you liked this problem, here is an NRICH task which challenges you to use similar mathematical ideas.
Student Solutions
Let T be the centre of the semicircle with diameter QR and let OT produced meet the circumference of the larger semicircle at U .
Image
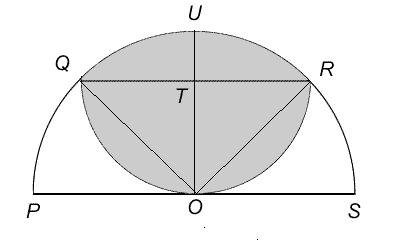
By symmetry, we note that OT is perpendicular to QR . As TR = TO = TQ (radii of the same semicircle), triangles ORT and OQT are both isosceles, right-angled triangles. So QOR is a right angle.
By Pythagoras' Theorem: QR ² = OQ ² + OR ² = 2 ² + 2 ² = 8. So $QR = \sqrt8$ = $2\sqrt2$ and the radius of semicircle QOR is $\sqrt2$.
The area of the shaded region is equal to the area of semicircle QOR plus the area of the quadrant bounded by OQ , OR and arc QUR less the triangle OQR .
So the required area is $\frac12 \pi (\sqrt2)^2$ + $\frac14 \pi 2^2$ - ($\frac12 \times 2 \times 2$) = $\pi + \pi - 2$ = $2\pi$ - 2.