 Links to the NRICH website Home page
Links to the NRICH website Home page

30 April (Primary), 1 May (Secondary)
Or search by topic
Number and algebra
Geometry and measure
Probability and statistics
Working mathematically
For younger learners
Advanced mathematics
Summing Consecutive Numbers



Summing Consecutive Numbers printable worksheet
Watch the video below to see how numbers can be expressed as sums of consecutive numbers.
Investigate the questions posed in the video and any other questions you come up with.
Can you draw any conclusions?
Can you support your conclusions with convincing arguments or proofs?
If you are unable to view the video, click below to reveal an alternative version of the problem.
Charlie has been thinking about sums of consecutive numbers. Here is part of his working out:
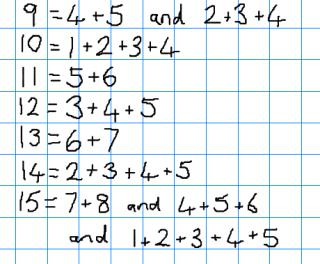
Alison looked over Charlie's shoulder:
"I wonder if we could write every number as the sum of consecutive numbers?"
"Some numbers can be written in more than one way! I wonder which ones?"
"$9$, $12$ and $15$ can all be written using three consecutive numbers. I wonder if all multiples of $3$ can be written in this way?"
"Maybe you could write the multiples of $4$ if you used four consecutive numbers..."
Choose some of the questions above, or pose some questions of your own, and try to answer them.
Can you support your conclusions with convincing arguments or proofs?
You may also like
Consecutive Numbers
An investigation involving adding and subtracting sets of consecutive numbers. Lots to find out, lots to explore.