You may also like

problem icon

Pebbles

Place four pebbles on the sand in the form of a square. Keep adding as few pebbles as necessary to double the area. How many extra pebbles are added each time?

problem icon

Great Squares

Investigate how this pattern of squares continues. You could measure lengths, areas and angles.

problem icon

Tri-split

A point P is selected anywhere inside an equilateral triangle. What can you say about the sum of the perpendicular distances from P to the sides of the triangle? Can you prove your conjecture?

Weekly Problem 3 - 2007

Stage: 3 and 4 Challenge Level: Challenge Level:1

1:1

Paper - solution


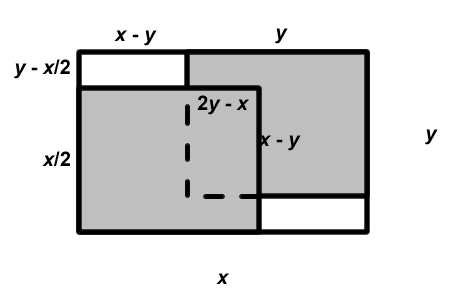
Let the sheet of paper have length $x$ and width $y$. Then the uncovered area consists of two congruent rectangles of length $x-y$ and width $y-\frac{1}{2}x$. So the uncovered area is $2(x-y)(y-\frac{1}{2}x)$, that is, $(x-y)(2y-x)$.

The area covered twice is a rectangle of length $y-(x-y)$, that is, $2y-x$, and width $\frac{1}{2}x-(y-\frac{1}{2}x)$, that is, $(x-y)$. So the area covered twice is also $(x-y)(2y-x)$.

This problem is taken from the UKMT Mathematical Challenges.

View the previous week's solution
View the current weekly problem
The NRICH Project aims to enrich the mathematical experiences of all learners. To support this aim, members of the NRICH team work in a wide range of capacities, including providing professional development for teachers wishing to embed rich mathematical tasks into everyday classroom practice. More information on many of our other activities can be found here.
Copyright © 1997 - 2012. University of Cambridge. All rights reserved.
NRICH is part of the family of activities in the Millennium Mathematics Project.