Dividing the field
Problem
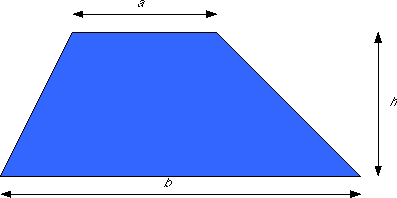
A farmer has a field which is the shape of a trapezium as illustrated below.
To increase his profits he wishes to grow two different crops.
To do this he would like to divide the field into two trapeziums, each of equal area.
How could he do this?
Student Solutions
A trapezium has area equal to half the sum of the lengths of the parallel edges times the perpendicular distance between them. The original field has area $h(a+b)/2$ and we are looking for a field with area $h(a+b)/4$.
One solution would be to join the midpoints of the parallel edges with a straight line.There are lots of other solutions. The farmer might want to start from a gate on the edge of length $a$ , at a distance $d$ from one corner, and $(a-d)$ from the other. The dividing line would have to be drawn to a point on the opposite side of the field at a distance $(a+b)/2$ from the corner.
Some people tried to solve a harder problem, that is to split the field by a line parallel to the parallel edges of the field. A particular solution to this was sent in by Jack , Henry, Paul and Matthew from Smithdon High School. It is shown below.
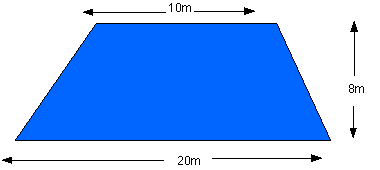

For the first field:
$60=y \times (10-x )/2$- (1)
and for the second field:
$60 = (8-y) \times(20+x)/2$ - (2)
Equation (1) can be rearranged to give:
y = 120 / (10+ x ) - (3)
and equation (2) gives:
120 = (8 - y ) (20 + x ) - (4)
Combining equations (3) and (4) gives:
$120 = (8-120/[10+x])(20+x)$ - (5)
so
$120(10+x)=(8[10+x]-120)(20+x)$
and
$1200+120x=(80+8x-120)(20+x)$
which leads to
$150+15x=(x-5)(x+20)$
and $150+15x=x^2+15x-100)$
so $x^2 = 250$m$^2$
which gives $x=15.8$m (3 s.f.)
and in equation (3) $y=120/(10+x)$ so $y= 4.65$m (3 s.f.)
A further challenge: The solution $x^2 = (a^2 + b^2)/2$ is very special mathematically because it is the same whatever the value of the distance $h$. The language mathematicians use is to say that "the solution is independent of $h$''. Can you explain why this happens in this particular problem? If you want a hint then you could use the fact that when you enlarge a shape with a linear scale factor $s$ then the area is enlarged by a scale factor $s^2$. Look out for more about this problem in a future article on areas and scaling.
Correct solutions to the problem were received from Nicholas - South Greenhoe Middle School ;Heacham Middle School; Lucy and Sarah - Archbishop Sancroft High School
