Once upon a time
Problem
Once upon a time there were three bears. There was Father Bear, Mother Bear and Baby Bear. But you knew that!

Mother Bear was three quarters the size of Father Bear.
Baby Bear was half the size of Father Bear.
And all the things they had were in the same proportions.
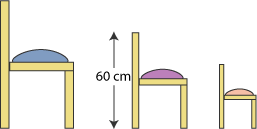
This means that Father Bear's chair was twice as big as Baby Bear's chair and Mother Bear's chair came exactly in between.


Getting Started
If Mother Bear's chair is three quarters of the height of Father Bear's, what is one quarter of the height of Father Bear's chair?
So can you work out how tall Father Bear's chair is?
How can you work out the diameter of Baby Bear's bowl from the diameter of Father Bear's?
Why don't you try measuring the height of the beds?
Student Solutions
Congratulations to all of you who explored the House of the Three Bears, and sent in some nicely reasoned answers. This problem involved using ratios, proportions, and fractions, as well as using and understanding appropriate mathematical language. We received lots of great solutions (including some from the USA, Canada, and also Turkey!), so thank you very much for this.
Marcus of Ardingly College answers the first part of the question about the chairs:
Father Bear had an $80$ cm chair, so three quarters of $80$ cm is $60$ cm and that is the height of Mummy Bear's chair.
Half of $80$ cm is $40$ cm and this is Baby Bear's height of his chair.
Kathryn, also of Ardingly College also nicely explained her answer about the chairs:
If Mother Bear's chair is $60$ cm and it is $\frac{3}{4}$ the size of Father Bear's chair then Father Bear's chair must be $80$ cm because $80\div4 =20$ $20\times3=60$.
Baby Bear is half the size of Father Bear so his chair must be $40$ cm because $\frac{1}{2}$ of $80 =40$.
Many other people submitted correct solutions to this part. These include: Chris and Sam from Ardingly College, Ivor from Eynsham Community Primary School, Philippa from Portsmouth Grammar Junior School, Jessica from Southgate School, Tutku, Berk, and Okyanusfrom FMV Isik Erenkoy ILKOGRETIM (Turkey), Gleb from Bourne Community College, Toby from Droxford Junior School, Charlotte, Niamh, and James from Eskdale School, Sarah and Megan from Tudhoe Grange, and Mrs. Darling's Class from Paton School Shrewsbury MA (USA). Mrs. Darling's Class used the "guess and check" method to work out the right answer.
For the next part of the problem, you were asked to find the diameter of Mother Bear's bowl, and the height of Baby Bear's bowl. Unfortunately, whilst many people submitted solutions with the correct working, they did not read the question! The correct solution should give an answer for the diameter of Mother Bear's Bowl, and the height of Baby Bear's bowl; some submitted the height of Mother Bear's Bowl instead, or the diameter of Baby Bear's bowl.
Philippa of Portsmouth Grammar Junior School gives a nice explanation for the bowls:
$\frac{3}{4}$ of $24$ cm = $18$ cm (Mother's bowl across)
$\frac{1}{2}$ of $24$ cm = $12$ cm ( Baby Bear's bowl across)
$3$ into $12$ cm = $4$ cm (Baby Bear's bowl height)
Others who submitted correct soultions included: Chris and Sam from Ardingly College, Ivor from Eynsham Community Primary School, Jessica from Southgate School, students from Lomond School, Gleb from Bourne Community College, James and Andrew from Eskdale School, Lina and Ellie from the Whitby Maths Club, Claire from St. John's French Immersion School (in Ontario, Canada), and Sam from The Harrodian School.
Kathryn goes on to give a nicely explained answer for the rest of the question:
The blue bed has to be Father Bear's bed if my theory will work.
The red bed has to be Baby Bear's bed because it is half the size of Father Bear's bed.
Mother Bear's bed is the yellow one because it is $\frac{3}{4}$ the size of Father Bear's bed.
The only bed left is green so this must be Goldilocks'.
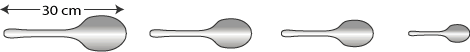
Father Bear's spoon is $30$ cm and $\frac{1}{6}$ of $30$ is $5$.
$5 \times 5=25$ so Goldilocks' spoon must be $25$ cm.
Baby Bear's spoon must be $15$ cm ($\frac{1}{2}$ of $30 = 15$)
So if Goldilocks' spoon is $25$ cm and Baby Bear's spoon is $15$ cm then it must be $\frac{3}{5}$ the size of Goldilocks' spoon.
Ellie, from Portsmouth Grammar School expressed the lengths of the spoons as a fraction of Daddy Bear's:
daddy's= $\frac{6}{6}$, goldilocks= $\frac{5}{6}$, mother's= $\frac{4}{6}$, babys= $\frac{3}{6}$ therefore baby's spoon is $\frac{3}{5}$ of goldilocks' spoon
Well done also to the following people, who submitted the right answer to both the bed and spoon questions: Ivor, Harshil from H.A.B.S., the students from the Lomond School, Sam from The Harrodian School and Maya from Green Dragon Primary School.
Well, as you can see the Bear family all have their own things, in their own size, which is just right for each of them. Goldilocks too has her own spoon and bed, which is just the right size. We now know whch object belongs to whom, and their dimensions!
If you enjoyed this problem (I know that you did- I certainly did!), have a go at these similar problems: Orange Drink, and Timber!. If you would like more practice, try Cherry Buns.
Teachers' Resources
Why do this problem?
Possible approach
Key questions
Possible extension
Learners could try these similar problems - Orange Drink or Timber!.
Possible support
Suggest starting by drawing the bears' chairs on squared paper and counting squares or trying this shorter, and therefore less daunting, problem - Cherry Buns.
