Tea cups
Place the 16 different combinations of cup/saucer in this 4 by 4 arrangement so that no row or column contains more than one cup or saucer of the same colour.
Problem

Aunt Jane had been to a jumble sale and bought a whole lot of cups and saucers - she's having many visitors these days and felt that she needed some more. You are staying with her and when she arrives home you help her to unpack the cups and saucers.
There are four sets: a set of white, a set of red, a set of blue and a set of green. In each set there are four cups and four saucers. So there are sixteen cups and sixteen saucers altogether.
Just for the fun of it, you decide to mix them around a bit so that there are sixteen different-looking cup/saucer combinations laid out on the table in a very long line.
So, for example:
a) there is a red cup on a green saucer but not another the same, although there is a green cup on a red saucer;
b) there is a red cup on a red saucer but that's the only one like it.
There are these sixteen different cup/saucer combinations on the table and you think about arranging them in a big square. Because there are sixteen, you realise that there are going to be four rows with four in each row (or if you like, four rows and four columns).

1) In any row there must only be one cup of each colour;
2) In any row there must only be one saucer of each colour;
3) In any column there must only be one cup of each colour;
4) In any column there must be only one saucer of each colour.
Remember that these sixteen cup/saucers are all different so, for example, you CANNOT have a red cup on a green saucer somewhere and another red cup on a green saucer somewhere else.
There are a lot of different ways of approaching this challenge.
When you think you have completed it, check it through very carefully. It's even a good idea to get a friend who has seen the rules to check it also.
This challenge is also found at nrich.maths.org.uk/7397
Printable Roadshow.pdf resource
Getting Started
Have you checked that all your cup and saucer combinations are different?
What could you try on the diagonals?
Is there any pattern to the way you have arranged the cups and saucers so far? Could you continue the pattern?
Student Solutions
We had a few good solutions to this challenge. Firstly from The Blue Coat Primary School in Wotton-Under-Edge
First I would put all the combinations together, then I'd line up all the double colour combinations together (red, red, white, white, green, green, blue, blue). After that I put all of the red tops diagonally and the blue tops. I found it easier by spacing my cubes out, in my diagonal rows the saucer couldn't be the same as my double saucer, so I had to look really carefully according to the rules. Finally I filled the rest in by always having only one of every colour in each row and column.
My tip is to look at the problem in a different way :).
Next, from Eliza at St. Joseph's School in Australia
To start with I drew a 4 x 4 grid. In the grid I placed 4 saucers of each colour so that It matched the criteria. When they were done I placed the tea cups on top so that there was only one green on red and only one red on green and so on. Then I had four tea cups left and I places them on their own colour. Once I had placed the cups on their correct saucers I went through and check and double checked. This is how I did tea cups.

Then, from Grace from Birdwood Primary in Australia
I used some counters and a grid that was four squares long and four squares wide. I placed counters for the saucers first and realised that I couldn't have the same colour counter on the diagonal. So I placed different colour counters on the diagonals. Then I worked out where the remaining counters had to go. All of the counters for the saucers were placed and checked to see that there were no counters the same colour in each row and column. I then placed the red cups on one diagonal and the white cups on the other diagonal. I then placed the blue and green cups. I then checked my work.
The picture seems to indicate that the coloured counters were replaced to give an interesting picture!

Next from Andrew from Dulwich College in Singapore
Explanation:
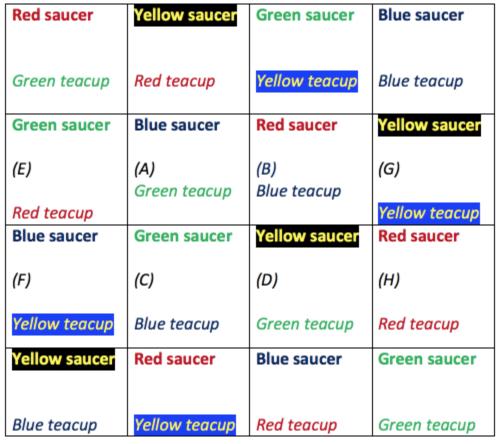
You would want to create the 2 main diagonals (the 4 square ones)matched up with the same teacup or saucer. Then once that's done, find the lower diagonal square of Position A (which is the upper diagonal square is position F) and put in the same saucer in F as you did in A. Do the same thing for Position B and H. They can be random teacups for those 4 positions, but not for C,E,D and G. Match the same saucer for the upper diagonal square for Position C (which the upper diagonal square is position E), but MAKE SURE THAT THE TEACUPS MATCH. Do exactly the same for positions D and G. Then, do the remaining squares on the outside matching the saucers but at the same time making sure that the teacups match, as this is crucial. Once you have done the outside, this should be done and if they are still mistakes, take out all the mistaken squares out and re-arrange them with diagonals so they match, although if you did everything carefully and accurately, there should be no need for this bonus step. By the time you do all of this, you should have a beautiful, satisfying square of 16 saucers and teacups. Lovely, isn't it?
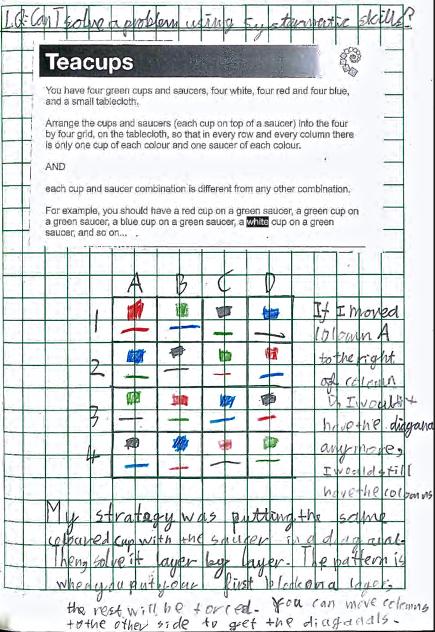
Next, here's Dylan's work sent in by the teacher from Dulwich College in Beijing China
Here is a solution from Dylan at an international school in Beijing. We spent two lessons exploring patterns and moved onto looking at 3 layers (including a plate). Dylan managed to discover the patterns with rotating opposite cups and saucers to reach the combinations. He also ensured that the diagonal line had the same coloured cup and saucer as this was his
first strategy to solving the problem.
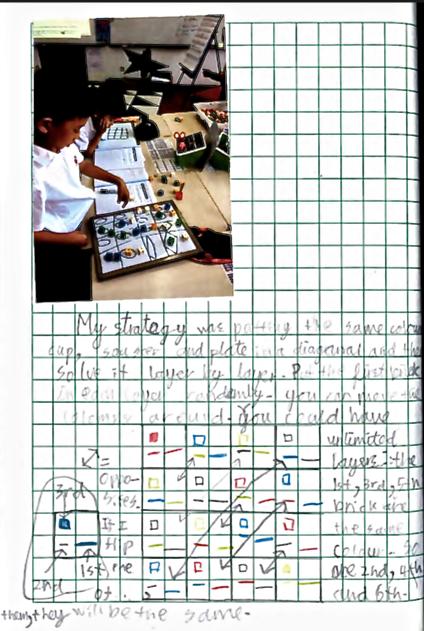
Finally Sam from Raymond Park Middle School in the USA

Teachers' Resources
Why do this problem?
Possible approach
See also this article by a PGCE student about using this activity.
If you need to check solutions you might like to use this interactive.
(When you have a complete solution it will say Well Done!!)
Key questions
Possible extension
For more extension work
You can expect these pupils to look carefully at different solutions (as opposed to different ways of solving the challenge) and then compare them to sort out similarities and differences as well as equivalences. Then the problem can be extended to include a third attribute, for example a plate, so that the cup, saucer, plate combination would use three different colours. Deciding how to record solutions in this case is quite a challenge.
Is it possible to arrange the cups and saucers if the diagonals also have to be different? Is there a system for getting all the possible answers?
Possible support
For youngsters who have difficulties with colours you might want to use this image:

(A bigger version can be seen here.)
Children have done this activity using a variety of different materials to help them - it can be made part of the challenge for them to decide on the materials they will use. You could start with just three differently coloured cups/saucers to be arranged in a 3 by 3 grid so that the aim of the investigation is understood. (The Teddy Town problem is essentially the same investigation as this one, but starts at a simpler point.)
Extra







