Fiene, who is homeschooled in The Netherlands, sent in the following picture:



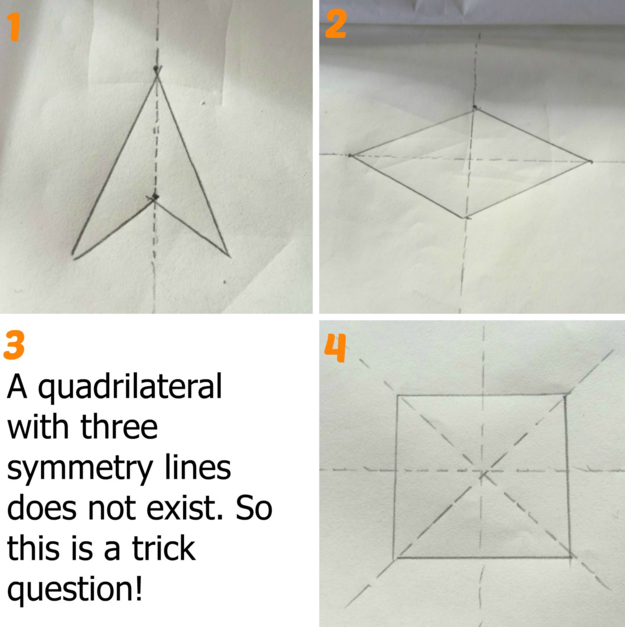
This is what Fiene said:

1. quadrilateral 1 symmetry:
Imagine this shape is a cookie. It only has 1 symmetry line because there is only 1 way to break it in 2 so that you get 2 equal halves.

I wonder whether you know the mathematical name for your first shape, Fiene?

2. quadrilateral 2 symmetry:
You have a rhombus, there are two ways to break it so that you have equal halves.

3. quadrilateral 3 symmetry:
This is a trick question! I don't think a quadrilateral with three symmetry lines exists.

4. quadrilateral 4 symmetry:
You have a square, there are four ways to break it in 2 so that you have equal halves.

 
Will, Hugh, Emil and Ben from Oare Primary School wrote:

1] Firstly, we got a ball of string and rolled it out so we could each hold a corner and we cut it.

2] Next, we thought up a shape with four corners [quadrilateral]. We thought of two, a trapezium and a kite. To persuade people, you draw a kite, and tell people to draw all the lines of symmetry and there should be one.

3] A shape with only two lines of symmetry is an oblong. To prove it only has two lines, you repeat the same method as last time but with two lines of symmetry.

4] It is not possible to have a quadrilateral with three lines of symmetry, because you can't divide 4 by 3.

5] A square is a shape with four lines of symmetry.

Robert, Anezka, Billy, Liam and Zoe from Coldean Primary experimented with lots of different quadrilaterals. They found some shapes with one line of symmetry, some with two and some with no symmetry at all. They also found that there are no quadrilaterals with three lines of symmetry, and that only squares have four lines of symmetry. You can see their very well explained results here. You will also see from that document they tested a hypothesis about the relationship between symmetry and pairs of equal edges.

In answer to how you could prove to someone watching that you have identified all the lines of symmetry, they suggested making the shapes out of paper and folding them in half so that the edges and vertices match up. You could also prove it with a mirror. If you get the person to place a mirror halfway across your shape and gradually rotate it, they will see that the only positions where the reflection allows you to 'see' the whole of the original shape are the lines of symmetry that you have identified.

Thank you for sending in these solutions, they were interesting to read.