 Links to the NRICH website Home page
Links to the NRICH website Home page

30 April (Primary), 1 May (Secondary)
Or search by topic
Number and algebra
Geometry and measure
Probability and statistics
Working mathematically
For younger learners
Advanced mathematics
Cuisenaire Counting



- Problem
- Getting Started
- Student Solutions
- Teachers' Resources
We have had a few good solutions come in for this task.
Mahad from Beechview Academy sent in the following:
First, you need to look at the yellow rod. A yellow rod makes FIVE white squares so that is one way. Now, look at the red rod. 1 red rod = 2 white rods, so you could make 2 reds and 1 white, as well as the inverse (1 white and 2 red). Then you could do others based on what you just worked out (eg. red, white, red). You could also do 3 white and 1 red and make combinations from that.
Now, look at the dark green rod. 1 dark green rod = 6 WHITE rods. So you could do 3 reds next to each other. You could also do 2 white and 2 red, etc.

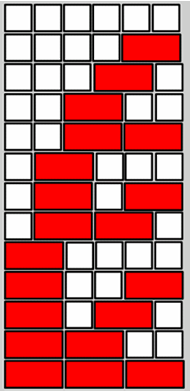
Laura sent in her solution as pictures copied from the interactivity, which is very helpful - thank you, Laura.
Here is her solution to the first challenge and the 13 different ways to make the green rod using reds and whites:

Mohammed raza Khunt from Mahatma Gandhi International School in India wrote:
My solution is showing that every even number requires some number of red rod and odd rods require some number of white AND red rods to make an odd rod. The conclusion is that odd requires red and white rod and even requires only some red rod. I have made some combinations for it in the picture.
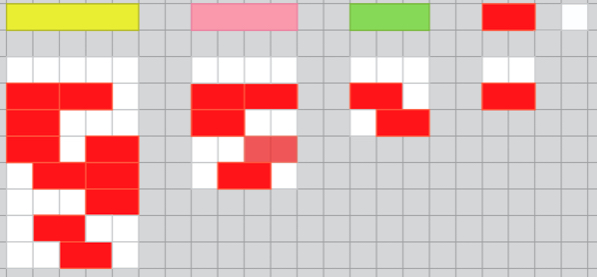
I think I understand what you've been thinking, however the explanation probably needed to say something about how the even numbers can be made from only red rods while odd numbers will require a white.
Thank you for these, maybe others seeing these may also have a go at this task.