These solutions came from Fred from Albion Heights Junior Middle School and Low Zhen Lin from Garden International School. Both contributed to this solution. From Low Zhen Lin's diagram you will see that he had the clever idea of cutting one of the faces into four pieces.

To maximize the size of the net, we transform it so that as many of the vertices of the net as possible touch the edge of the paper. This is to maximise the length of the sides and therefore, the volume.

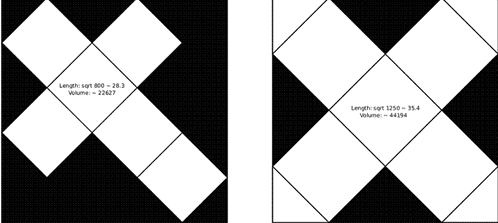
For the traditional net, the most efficient way is to have the lines parallel to the diagonals of the square, as shown in the diagram on the left.

To find the length $E$ of an edge of the cube divide the paper into 5 by 5 and use Pythagoras' theorem. So $E^2 = 20^2 +20^2$ and $E=\sqrt{800}$ or approximately 28.3, and the volume is 22627. Six of the eight non-retroflex vertices touch the edge of the square, and the net fills 48 per cent of the square.

However, this is not the most efficient net. The traditional net has a 3 $\times$ 4 bounding rectangle - the smallest rectangle that it will fit in. The most efficient net will have a bounding rectangle that is a square. That net is shown in the diagram on the right which fills 75 per cent of the square.

The edge of this cube is $\sqrt{1250}$, or approximately 35.4, and the volume is 44194.